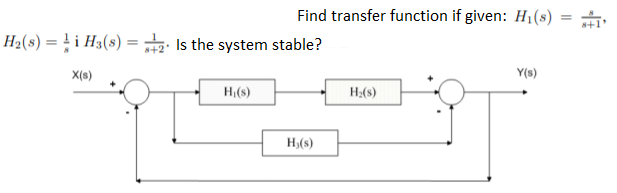
After solving I got $ \frac{s^{2}+3s+3}{(s+1)(s+2)} \\ for\, \, system\, \, stability\, \, I\, \, got \, \, s1=-1 \\ s2=-2 $$$T(s) = \frac{s^{2}+3s+3}{(s+1)(s+2)}$$
Soand for the stability of the system I got: $$ s_1 = -1 $$
$$ s_2 = -2 $$
So, is the system stable?