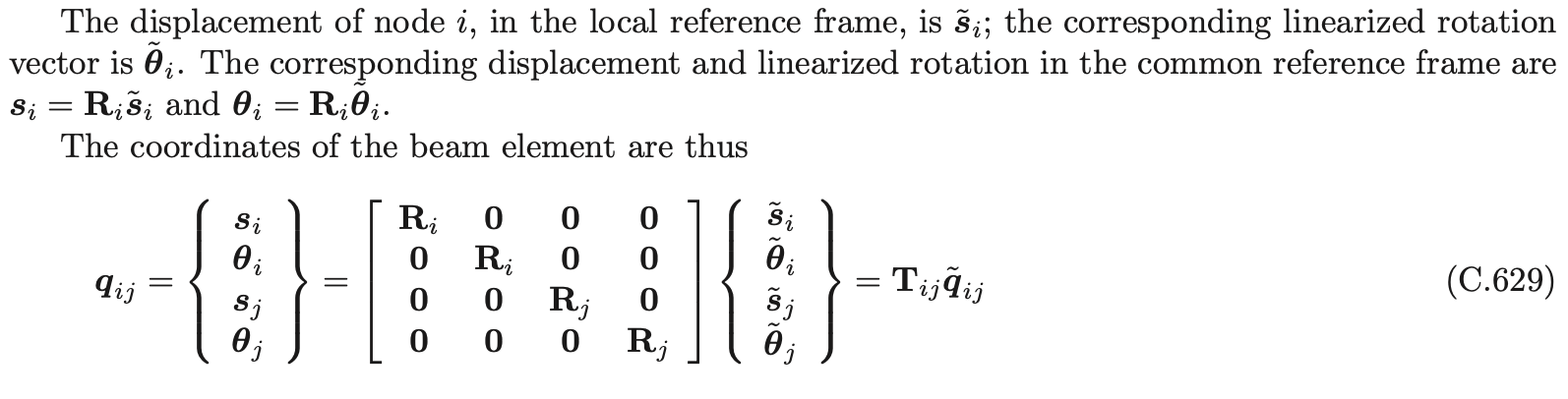
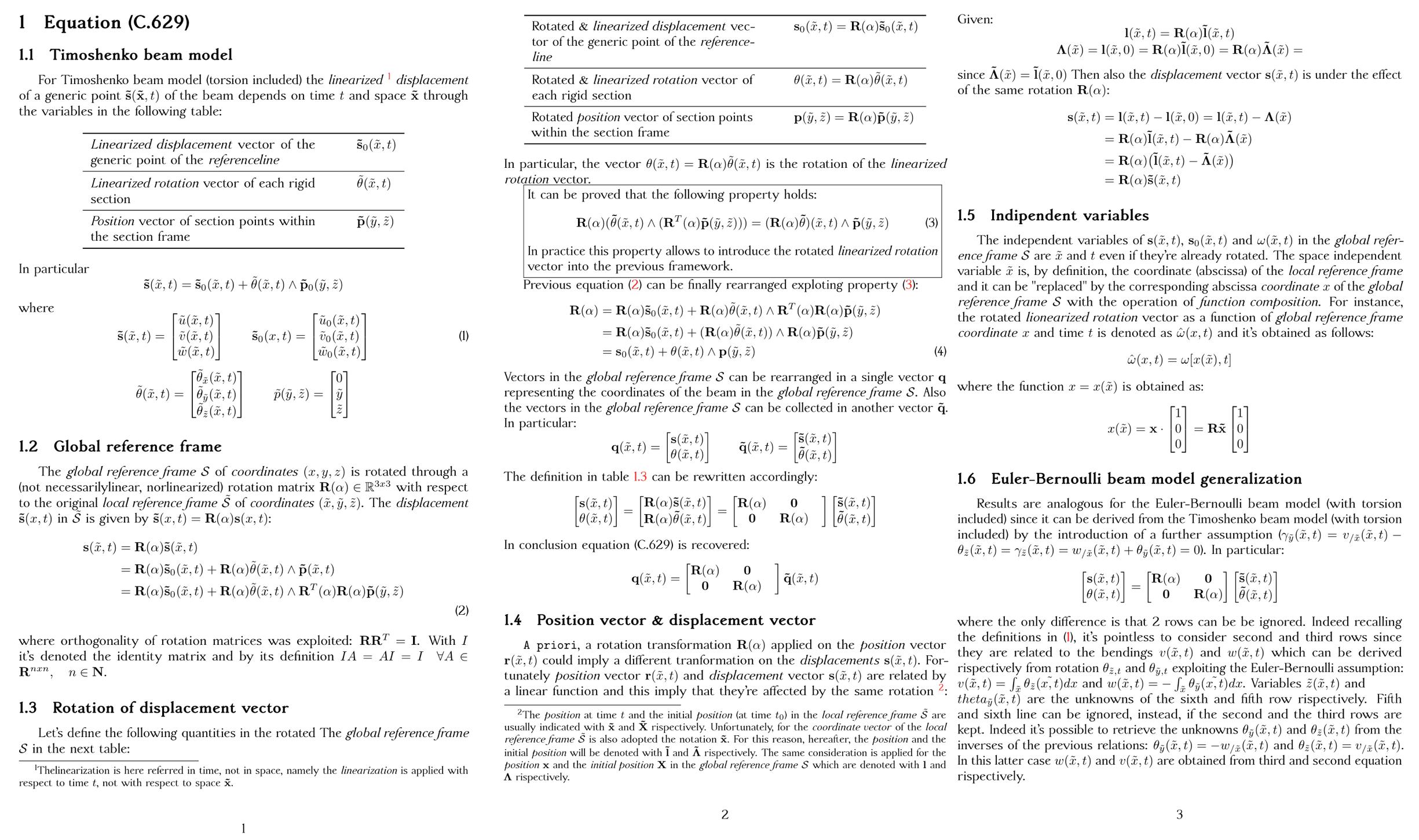
$s=R\tilde{s}$ is fine, but why $\theta=R\tilde{\theta}$? Namely: why vectors of linearised rotations $\theta_i$ and $\theta_j$ (at nodes $I$ and $j$ respectively) are rotated like they were vector displacements $s_i$ and $s_j$? For instance, rotating a frame in 2D by an angle $\alpha$, the initial rotation $\tilde{\theta}$ of a vector displacement $\tilde{s}$ becomes a final rotation $\theta$ in the new frame such that $\theta=\tilde{\theta}+\alpha$ and not $\theta=R\tilde{\theta}$. I don't think linearisation of angle $\theta$ is the reason.
1 Answer
$\begingroup$
$\endgroup$