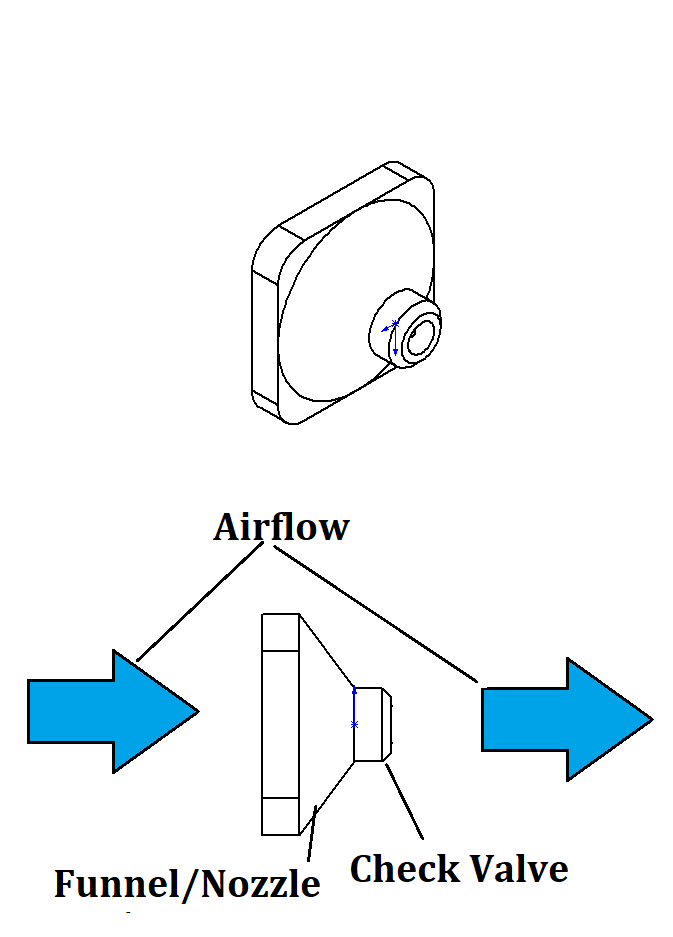
So, I was thinking about trying to build some kind of body cooling suit and started looking into the wonderful world of refrigeration systems. I've managed to get myself side-tracked pretty badly because I can't figure out why I'm right/wrong about this situation. It's a pretty simple concept.
A fan blows air into a funnel, the air speeds up out of the funnel, as the air speeds up it loses pressure, because it loses pressure, it loses temperature, I thought perhaps that flow would be obstructed by air rushing in from the other side but this can be fixed rather easily with a check valve. I didn't think it would be much but when you have a pretty extreme funnel the results get pretty dramatic, presuming my calculations are correct, which is usually a long shot. I just don't understand if or where I'm going wrong, although I'm pretty sure I'm going wrong.
I'm aware in refrigeration systems they use a different type of expansion device this is just for my own understanding.
Calculations are below, I just used an online calculator:
Gravity = 9.81 m/s
Fluid Density = 1.27 kg/m^3
Position 1
Pressure = 101,325 pascals
Height = 1 m
Speed = 15 m/s
Pipe Diameter = 0.1m
Temperature = 293.15 Kelvin
Position 2
Pressure = 12,171 pascals
Height = 1 m
Speed = 375 m/s
Pipe Diameter = 0.02m
Temperature = 293.15/8.325 = 35.21 Kelvin
Pressure change of 89,154 pascals
The Temperature out of the funnel is -237.94 Celsius! (This seems insane to me)
So to get the temperature at position 2 because temperature was not included on the online calculator, I just figured the pressure had been divided by 8.325 so I did the same to the temperature. Don't know if that's right. If anyone could enlighten me I'd be really appreciative!
-David.