1
$\begingroup$

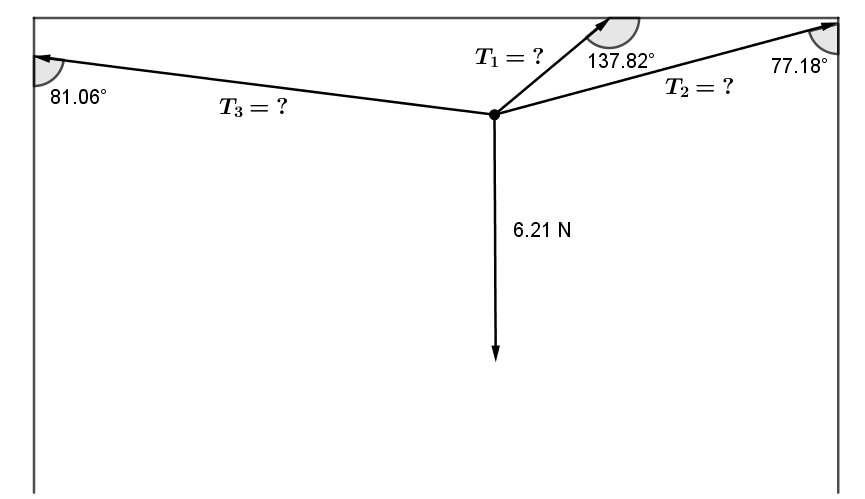
This problem is related to my previous question on the generalized Lami's theorem. I would like to see how you solve this problem and compare with my solution. My motivation for this problem is that I have not seen A SINGLE problem of this type on the internet that considers a 4-force system in static equilibrium. All the problems that I have seen consider 3 forces and those that consider 4 never ask for three unknowns, but offer more information in a way that can be solved by vector components. How do you solve this problem using vector components? I apologize for the ugly problem.

enter image description here

Note: The cable for T2 only hangs from the vertical line, NOT the horizontal.

Improve this question
$\endgroup$
2
  • $\begingroup$ My answer was incorrect, as there IS no unique/exact solution. It can be easily proved using the graphic method. $\endgroup$
    – r13
    Commented Jul 17, 2022 at 3:30
  • $\begingroup$ What are the possible solutions according to you? $\endgroup$
    – Emmanuel José García
    Commented Jul 17, 2022 at 3:44

1 Answer 1

Reset to default
1
$\begingroup$

The graphic diagram below shows there are infinite solutions (non-unique) to this problem.

Steps:

  1. Draw the gravity load to scale and mark the ends "a" and "b".

  2. Draw a construction line parallel to the vector $T_1$ from point "a".

  3. Draw a construction line parallel to the vector $T_3$ from point "b".

  4. Now make a line parallel to the vector $T_2$, but, what is the unique line length required to close the vector loop???

enter image description here

Let's try another sequence to draw the vector loop.

  1. Draw a line (3-3) parallel to $T_3$.

  2. Draw a line (1-1) parallel to $T_1$ and let it intercept the line 1-1.

  3. Set the scaled vector 6.21 on line 3-3 at 2 locations, and call the upper points "a" and "b" respectively.

  4. Draw two lines parallel to $T_2$ and let the lines pass the points "a" and "b", now we get two sets of solutions, which can be more.

enter image description here

Improve this answer
$\endgroup$
9
  • 1
    $\begingroup$ What are the tensions in the three cables according to your approach? $\endgroup$
    – Emmanuel José García
    Commented Jul 16, 2022 at 19:40
  • $\begingroup$ I've pointed out 3 of the equations required to solve the 3 unknowns. You shall be able to write the equations and solve them. The only thing you need to pay attention to is assigning the +/- sign consistently with the sign convention of your choice. $\endgroup$
    – r13
    Commented Jul 16, 2022 at 20:11
  • 1
    $\begingroup$ I think your diagram is wrong because you are assuming that the head of the vector of T2 hangs from the intersection formed by the horizontal line and the vertical line. But the vector head of T2 just hangs from the vertical line. It doesn't touch the ceiling. Sorry for the bad drawing. $\endgroup$
    – Emmanuel José García
    Commented Jul 16, 2022 at 22:02
  • $\begingroup$ In other words, the heads of the vectors of T1 and T2 do not form a horizontal line. $\endgroup$
    – Emmanuel José García
    Commented Jul 16, 2022 at 22:09
  • 1
    $\begingroup$ I suggest posting the question on the mathematics forum, from which you might get a satisfactory answer. This is a very interesting/challenging exercise though. $\endgroup$
    – r13
    Commented Jul 17, 2022 at 18:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.