Calculate the required torque of a motor

Question

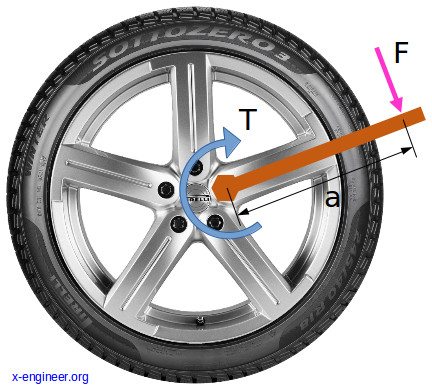
I want to know how much torque I need for my engine.

Imagine a wheel with a motor in the center. One like this:

Let's say the wheel measures 400mm, weighs 10KG, what is the formula to make that calculation?

I think the formula is (correct me if I'm wrong): 𝑇=𝐹⋅𝑎

Diameter: 400mm = 40cm = 0.4m
Force: 10kg = 100N

𝑇=𝐹⋅𝑎
𝑇=100⋅0.4
𝑇=40Nm

That is, I need a motor with a torque of at least 40 Nm to work (in theory, in practice it would be about 50 Nm, to say something), right?

Source: https://x-engineer.org/power-vs-torque/

I ask because I am not an expert, do you see any errors?

Answer 1

See here for the actual calculation: How can I calculate the power and torque required for the motor on a wheeled robot/vehicle?

But that doesn't go into the principles and from your question I think that will be required to clear up how things work:

1. $F=ma$ is linear but has a rotational equivalent which is $\tau=I\alpha$ where $I$ is the moment of inertia, $\tau$ is torque, and $\alpha$ is angular acceleration (radians per second). Mass and weight don't mix with torque.
2. Moment of inertia is the rotational equivalent of mass and is obtained from mass and geometry. For a wheel it would be a cylinder or circle. You would look for the equation for your shape in a table of moment of inertia equations to figure out what you need for simple shapes
3. The force applied at the edge of the wheel and radius/length of the lever arm determines torque. The force applied at the edge of the wheel is not weight. You can convert between torque and force if you have a radius/lever length: $\tau=Fr$
4. I assume you are not interested in spooling up the wheel in free space but rather moving the car. So you would not use wheel mass or moment of inertia of the wheel. You would use force at the wheel edge required to accelerate the mass of the car up to speed plus the force required to overcome the rolling friction, incline against gravity, air resistance which are required to maintain speed once the car is up to speed.
5. Using the equation from #1 only calculates how much torque is required to spool up the wheel as if it were a flywheel in space, off the ground.
6. As far as spooling up the wheel goes when moving the far, you would only need to account for this effect if was a significant fraction of the torque required to accelerate the car which it is not so you can neglect it.
7. With no losses like friction or gravity pulling down on an incline, accelerating a car up to speed is similar to spooling up a flywheel in that you only apply force to accelerate it up to speed. After that it remains in motion at that speed if you remove the accelerating force as per Isaac Newton. Real life has losses like friction so you need to apply extra force/torque on top of that to overcome those losses. But once they are overcome, it is as if friction iand gravity are gone since they are being cancelled so any extra force on top of that goes into acceleration as if it were frictionless or flying in space. Once the car or flywheel is up to speed you can remove that extra force/torque for acceleration and as long as you keep the force/torque to overcome losses like friction, speed is maintained. That's why freeway driving is easier on the engine than start and stop traffic.
8. Notice that apart from overcoming losses to maintain speed (whether that speed be zero or non-zero), acceleration plays into everything else so you need to specify what acceleration you want.
9. If the engine were applying just enough force to maintain speed but your speed was zero it would be like your car sitting still on a frictionless ice surface. If you nudged it in the correct direction, all that force is extra so it would accelerate to a speed based on the nudging force and then continue to move at that speed since the engine was overcoming friction.