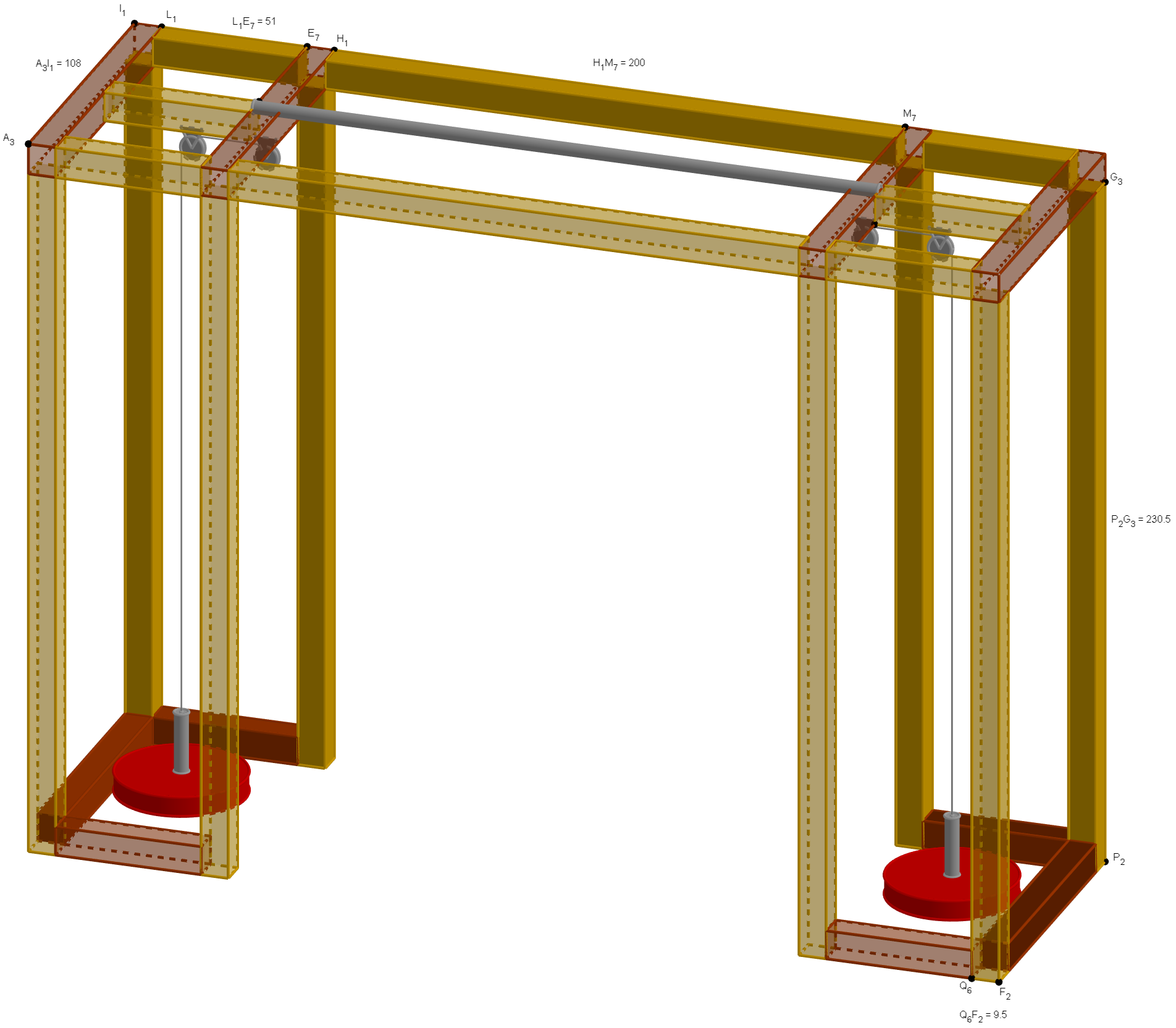
I will add my answer to the others although the results will not be much different.
The way I see it the following parameters are the most important:
- The worst case scenario for the bending of the beam:
Instead of the open handle (which is a four point bending), I would go for the close handle (which is three point bending). This is a worst loading scenario for a given force.
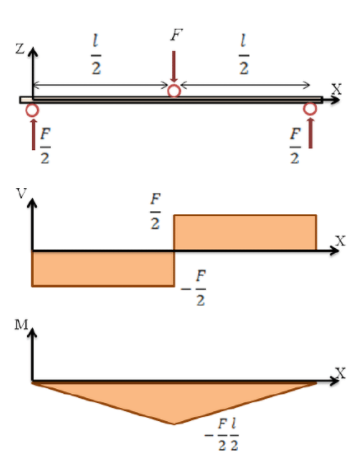
Figure 1: Three point bending load case with shear forces and bending moments
The idea behind the calculation in the question you are referring is what would happen if someone pulled the chin up and then let go only to hang. IMHO, this is still a valid scenario in your case. Because the intended use is only for strength, that does not preclude an impact load (the equivalent in a car design would be to design cars only for transporting and for crashworthiness). Therefore, I would still go for the 5.5kN (which is BTW approximately 500 kg).
- Use yield stress instead of uts:
Now this is the hard part, since you probably will be able to get easier steels rod with better strength properties rather than steel pipes. The value would be depended on the surface/heat treatments that the rod has been subjected to, so you need to have an idea. In any case you can take a small value and compare.
comparison of 42.4mm diameter pipe and 28 m steel rod.
In order to compare those to, the most basic comparison is check for their second moments of area. Since you don't know the thickness I will assume its t. Then:
| pipe |
rod 28 |
| pipe 42.4mm with t |
rod 28[mm] |
| $$\frac{\pi}{64}(d_p^4 - (d_p-2t)^4)$$ |
$$\frac{\pi}{64}d_r^4 $$ |
| $$I_p=\frac{\pi}{64}(42.4^4 - (42.4-2t)^4)$$ |
$$I_r = \frac{\pi}{64}28^4 $$ |
The structure capable of enduring more bending is the one with the greater I. Therefore, pipe would be better than rod if
$$ I_p>I_r$$
$$\frac{\pi}{64}(42.4^4 - (42.4-2t)^4) > \frac{\pi}{64}28^4 $$
After some calculations we obtain that the pipe will be stronger if the thickness is greater that $ t>1.15 [mm]$. (Of course, if you use so small a thickness, then the pipe would probably crumble due to localised bending). So in terms of geometrical resistance, the pipe will probably behave better although I would require it to have a thickness of at least 8[mm] (which I doubt you will have.
Additionally, as I mentioned earlier, it is probable that you will easier find a rod with better material properties.
Calculation for simply supported
Because, I believe the 28[mm] rod is going to be easier on the grip, I will proceed with this calculation and provide the minimum yield stress for the beam.
I assume that the maximum force of F=5.5[kN] is correctly calculated and I will use that as maximum load.
Then the maximum bending for the 3 point bending will be equal for a simply supported beam would be equal to:
$$M=\frac{Fl}{4}= \frac{5.5 [kNm]}{4}= 1.125[kNm]$$
Then maximum stress would be equal to:
$$\sigma = \frac{M}{I_r}\frac{d_r}{2}=\frac{M}{\frac{\pi}{64}d_r^4}\frac{d_r}{2}=\frac{32 M}{\pi d_r^3}$$
$$\sigma = \frac{1125[Nm]}{2.248\cdot 10^{-6} [m^3]}$$
$$\sigma = \frac{1125[N]}{2.248\cdot 10^{-6} [m^2]}$$
$$\sigma = 522 [MPa]$$
This is quite a high strength for yield (although you probably will be able to get a material with those values).
improvement with fixed ends
The above calculation is for simply supported beam. However, if you welded the additional 190mm of the beam to the structure, you would have a fixed beam with a concentrated load. In that case the loading condition would improve. I.e.
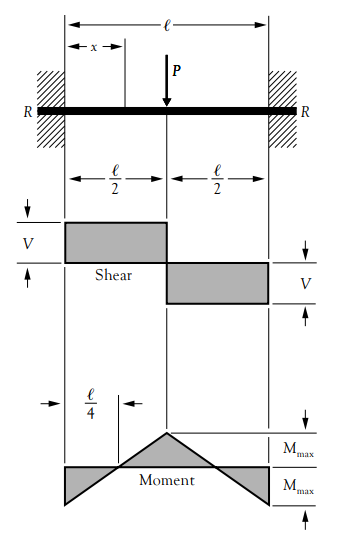
Figure 2: Beam fixed at both ends (source: awc
In that case the maximum bending moment would be $M_f= \frac{F l}{8} = \frac{M}{2}$. So the bending moment would be only half of the simply supported case.. As such the minimum yield stress would need to be greater or equal to:
$$\sigma_y \ge 261 [MPa]$$
(CAVEATS: there are some asterisks here, regarding with the rigidity of the rest of the structure and stress concentrations, however in general the welded version should perform better). This -hopefully- also answers your final question regarding constraining only horizontally.