1
$\begingroup$

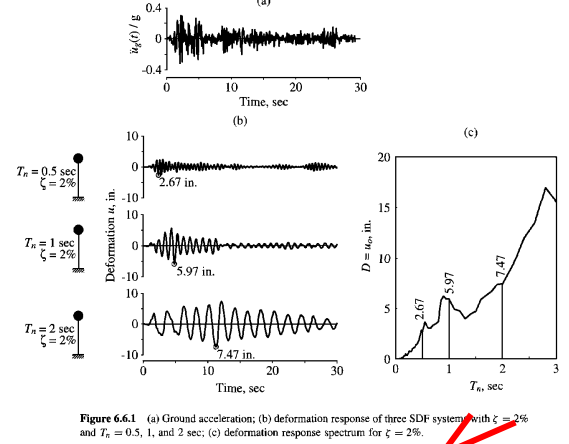
I'm reading a book of Anil. K. CHOPRA related to Structural Dynamics, I'm intending to obtain the deformation response spectrum from given ground motion. What I have here in hand is ug(t) ground motion, but I couldn't derive the deformation response from that. In book section 6.4 Response History mentions that" For a given ground motion ug(t), the deformation response u(t) of and SDF system depends only on the natural vibration period of the system and its damping ratio"

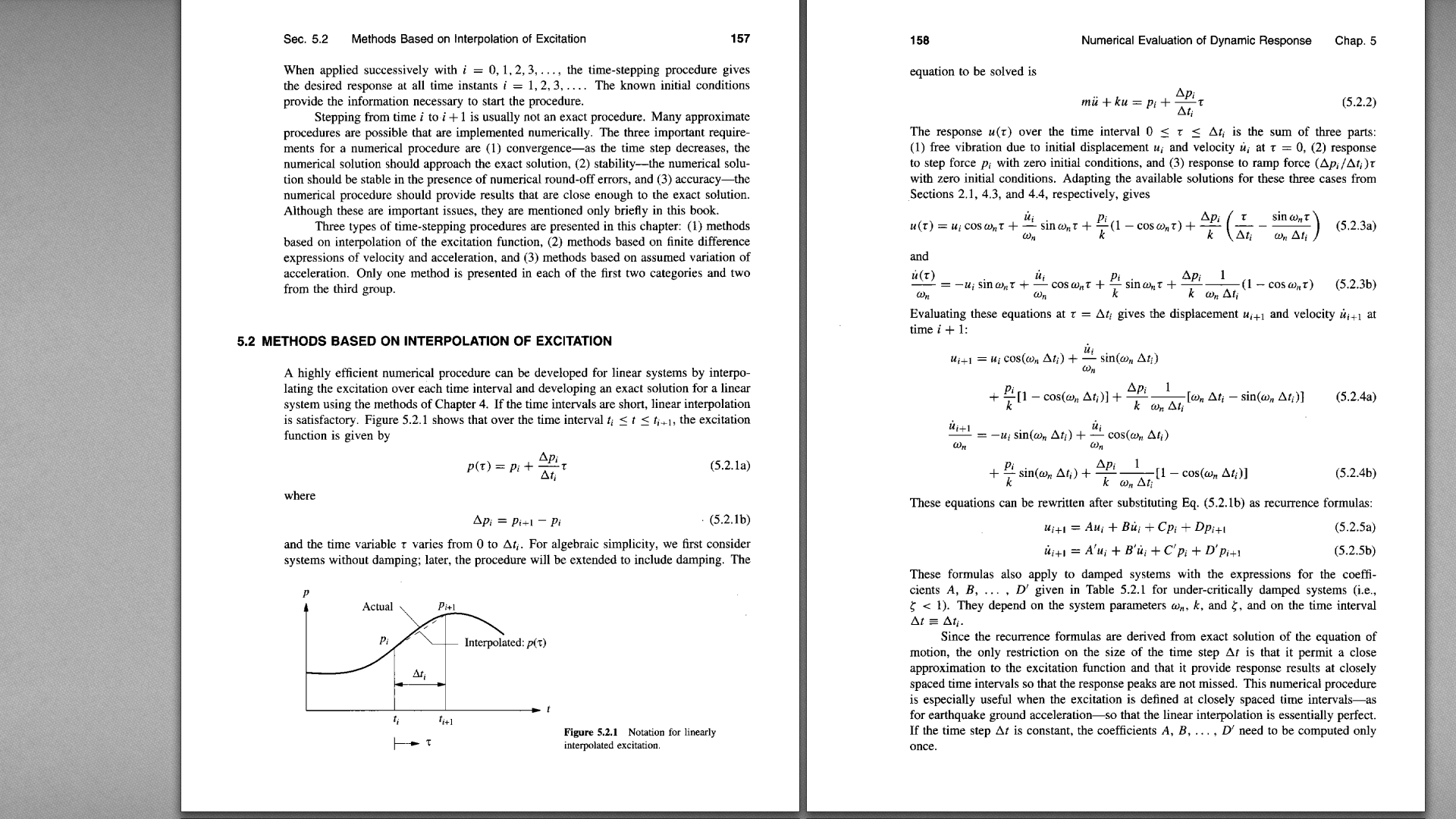
enter image description here

In the very same book there are sections which describes the Direct Integration methods such as "Methods Based On Interpolation of Excitation" to find the deformation response but in the formulation it includes the k spring coefficient of SDF system, that contradicts with previous statement in the book that deformation response depends on natural period and damping ratio.

Methods Based On Interpolation of Excitation

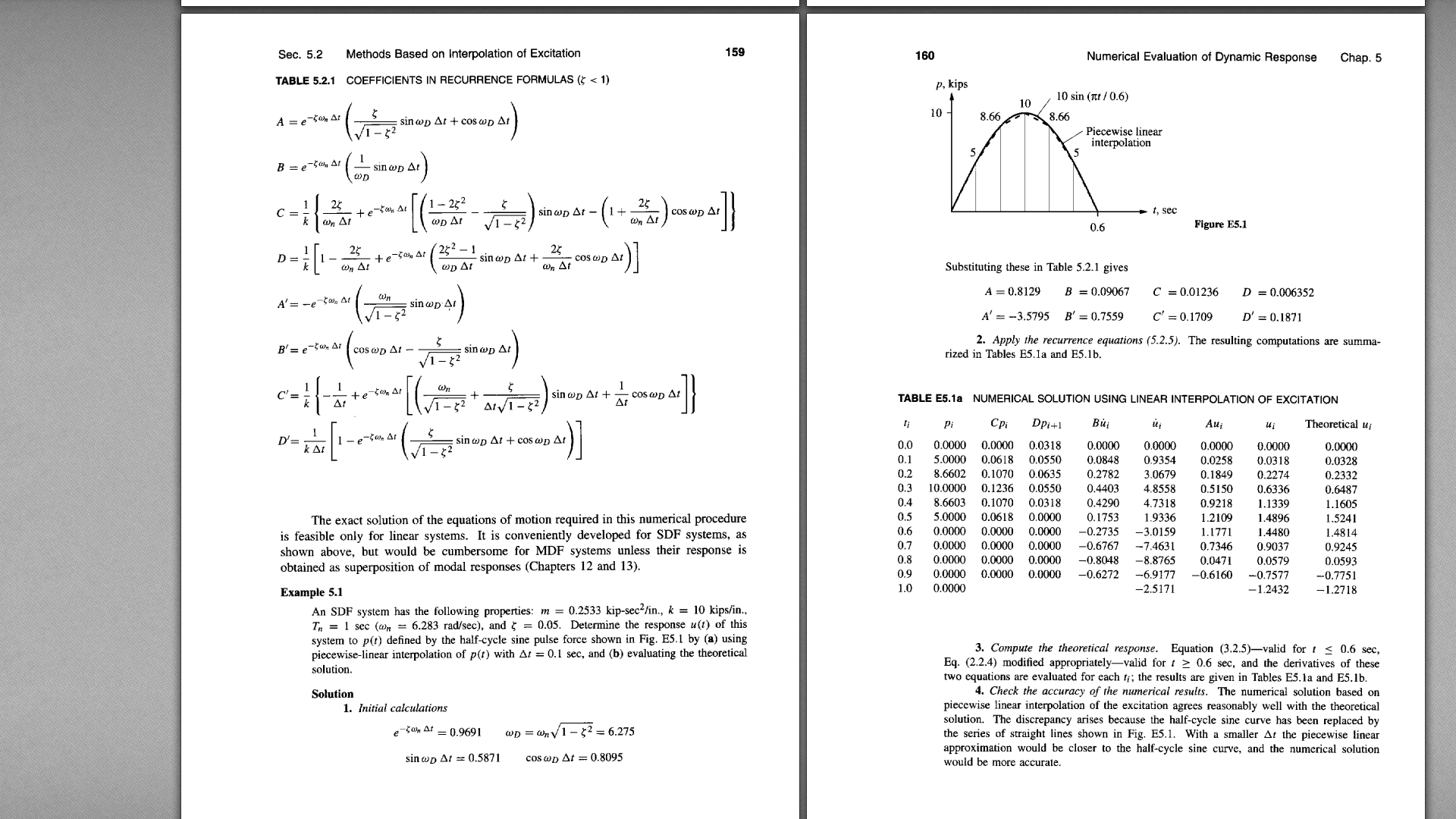
Here is the method that is mentioned by Author in the book, it is time integration method and since it has an example in it, it is very convenient for me to understand, everything is conceivable the glitch here is "C" and "D" coefficients includes "k" in their formula. I couldn't strip down that "k" because I don't know it, I was expecting simply 𝜉 and $T_n$.

enter image description here

enter image description here

enter image description here

So at the end how am I suppsed to obtain the deformation response history from given seismic ground motion?

Improve this question
$\endgroup$
4
  • $\begingroup$ Could you provide the text's description of u and p? You should find it in the preceding section 5.1. $\endgroup$
    – NMech
    Commented Jul 22, 2021 at 10:19
  • $\begingroup$ The numerical conditioning of equations 5.2.3 and 5.2.4 looks horrible. You shouldn't even think about calculating something that is approximately $\theta^2/2$ for a small value of $\theta$ as $1 - \cos\theta$, etc. I hope this isn't intended to be a practical numerical method for designing earthquake-resistant structures! $\endgroup$
    – alephzero
    Commented Jul 22, 2021 at 15:05
  • $\begingroup$ Updated, Since the eq. 5.2.2 is the RHS of motion equation it is obvious that this is the time dependent force equation, but I have to transform RHS into ground-acceleration as @kamran hinted out, I thought that it was simple since F=m*a(t) but this is where I'm failing. $\endgroup$
    – Sonya Blade
    Commented Jul 22, 2021 at 15:06
  • $\begingroup$ @alephzero ' I hope this isn't intended to be a practical numerical method for designing earthquake-resistant structures!' Probably it isn't not for practical purposes, just only to give some numerical examples but at the end, what Author refers to in his next sections (Section 6 Construction Of Response Spectra) is not consistent with each other, this solution method he proposes can not be solved with Section 5.2. $\endgroup$
    – Sonya Blade
    Commented Jul 22, 2021 at 15:15

3 Answers 3

Reset to default
2
$\begingroup$

I don't have the book so I don't know what the author means by "Methods Based On Interpolation of Excitation."

Practical methods for computer simulations can be based on Duhamel's integral. The idea is to find the impulse response of the system (which is simple for a damped SDOF model of the structure) and then apply an impulse to the structure at each time step to make the ground acceleration of the structure match the assumed (input) ground acceleration.

The size of the each impulse depends on the earlier motion of the structure during the simulation, of course.

In theory this can be reformulated in the frequency domain as a convolution integral, but Duhamel's method avoids the problem of trying to convert the ground acceleration data (measured in the time domain) into the frequency domain.

Improve this answer
$\endgroup$
2
  • $\begingroup$ To add to that, duhamel's integral approach essentially converts the general forced response into a problem of initial conditions, and as such it treats the response of the structure as a free vibration problem. The free vibration problem is only depended on $\omega$ and $\zeta$. $\endgroup$
    – NMech
    Commented Jul 21, 2021 at 11:55
  • $\begingroup$ Please check the main body of question, I've tried to elaborate it. Methods Based On Interpolation of Excitation seems more convenient to me, even though Duhammel's doesn't require changing the domain. $\endgroup$
    – Sonya Blade
    Commented Jul 21, 2021 at 14:15
1
$\begingroup$

The natural frequency of the system is given by

$$\omega_n=\sqrt\frac{k}{m}$$

So k and $\omega_n$ are related. There is no contradiction.

Improve this answer
$\endgroup$
1
  • $\begingroup$ You are correct, I was mistaken since period , angular velocity, spring stiffness and mass is correlated with each other. But the question still remains unanswered, how am I supposed to obtain the deformation response history from given seismic ground motion? $\endgroup$
    – Sonya Blade
    Commented Jul 21, 2021 at 14:13
1
$\begingroup$

You have to break the ground acceleration, $u_{gt}$ into simple segments like what your book example has done. The k if not given can roughly be estimated as a cantilever beam $k=\frac{3EI}{L^3} \ $ or calculating the force applied on top that causes the structure to deflect one unit.

There are some worked examples to calculate the stiffness of a complex structures.stiffness matrices

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.