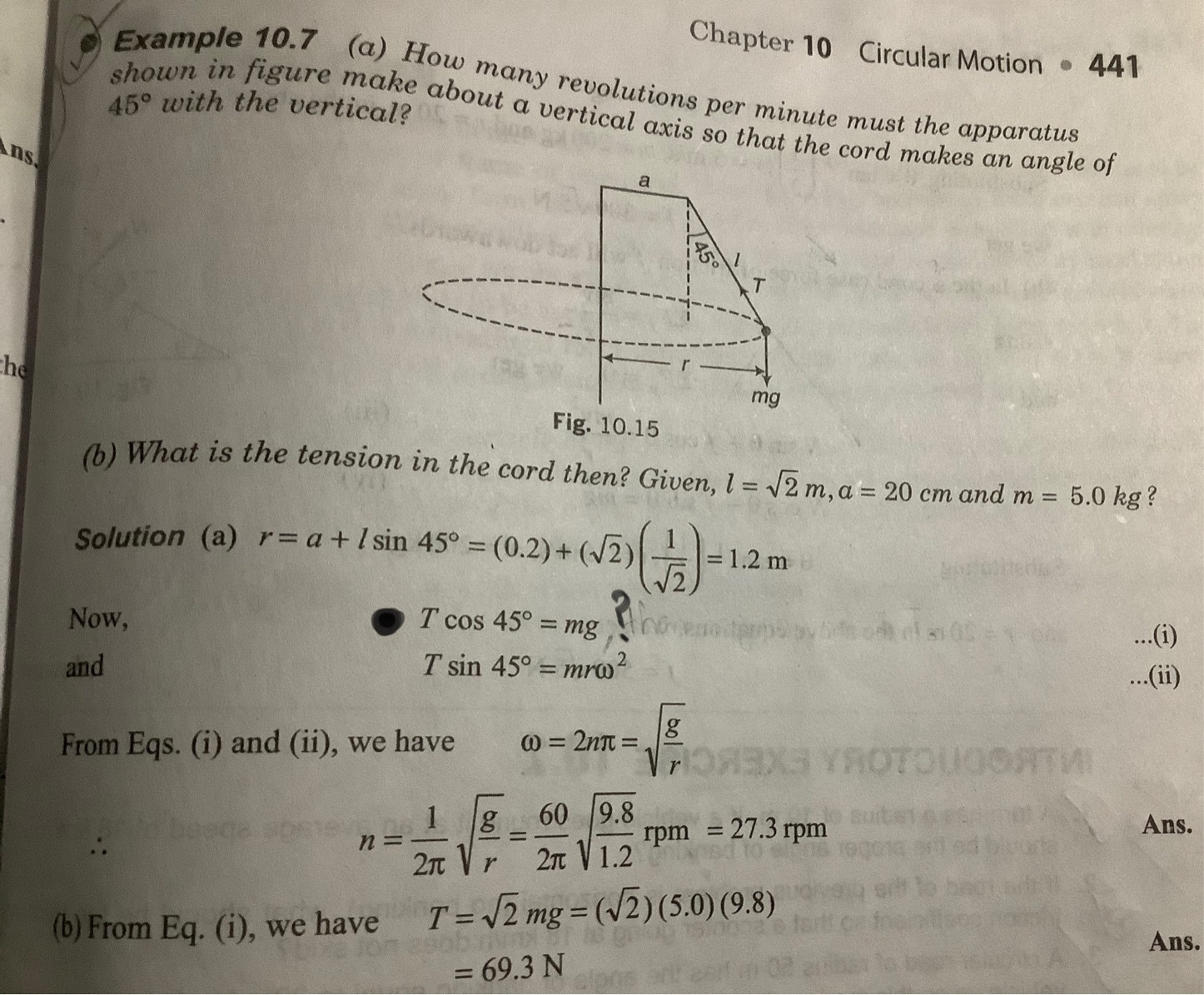
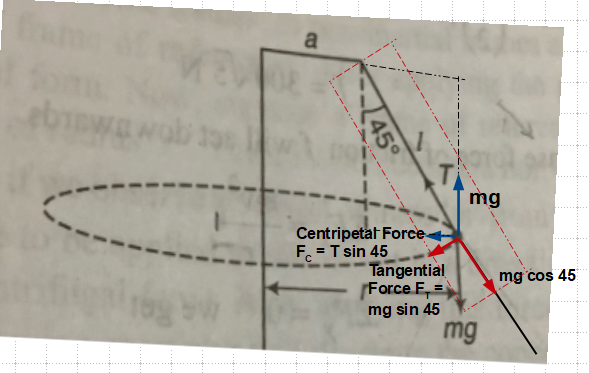
The following is a side and a top view of the problem (hopefully you understand the sketch).

- the green is the pole and wire
- the red is the circular motion trajectory
- the black are the forces.
- with light blue are the accelerations. Please note that $a_t=0$, and its only for illustration purposes in the image. As a consequence there is no force component on the tangential direction. This is also supported by the question which is about uniform circular motion.
If you analyze the forces on the side view you get:
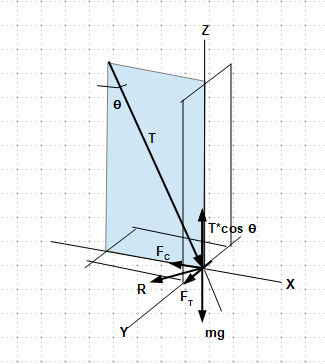
From the equilibrium on the vertical axis Z (there is no motion on the z axis , you obtain:
$$ \sum F_z = m\cdot a_z $$
$$ T \cdot \cos\theta - mg = m\cdot 0 $$
$$ T \cdot \cos\theta = mg \tag{eq.1}$$
From the (dynamic) equilibrium on the horizontal you obtain that:
$$ \sum F_n = m\cdot a_n $$
Where $a_n$ is the centrifugal and its equal to $\frac{v^2}{r}$ or $\omega^2 r$. Therefore:
$$ T\cdot \sin\theta = m\cdot a_n $$
$$ T\cdot \sin\theta = m\cdot \frac{v^2}{r} $$
$$ T\cdot \sin\theta = m\cdot \omega^2r \tag{eq.2}$$
If you put everything together:
$$(eq.2) \rightarrow T = \frac{1}{\sin\theta } m\cdot \omega^2r \tag{eq.3}$$
then susbstitutign the above t eq1.
$$(eq.1) \rightarrow \frac{1}{\sin\theta } m\cdot \omega^2r \cdot \cos\theta = mg \tag{eq.4}$$
$$(eq.1) \rightarrow \frac{1}{\sin\theta } m\cdot \omega^2r \cdot \cos\theta = mg \Rightarrow \tag{eq.4}$$
$$\omega^2 = \frac{\sin\theta }{\cos\theta}\frac{1 }{r} \cdot g $$
$$\omega = \sqrt{\frac{\sin\theta }{\cos\theta}\frac{1 }{r} \cdot g } $$
$$\omega = \sqrt{\frac{g }{r} \tan(\theta) } \tag{eq.5}$$
Therefore substituting eq.5, to eq.3:
$$T = \frac{1}{\sin\theta } m\cdot g \tan(\theta) $$
$$T = \frac{1}{\cos\theta } m\cdot g $$
$$T = \sqrt{2}\cdot mg $$
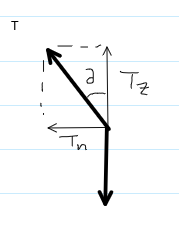
Also as Q2: can we say there is $a_t$ is along z axis for this question ?
if by Z you mean the vertical direction then NO. the $a_t$ will be on the horizontal plane and it will always be tangent to the red circle in the image.