4
$\begingroup$

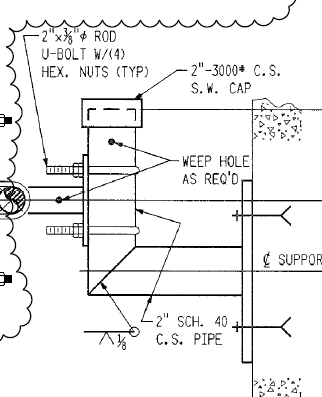
I'm attempting to calculate the clamping force from a u-bolt. This arises from an existing support configuration that is being re-qualified due to the installation of new equipment. I am stuck with this configuration unless it turns out to be truly impossible to re-qualify.

$\hspace{4cm}$Figure 1

$\hspace{7cm}$Figure 1

The u-bolts, as shown in Figure 1, are supporting a vertical load. This vertical load is being resisted only by the clamping force which exists from the 2 u-bolts around the vertically oriented 2" dia. Schedule 40 pipe.

My initial thoughts are to approach the qualification as I would for a bolt and nut in a slotted hole. That is to say, use the installation torque to calculate the bolt preload, and then use the preload along with an appropriate coefficient of friction to calculate the clamping load. This approach seems inadequate to me, but I don't really have a good reason why I feel that way.

Has anyone ever dealt with a similar issue? What method was used to calculate the clamping force?

Thanks.

Improve this question
$\endgroup$
2
  • $\begingroup$ Force is easy, as you suggested: it's whatever torque you used to tighten the nuts to their current position. Whether that provides sufficient holding force to keep the u-clamps from sliding down or rotating depends on the friction coefficient (and total contact area) between the clamps and the standpipe. There could be additional holding power if you physically deform the pipe, perhaps. $\endgroup$
    – Carl Witthoft
    Commented Sep 20, 2016 at 14:25
  • $\begingroup$ it seems to me it would be pretty simple to do a proof test to validate your estimate. $\endgroup$
    – agentp
    Commented Sep 20, 2016 at 14:44

1 Answer 1

Reset to default
1
$\begingroup$

I had to design these once to hold up a conveyor in a farm building. I didn't have that much load on there, so I specified to tighten the 4 bolts to 70% of their ultimate load (as required in CSA S16 for slip-critical bolts) in an X pattern. I then used 75% of the value of the ultimate load in tension for my bolt to find my normal load on the post, just to take into account any creep or fatigue that could arise. With the normal load, the area of my plate and the coefficient of friction, I found how much I could withstand.

I guess you should also check the tube for a thin point load if you have a lot of load; this wasn't my case!

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.