Note: This is no homework, but self-study.
I have a thermodynamics and fluid mechanics background and am trying to work through this book in order to get better understanding of turbomachines (especially for compressible flow). The book was praised across the internet as a classic and basically one of the best turbomachinery books out there. I immediately ran into problems and I think the book has errors. I give two examples and hope you can review them. I hope you can point out mistakes on my side, otherwise this would heavily discourage me to further work through the book.
First problem. So I reviewed the topics of chapter 1 and tried to solve the end-of-chapter problems. This is literally the first problem in the book (1a is easy, 1b has the supposed error).
1a. Air flows adiabatically through a long straight horizontal duct, $0.25 \text{ m}$ diameter, at a measured mass flow rate of $40 \text{ kg/s}$. At a particular section along the duct the measured values of static temperature $T = 150 \text{ °C}$ and static pressure $p = 550 \text{ kPa}$. Determine the average velocity of the airflow and its stagnation temperature.
1b. At another station further along the duct, measurements reveal that the static temperature has dropped to $147 \text{ °C}$ as a consequence of wall friction. Determine the average velocity and the static pressure of the airflow at this station. Also determine the change in entropy per unit of mass flow between the two stations. For air assume that $R = 287 \text{ J/kg/K}$ and $\gamma = 1.4$.
Solutions. 1a is simple: $v = \frac{\dot{m}}{\rho\,A} = \frac{\dot{m}\,R\,T}{p\,A} = 179.93 \text{ m/s}$ and $T_0 = T + \frac{v^2}{2\,\frac{\gamma\,R}{\gamma - 1}} = 439.26 \text{ K}$ which matches the book solution. But now for 1b: $h_{01} = h_{02} = \frac{\gamma\,R}{\gamma - 1}\,T_1 + \frac{v_1^2}{2} = \frac{\gamma\,R}{\gamma - 1}\,T_2 + \frac{v_2^2}{2}$ gives $v_2 = \sqrt{2\,\left(h_{01} - \frac{\gamma\,R}{\gamma - 1}\,T_2\right)} = 195.96 \text{ m/s}$ (not even given in the book solution, even though asked), and then $p_2 = \frac{\dot{m}}{v_2\,A}\,R\,T_2 = 501.42 \text{ kPa}$ (this also matches the book solution). Buut now for the entropy we should have, since we are treating this as a perfect gas, $$ \Delta s = \frac{\gamma\,R}{\gamma - 1} \, \ln{\frac{T_2}{T_1}} - R\,\ln{\frac{p_2}{p_1}} \hspace{1cm}\text{ (also given as Eq. (1.31) in the book})$$
But this gives $\Delta s = 19.39 \text{ kJ/(kg K)}$, while the book solution gives $39.24 \text{ kJ/(kg K)}$.
This I miss anything or does the book present a false solution right from the first problem?
Second problem.
This leads to problems in the end-of-chapter problems, but really appears to be a big typo in an important equation. I found it right in chapter two after turning a few pages from the first problem. On page 47 (7th edition) it says: For a compressor, the isentropic efficiency is defined in Chapter 1 and can be written as $$ \text{Eq. (2.10a)} \hspace{1cm} \eta_C = \frac{\Delta h_{0s}}{\Delta h_0} = \frac{((p_{02}/p_{01})^{\gamma / (\gamma -1)} -1)}{\Delta T_0 / T_{01}} $$.
Now ... apparently the exponent is switched around, correct (in my opinion) is $$ (p_{02}/p_{01})^{(\gamma -1) / \gamma} $$ from the isentropic change of state relations.
I would greatly appreciate your opinion on these two examples. I can't really work through a book when I must assume errors in the text. These I just found at first glance and in the first problems I tried, so this is really discouraging. Hope the errors are on my side!
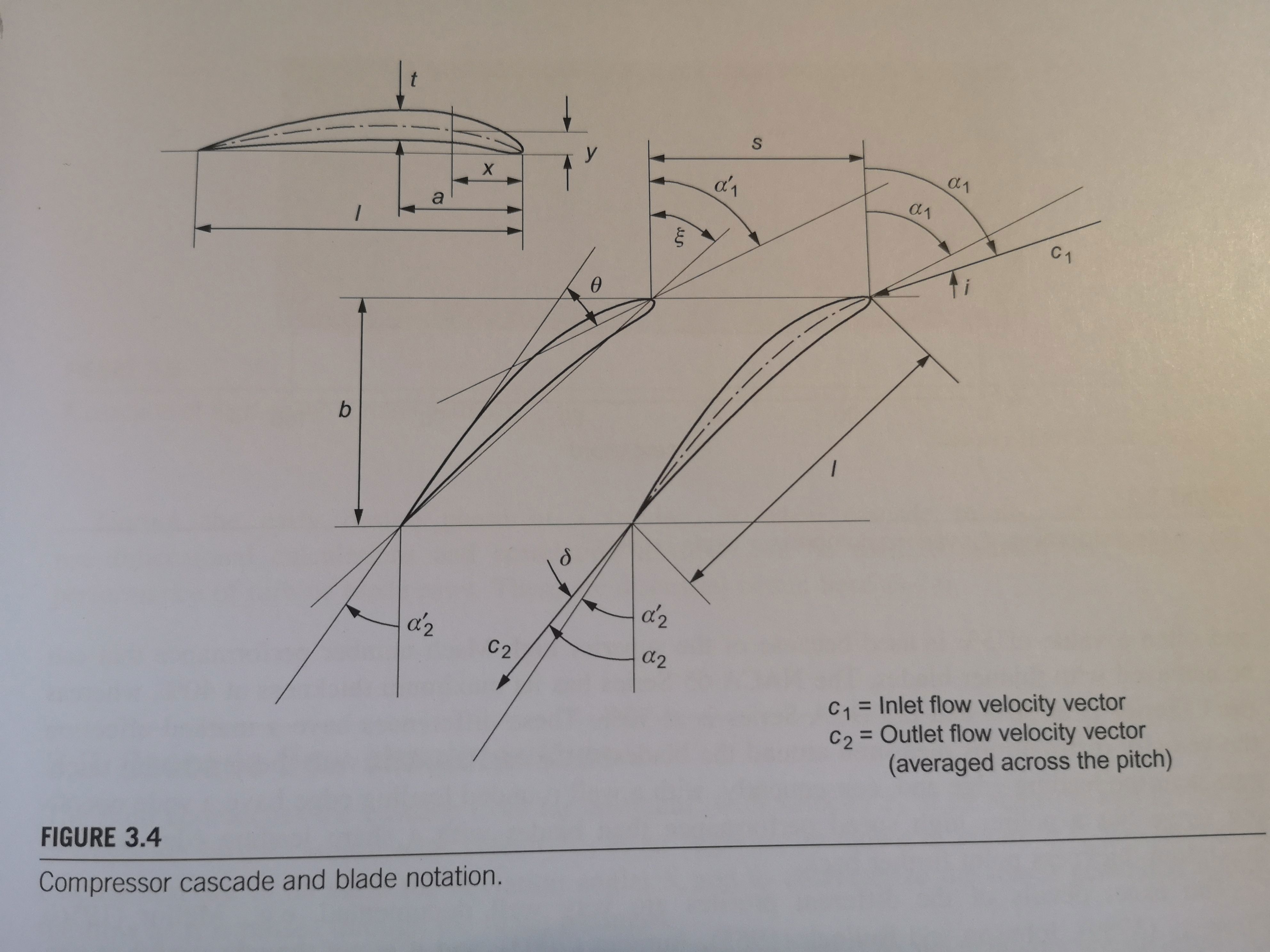
Edit: Third problem On the right, $\alpha_1$ is used twice, one of them needs to be $\alpha_1^{'}$.
Edit: Fourth problem Multiple references in the book, e.g. text references to figures 3.15 to 3.19 are wrong (numbers are wrong, e.g. 3.17 is used for 3.16).