Can over correcting power factor cause damage to equipment?

Question

If you over-correct your power factor, that is your power factor is leading, not lagging as expected by the utility, can you damage your (as opposed to the utility's) equipment?

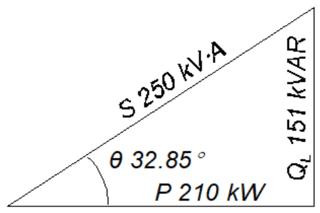
As we are all aware, Power Factor is the ratio of the actual electrical power being consumed in Watts and the apparent power, in VA (Volt-Amps). This site has a good description. The power factor angle, $\Phi$ can be expressed by:

$\Phi =sin(PF)$

The imaginary, reactive power $Q$ (from the power triangle), as measured in VAR (volt-amperes-reactive) can be expressed as

$Q=VA*cos(\Phi)$

While there are lots of snake-oil products around that use power factor correction to "save energy," power factor correction is a real concern for big consumers of electricity get charged for poor power factor. A big office building I used to work in had a kVAR-Hour meter in the basement. I was not involved with the billing but I imagine we got charged for kilowatt-hours and kiloVAR-hours.

This site says, "Overcorrecting power factor can lead to motor failure and costly equipment damage." I don't doubt that if you overcorrect and have a leading power factor, the utility will not be happy but causing damage? I can't see that happening.

If the web site were for anybody but a utility, I'd just roll my eyes and move on. In this case, I am quite confident that somebody at Cass County Electric Cooperative is solid in such matters but were they consulted in the preparation of the web site? Maybe, maybe not.

Answer 1

Your formulas are wrong.

$$pf = \frac {P}{S}$$

If you sketch the power triangle, $cos\ \theta = \frac {Adjacent}{Hypotoneuse} = \frac {P}{S}$, which means $pf = cos\ \theta$. Basic right triangles and trigonometry.

Similarily: $sin\ \theta = \frac {Opposite}{Hypotoneuse} = \frac {Q}{S}$.

This means $P = S\ pf = V I \ cos(\theta) $ and $Q = S\ sin (\theta) = V I \ sin (\theta)$.

The site is technically right, but mostly wrong.

You pay for kW (specifically kW•h) (210kW), but the power company produces kV•A (250kV•A).

$pf = \frac {P}{S}$, so it is a kind of an efficiency of a circuit. The closer it is to 1, the better for the power company.

Induction motors and fluorescent lights are inductive in nature, which means a lagging power factor.

During the commisioning process for a new building or after major renovations, the power company checks the power factor with all motors on. If the power factor is below 0.9 lagging, they add a surcharge for every kW•h purchased. This forces consumers to correct poor power factors with power factor correction (capacitors or over-excited synchronous motors). There is not much difference for the power company between power factors greater than 0.9 and 1, so power factor >0.9 is acceptable. It is usually not cost effective to improve power factor to 1.

Power factor correction can be done within the device (decreases current, which allows smaller wires and protection - device on/off correction on/off) or the full plant (decreases current for plant - possible issue Cass County Electric Cooperative are trying to illustrate). Buildings measure power factor per floor and add capacitors as required to correct power factor.

Example based on 200kW, 415V, 50Hz, 3-phase, squirrel cage induction motor with 0.84 lagging power factor and 95% efficiency. Rated three-phase current is 348.6A and supplied apparent power is 250kV•A.

If correct power factor to 1, power triangle goes away. S = P = 210kV•A. $Q_C = 151 VAR$. New current is 292.9A.

Within motor, current is 348.6A, but source provides 292.9A. So we can size wiring and protection, appropriately as required.

If the motor is not on AND power factor correction is on, the source provides 151 VARs or 210A to capacitors.

210A is less than 292.9A power factor corrected wiring and protection are sized for. For the Cass County Electric Cooperative claims to be true, pf must be less than 0.707 ($\theta < 45°$) and pf correction = 1. More specifically, inductive loads must be off and power factor correction on. So technically correct, but unrealistic.