I think there are multiple aspects to this question.
If I'm not mistaken, your concept would basically mean, that every floor is anchored to those 4 cables in the corners, thus basically "floating" in the air?
As a consequence, the upward force generated by those balloons would be (greater) equal to the weight of the floors. Something like this:
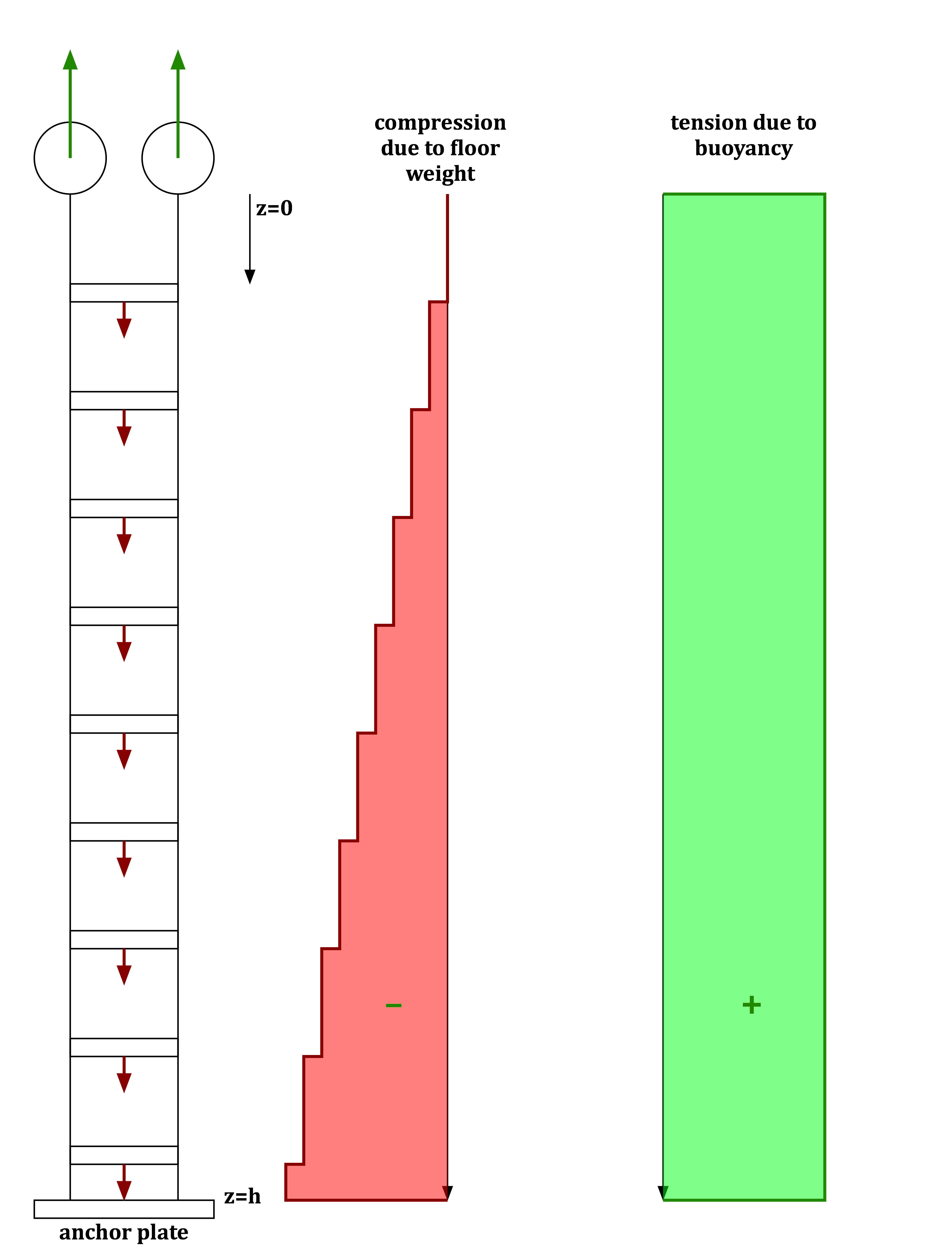
In this graphic I have neglected the tension due to the dead load of the cables.
stability and safety
Probably the biggest problem aside from statics would be the stability and safety of the structure; leaks, failure of a balloon, wind, etc.
statics
In normal conditions, Helium has a density of $\rho_{He}\approx0.179kg/m^3$, while air's is around $\rho_{air}\approx1.29kg/m^3$. If we neglect the weight of the hull of the balloon as well, we can come up with a net buoyancy force $f_B$ per cubic meter of Helium. $$ f_{B}=(\rho_{air}-\rho_{He})g=10.9N/m^3_{He} $$
If we take a $10m\times10m$ concrete floor, with a thickness of $t=0.2m$, and a density $\rho_c=2'500kg/m^3$, you would already need a balloon volume $$ V_{He}\approx45'000m^3 $$ which is a sphere of radius $r=22m$. An that is for one single floor.
availability of helium
Though being the most abundant element in the universe, helium is relatively rare on the earth. Currently, most helium is extracted from natural-gas, but those fossil fuel resources are limited. I'm not sure whether we earnings would want to risk losing such a precious resource.