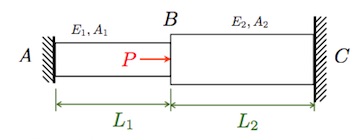
Here's the setup:
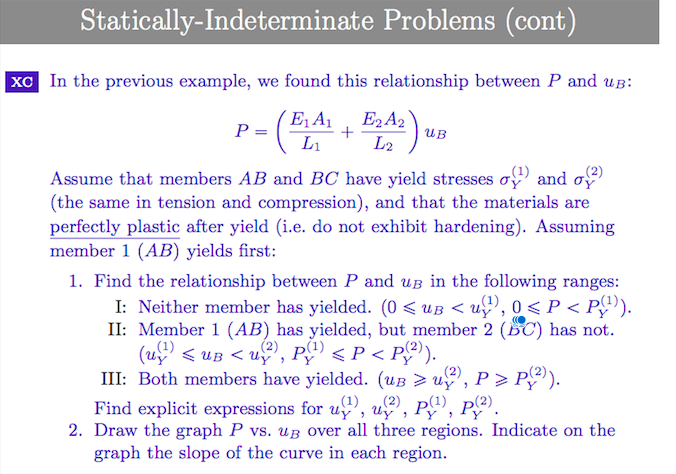
The problem asks to find the relationship between the force $P$ and the displacement of B ($u_B$). I have done this correctly:
$$P = \left(\dfrac{E_1A_1}{L_1}+\dfrac{E_2A_2}{L_2}\right)u_B$$
Now imagine that members AB and BC both have yield stresses ($\sigma_{Y_1}$ and $\sigma_{Y_2}$) and are perfectly plastic after yield.
I need to find the relationship between uB$u_B$ and P$P$ in the following cases:
- No member yields - ($0 < u_B < \sigma_{Y_1}$) & ($0 < P < P_{y_1}$)
- Member AB yields, but not member BC - ($u_{Y_1} < u_B < u_{Y_2}$) & ($P_{y,1} < P < P_{y,2}$)
- Both members yield - ($u_B > u_{Y_2}$) & ($P > P_{y,2}$)
I'm having trouble finding explicit expressions for $P_{y_{1/2}}$ and $u_{Y_{1/2}}$. I'm not quite sure how to relate yield stress to elongation and displacement of the members.
To be honest, this problem has tied my brain in a knot and I believe my conceptual understanding (or misunderstanding) of the problem is limiting my ability to solve it mathematically. Any help at all would be greatly appreciated!