I'm looking for a control method for a production process with the following characteristics:
- 1 control variable
- many procesprocess parameters (+-50)
- 1 resulting variable
- continuous measurement of resulting variable with 30-90 second delay
- complex physics that govern the process and numerous factors that determine the (non-linear) relation between control variable and resulting variable
With constant procesprocess/input parameters, the distribution of the resulting variable is normal.
The control method should ensure that the resulting variable should be above a set minimum and that the average of the resulting value should be as close to the limit as possible. Being above the lower limit makes the product more expensive, being below the lower limit however is more expensive: the product is scrapped.
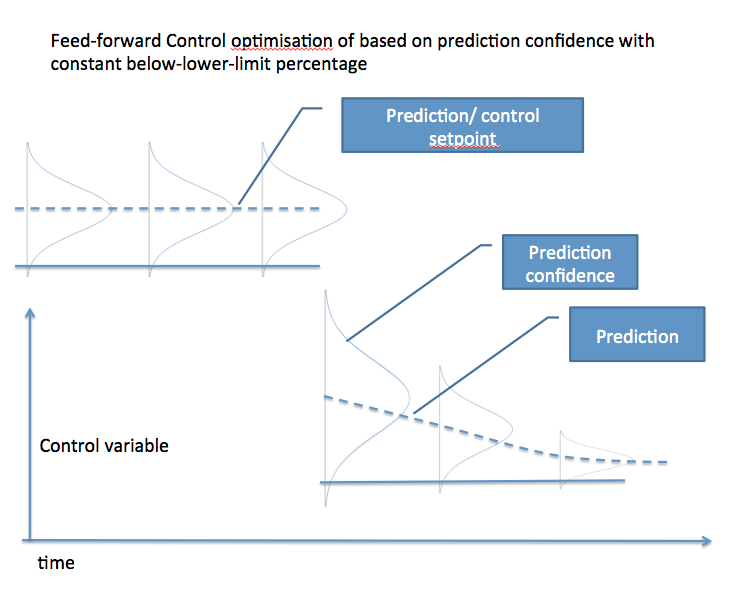
Now I want to try the following: I have want to make a statistical model using some procesprocess parameters as input predicting the control variable that leads to the desired value for the resulting variable. Next to the value of the control variable, I also want to determine the 'confidence' or distribution of that value. Then I want to state a portion of the time the control variable must be above the lower limit (i.e. 3 sigma). Consecutively I'd like to set the setpoint for the control variable at the such a value that I achieve the given portion above the lower limit. To illustrate what I envision I made the illustration below.
Do you think this setup makes any sense? Am I missing any obvious alternative solution that is much simpler? Is this a known approach?