If we have a fluid, is one force acting along the surface of it enough to cause sheering? In most diagrams I see for real Newtonian fluids they show two forces of equal magnitude along the top and bottom, but most problems I have done involve a single force acting over a real fluid, such as a block acting on a liquid.Is the deformation requiring two opposite forces?
3
-
$\begingroup$ Aren't there always two forces? "for every force there is always an equal and opposite force"... $\endgroup$– Solar MikeCommented Oct 13, 2022 at 5:24
-
$\begingroup$ @SolarMike And inertia produces the opposite force in an object when there is no second obvious force. $\endgroup$– DKNguyenCommented Nov 10, 2022 at 17:35
-
$\begingroup$ @DKNguyen but do you think the OP understands? $\endgroup$– Solar MikeCommented Nov 10, 2022 at 17:36
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
A single force on a material is sufficient to cause shearing; the material will begin to translate and (if the force isn't applied to the center of mass) rotate, and a shear stress state will arise internally because of the material's inertia.
In the context of standard elasticity, where the material is assumed to remain motionless, fully four forces are required: As noted above, one would cause translational acceleration, and adding an equal and opposite force (to produce a couple) would cause rotational acceleration.
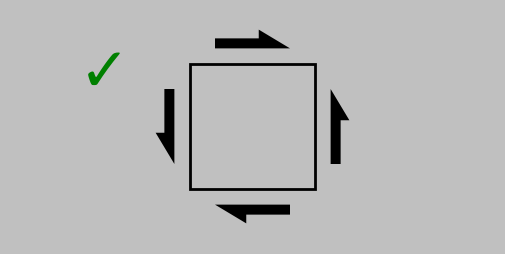
Although we often see only two force arrows in schematics of sheared solids, this is because the orthogonal stabilizing forces are ignored (e.g., if the material has a large lateral extent, only small stabilizing forces building up at the ends are required to produce a sufficient offsetting moment), not because they don't exist.
$\begingroup$
$\endgroup$
3
Shear can occur thru two opposite forces, or a single force on the medium that lay against another medium/solid. The result of the latter is represented by a force and a reaction (due to shear friction) in the opposite direction.
-
$\begingroup$ That would make sense, because in the block example, indeed there is a small motion in the fluid against a ramp. $\endgroup$ Commented Oct 11, 2022 at 16:08
-
$\begingroup$ A fluid forced through the pipe is another example. $\endgroup$– r13Commented Oct 11, 2022 at 16:51
-
1$\begingroup$ Not even another medium/solid is required. I can shear a material exposed to vacuum simply by lateral movement of its underlying support. $\endgroup$ Commented Oct 12, 2022 at 19:41