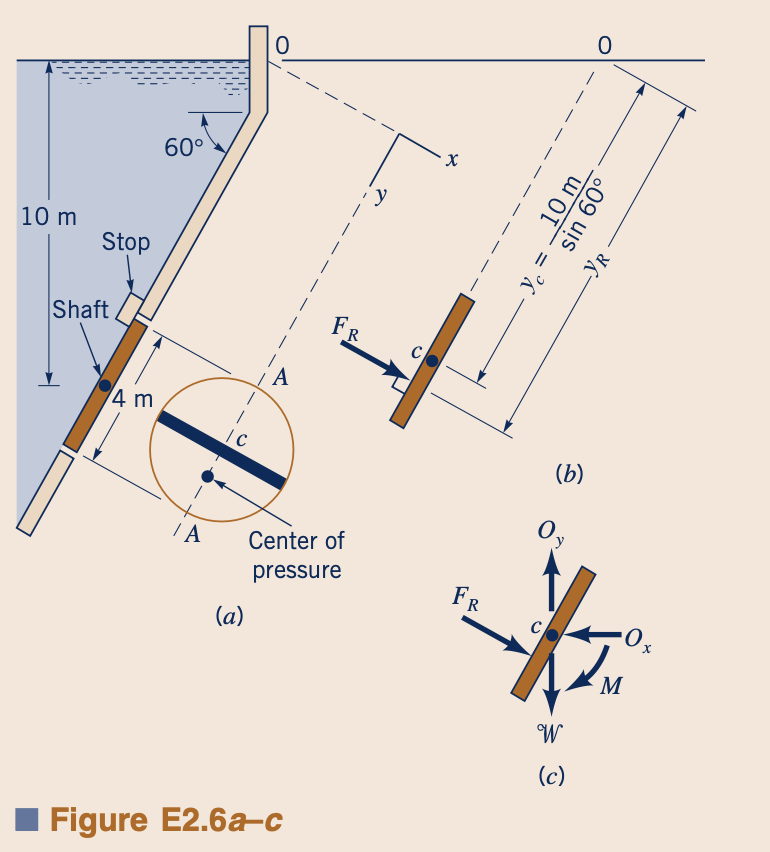
I am presently studying fluid statics from Munson's textbook. I cannot seem to understand part (b) of example 2.6. The full question is as follows:

GIVEN The 4-m-diameter circular gate of Fig. E2.6a is located in the inclined wall of a large reservoir containing water ($\gamma = 9.8 \mathrm{kN/m^3}$). The gate is mounted on a shaft along its horizontal diameter, and the water depth is $10\mathrm{m}$ above the shaft.
FIND Determine
a) the magnitude and location of the resultant force exerted on the gate by the water and
b) the moment that would have to be applied to the shaft to open the gate.

with the associated diagram

I had a few questions about this problem. Firstly, what is the role of the shaft? Is it used to hold the circular gate in place? Can the gate rotate freely about the shaft in the absence of the stop? The author writes the following in his solution to the problem

The moment required to open the gate can be obtained with the aid of the free-body diagram of Fig. E2.6c. In this diagram $\mathcal{W}$ is the weight of the gate and $O_x$ and $O_y$ are the horizontal and vertical reactions of the shaft on the gate.

It appears that his convention for $x$ and $y$ is different than the coordinate system he has used. That aside, I understand where $O_y$ comes from; I guess it is the reaction force to the weight $\mathcal{W}$. However, it is not clear to me where $O_x$ arises from. Is it a reaction force to the horizontal component of $F_R$? It doesn't seem like that to me. Next, the author writes

We can now sum moments about the shaft
$\sum M_c = 0$

I assume this implies an equilibrium condition at which point the shaft just opens. I also assume we discount the presence of the stop since it, in the current state of the gate, only prevents anticlockwise rotation of the gate.

Any kind of inputs and explanations would be most appreciated.