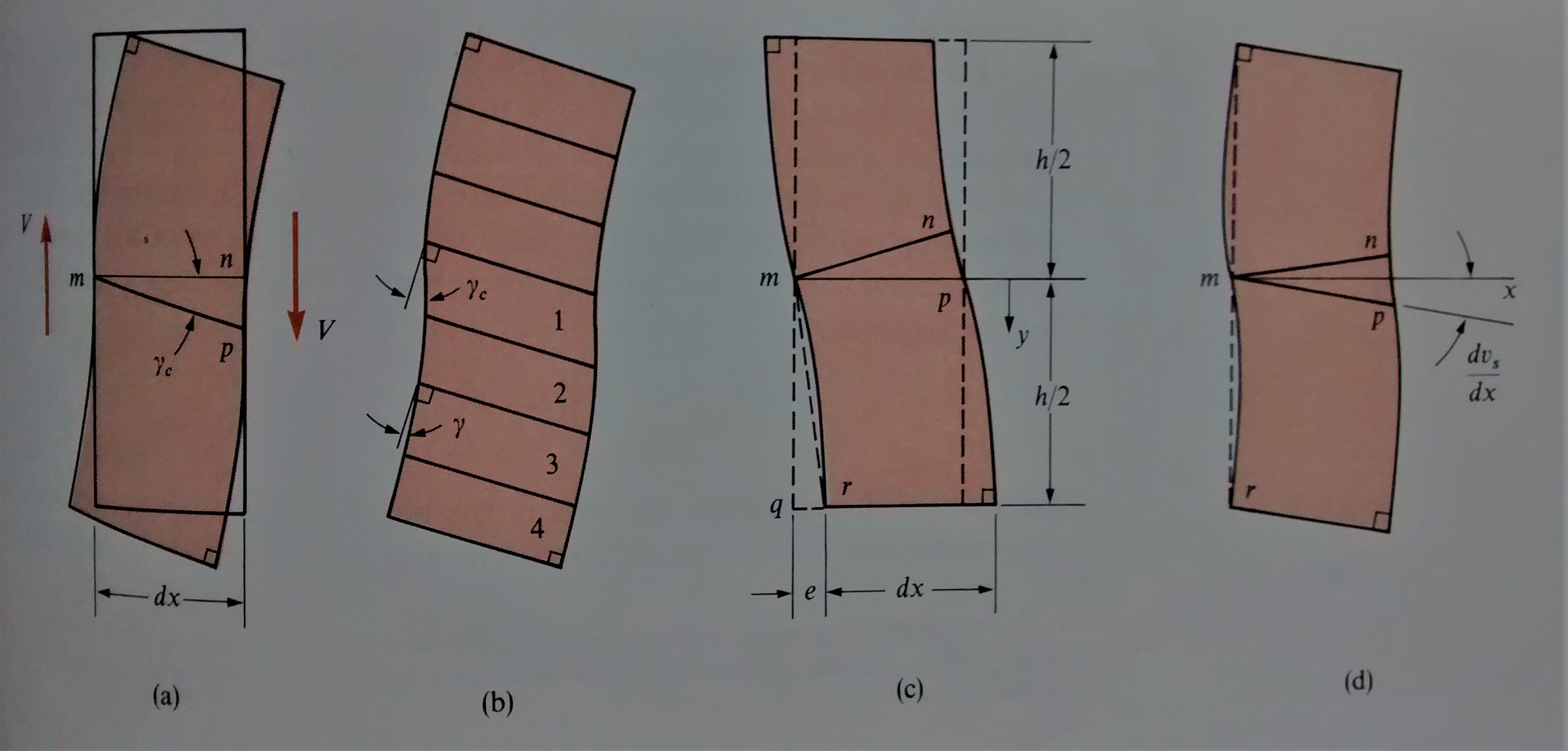
On top of flexural deflection, the Timoshenko Beam Theory includes a term called "Shear Deformation" that is to account for the additional deflection and shear stress due to shear effect, which is quite samall compared to the effect from the flexural, thus it is usually ignored except higher accuracy is desired. (See figure below)
The slope of the deflection curve of the beam due to shear alone is approximately equal to the shear strain at the neutral axis. Thus denoting by v_s the deflection due to shear alone can be evaluated through the expression for the slope:
dv_s/dx = r_c = alpha_s*V/GA
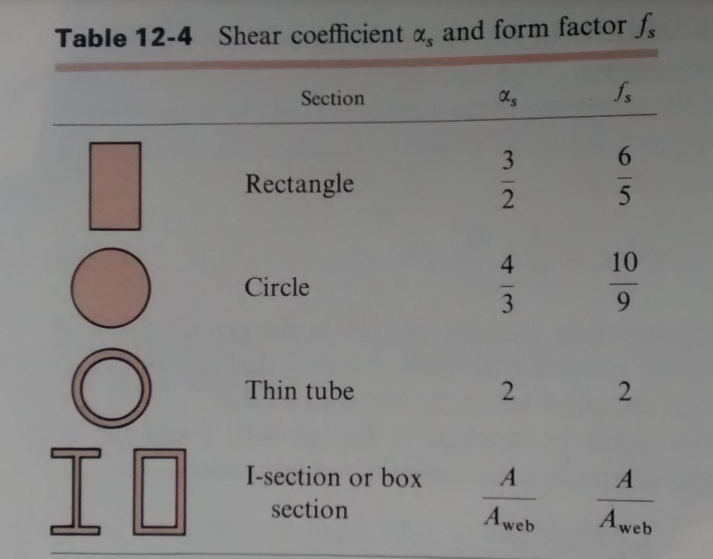
In which the "alpha_s" is called "shear coefficient" by which the average shear stress (V/A) must be multiplied to obtain the shear stress at the centroid of the cross-section. The shear coefficient for a rectangle cross-section is 3/2 (isn't it familiar?), and is 4/3 for a circular cross-section.
However, shear deflection obtained by solving the equation using the unit load method is considered an approximation for two reasons:
The deflections are based upon the shear strain at the neutral axis, hence, they do not take into account of variation in shear strains throughout the height of the beam.
The deflections are based upon a bending theory derived for the case of "pure bending" only.
The second defect can be remedied only by the more exact methods of the theory of elasticity, whereas the first can be eliminated by using the principle of virtual work, which is expressed as:
Wv = ⌠VvVL/GI^2 [⌠(Q^2/b^2) dy dz] dx
To simplify the expression, a cross-sectional property was introduced:
fs = A/I^2 ⌠Q^2/b^2 dA
fs is called the "form factor" for shear. The form factor is a dimensionless quantity that can be evaluated for the particular shape of beam. It is 6/5 for a rectangle cross-section, and 10/9 for a solid round beam.
Here is a table of the shear coefficient and shear factor for a few typical cross-sections.
Reference: "Mechanics of Materials", 2nd, Gere & Timoshenko. CH 7 & CH 12.