Questions about the level flight lift equation

Question

In level unaccelerated flight we have relation $$ W=L=\frac{1}{2}\rho\cdot V_{stall}^2\cdot S\cdot C_{l,max} $$ taken from book Daniel P Raymer "Aircraft Design: A Conceptual Approach" equation (5.5) at page 85.

The question is: why does this formula use $C_{l,max}$ and not $C_{l,min}$? In fact in the case that the $C_l$ in use is not $C_{l,max}$, and the speed is little more than $V_{stall}$ (valued for $C_{l,max}$), it happens that the lift is not enough and stall happens on aircraft anyway. If using $C_{l,min}$ the calculation is more cautious.

Answer 1

Disclaimer: I am no expert on aviation, I solely got this information via your paper and a little bit of research about the used variables.

First of all I can't follow your deduction. If you would have $V$ higher than $V_{stall}$ and $C_l$ lower than $C_{l,max}$ the equation should still hold. What you decrease with $C_l$ you increase squared via your velocity. So all in all the left side should not decrease and therefore $L$ should not decrease.

Furthermore the paper states

Equation (5.5) states that lift equals weight in level flight, and that at stall speed, the aircraft is at maximum lift coefficient.

So this is rather a deduction than an assumption to base design on. So for level flight for a given $V_{stall}$ you cannot decrease $V$ any further without increasing the angle of attack. However since you already are at $C_{l,max}$ you risk stall if you do decrease speed further.

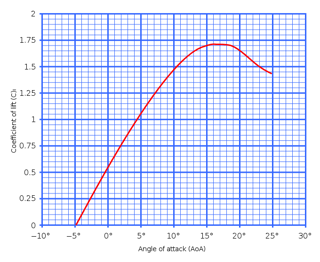
This is now in contradiction to Carls answer: The pilot would then have to extend flaps in order to fly slower than stall speed because the angle of attack cannot increase further.

Values range from about 1.2 to 1.5 for a plain wing with no flaps to as much as 5.0 for a wing with large flaps immersed in the propwash or jetwash.

If I understand the paper and my research correctly, the misconception here lies within the assumption that you would use the equation with given values and disregarding that the values are not independent from one another.

$$C_l=\dfrac{L}{\frac{1}{2}\rho v^2S}$$

Is just rearranged to solve for $L$. You do not choose $C_l$ but you determine it experimentally. See this reference

One way to deal with complex dependencies is to characterize the dependence by a single variable. For lift, this variable is called the lift coefficient, designated "Cl." This allows us to collect all the effects, simple and complex, into a single equation.

I hope this shed some light on your question.

Answer 2

My interpretation is simply that no idiot would try to decrease flight speed close to stall speed without first dropping all flaps, i.e. max lift coefficient.

In the general case, again if I understand that excerpt from the book, you could calculate the stall speed for any given wing configuration and use that lift coefficient.

Answer 3

Reading this section (5.3) of the book carefully, you will note that equation 5.5 is a stepping stone. The author is pointing out that, at stall speed, there is a relationship between Cl, the weight of the aircraft and velocity. He goes on to conceptualize the ability to use lift devices to modify Cl in equation 5.7. Given that this is aircraft conceptual design, he is providing insight into the relationships that must be developed by the engineer to move from a vehicle gross weight, a takeoff/landing speed and a wing lift coefficient.

The wing lift coefficient will later be turned into a relationship between angle of attack and Cl (a polar), and from there into a tool by which airfoils may be selected to provide optimal performance of lift and drag over various regions of flight.

I understand your confusion, based on where he goes with the rest of the section I think equation 5.5 would have been better stated if he had rearranged it to solve for Cl, rather than stating the equation in terms of lift force required. However, your statement about Cl,min ignores the definition of stall. At the point of stall (angle and velocity combination), there is a decrease in lift coefficient either way (increased or decreased angle). As such, the minimum condition for flight (and hence the minimum takeoff velocity) is given by the relationship in equation 5.5.

https://en.wikipedia.org/wiki/Stall_(fluid_mechanics)#.E2.80.9CStall_speed.E2.80.9D