Is bending stress over round thin-walled tube cross section linear?

Question

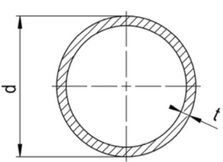
It is thin walled because roughly d=200mm and t=6mm of a carbon fiber wrapped tube.

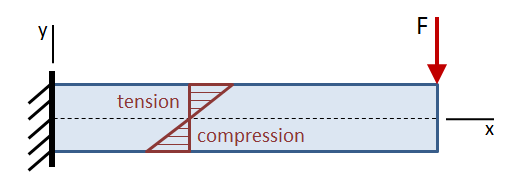
The question is, if the bending stress $\sigma$ over the cross-section (in y-direction) is linear or not.

It is clear to me that the bending moment over the cross-section must be linear (as depicted in the top picture), but because of the relation:

$\sigma_{b} = \frac{M_{b}}{W_{b}}$ and in this case $W_{b} = \frac{I_{xx}}{d/2}$

i am unsure if the bending stress would also be linear because the second moment of area $I_{xx}$ is defined as $I_{xx}=\int y^2 \, dA$ which surely isn't linear for the given thin walled tube cross-section, right?

If my train of thought is correct, then what would the form of the bending stress diagram over the cross-section?

Context: I try to measure the bending stresses of a CFRP tube without knowing the position of the main axis of the load. In turn, I will be using 3 strain gauges around the outside of the tube spaced 120° apart. But I need to know the distribution of the bending stresses across the cross-section to see if and how I can calculate the position and amplitude of maximum bending stress from these three measurements.

Answer 1

The stress diagram across the x-section is linear.

$\sigma = \dfrac{My}{I}$　

Note that the equation for "Moment of Inertia for Circular Cross-Section" is I=πd4/64, and a pipe's moment of area is calculated similarly given by I=π(D4-d4)/64, where D=Pipe OD and d=Pipe ID.

Answer 2

Just to add on to what r13 mentioned, with your tests, I am sure you will find that the strain and calculated stresses vary with location on the outer and inner skin of the tube. If you consider the formula at y=0, what will the stress be? I think you see where I am going as your post has an excellent diagram showcasing this point.

Further, you can pick up any Machinery's Handbook and find the formula for stress at any point: s=W*(l-x)/Z.