I am reading Modern Compressible Flow by John D. Anderson Jr and I am going through the integral form of the continuity equation, I am struggling to wrap my head around the fact that said form has two integrals associated with it. I have come across the expression before, but I just hadn't stopped to think about why it has two integrals, any help will be appreciated. My impression has been that when we use the infinitesimal fluid element approach where the fluid element is on a blob (random geometric shape), we use a single integral to show that we are summing the fluid elements over that blob but with the two integrals, that's throwing me off.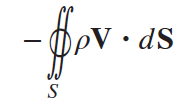 
$\begingroup$
$\endgroup$
2
-
$\begingroup$ A surface integral doesn't have two integrals. It is a concept with its own symbol (and its own meaning) as you have posted. But one way to evaluate some surface integrals is to express the surface as a function of two variables, then integrate a functional expression defined parametrically in those two variables over the surface bounds. Then check to see if reversing the order of the variables in the integration changes the result. If it doesn't, the answer is probably correct. If it does, one of the answers may turn out to be useful, but you have to figure out which, if either, serves. $\endgroup$– Phil SweetCommented Dec 8, 2023 at 1:25
-
$\begingroup$ But there are many situations when this approach simple doesn't work. You may need to define the surface, or the functional, in other ways in order to get an answer. Green's theorem is a common example. $\endgroup$– Phil SweetCommented Dec 8, 2023 at 1:29
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
0
If you are doing surface integrals starting from single dimensional infinitesimals, then you need two integrals because areas are two dimensional. If your integral is formed such that it already happens to start with an infinitesimally thick slice of the surface then you don't need two integrals because part of the work is already finished.
Essentially, with two integrals you are building up from linear dimensions, to a slice, and to an area. The first integral integrates any one a slice of the surface. The second integral integrates all the slices to form the entire surface.
It progresses the same way to volume integrals, where the surface you have after two integrals is actually a cross section of the volume. Then the third integral adds up all the cross sections to get you the volume.
