1
$\begingroup$

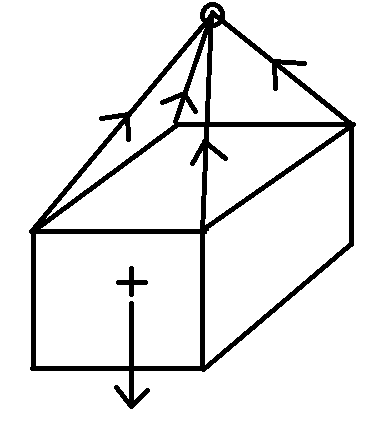
I have a solid object that has been lifted to a hieght by 4 strings. I know the lifting point and the 4 corners of the object. Also the mass and the center of the gravity location of the object is known. I need to find out the tensile forces on the string. enter image description here

The system has 4 unknowns i.e. the tensile forces of the string and I have 6 equations (3 force equations and 3 moment equations). I am not able to understand how to solve the system.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Start by showing what you have done. $\endgroup$
    – Solar Mike
    Commented Jul 28, 2022 at 21:09
  • $\begingroup$ How far is the lifting point, where the four strings meet from the top of the object? The angle of the strings relative to the weight will make a big difference. II would start by assuming the distance is infinite (or you have four strings going all the way up) and when you work that out, you can add in the angles and resolve the components. $\endgroup$
    – user1683793
    Commented Jul 29, 2022 at 0:40
  • $\begingroup$ Required is a dimension of distance ratio of string to object, or angle and mass, then tensile forces may be computed by the angle of the string above the top surface $$f=m/4cos\phi$$ $\endgroup$
    – Tony Stewart EE75
    Commented Jul 29, 2022 at 1:11
  • $\begingroup$ Also take in account that this system is statically indeterminate. $\endgroup$
    – Vladimir
    Commented Sep 1, 2022 at 11:07

2 Answers 2

Reset to default
1
$\begingroup$

assuming symmetry thus the CG of the cube in it's center, the tension in the cable is m/4 * the ratio of the length of the diagonal cable to the height of the cone.

$$T = (m/4)* \sqrt( (a/2)^2+(b/2)^2)+c^2)/c$$

  • a= long side of the box
  • b= short side
  • c= distance from the top of box to connectio point of cables.
  • m = mass of the box
Improve this answer
$\endgroup$
1
  • $\begingroup$ Wrong. that's the best approximation we can make, but if you make one string 1mm longer than the others it will take less load. $\endgroup$
    – Greg Locock
    Commented Dec 29, 2022 at 21:59
0
$\begingroup$

enter image description here

$$mg=4T\cos(\phi)\hspace{0.5cm}\Rightarrow\hspace{0.5cm}T=\frac{mg}{4\cos(\phi)}$$ Also, the ropes must hold additional loading from acceleration when lifting the payload.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.