0
$\begingroup$

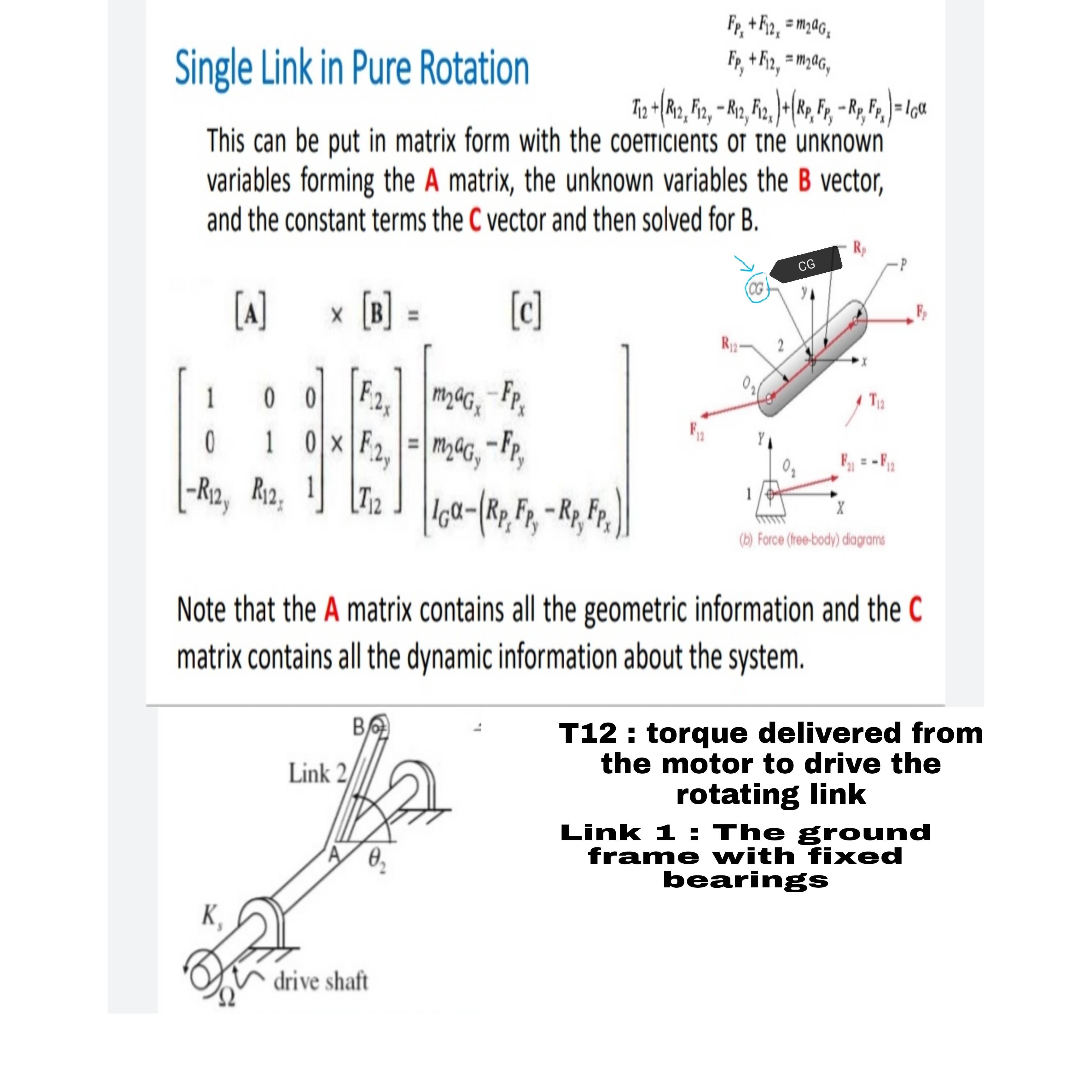
Below is the dynamic analysis for a single rotating link which is driven by a motor. It shows the 2d front view and the 3d full view. The equations for the analysis require knowing the location of the CG (center of gravity) as it will be included in the torque equation. Here, the centre of gravity location for this link is half of the link's length; because this link is symmetrical. I noticed that the effect of the shaft's mass, centre of gravity, and moment of inertia, are all not taken in our considerations for link 2. It is like dealing with link 2 as if it's a seperated rigid body from the shaft. While it's actually a part of the motor shaft, since they are rigidly connected and they do rotate at same speed taking their source torque or power from the source motor, and transmit their force to bearings (link 1) directly through the shaft.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

I think this is a steady state analysis (constant rpm) without gravity. In such a case, if you rotate just the shaft at constant rpm, there will be no forces. Only thing that can introduce forces there is a part with center of mass offset from the rotation axis, which in this case is the link.

In reality, the shaft mass would probably matter, but its effects would not be easily calculated using steady state analysis.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.