2
$\begingroup$

This is a truss I simulated using an online calculator. I tried to calculate all the member forces using F=ma=0 in both x and y direction. However, I found a different set of solution which also satisfy all of the 8 equations I described below and checked the equation with online calcualtor value which also satisfy the 8 equations. Is it possible that it can yield 2 different set of solutions or is it unstable? Give it a try :)

Thank you for reading

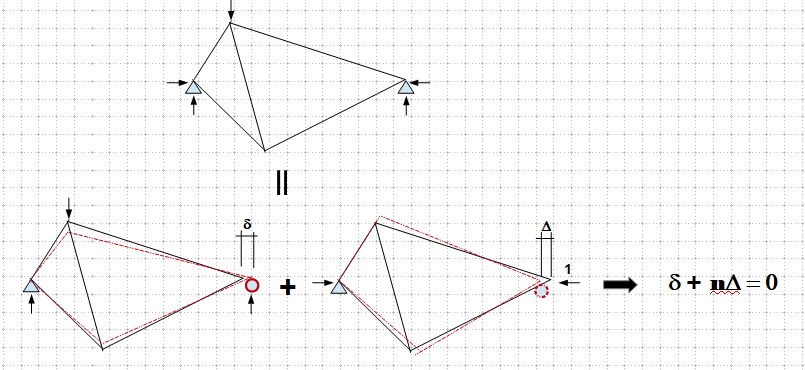
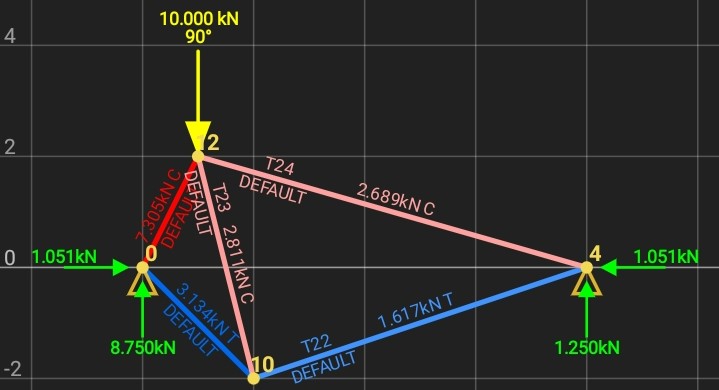
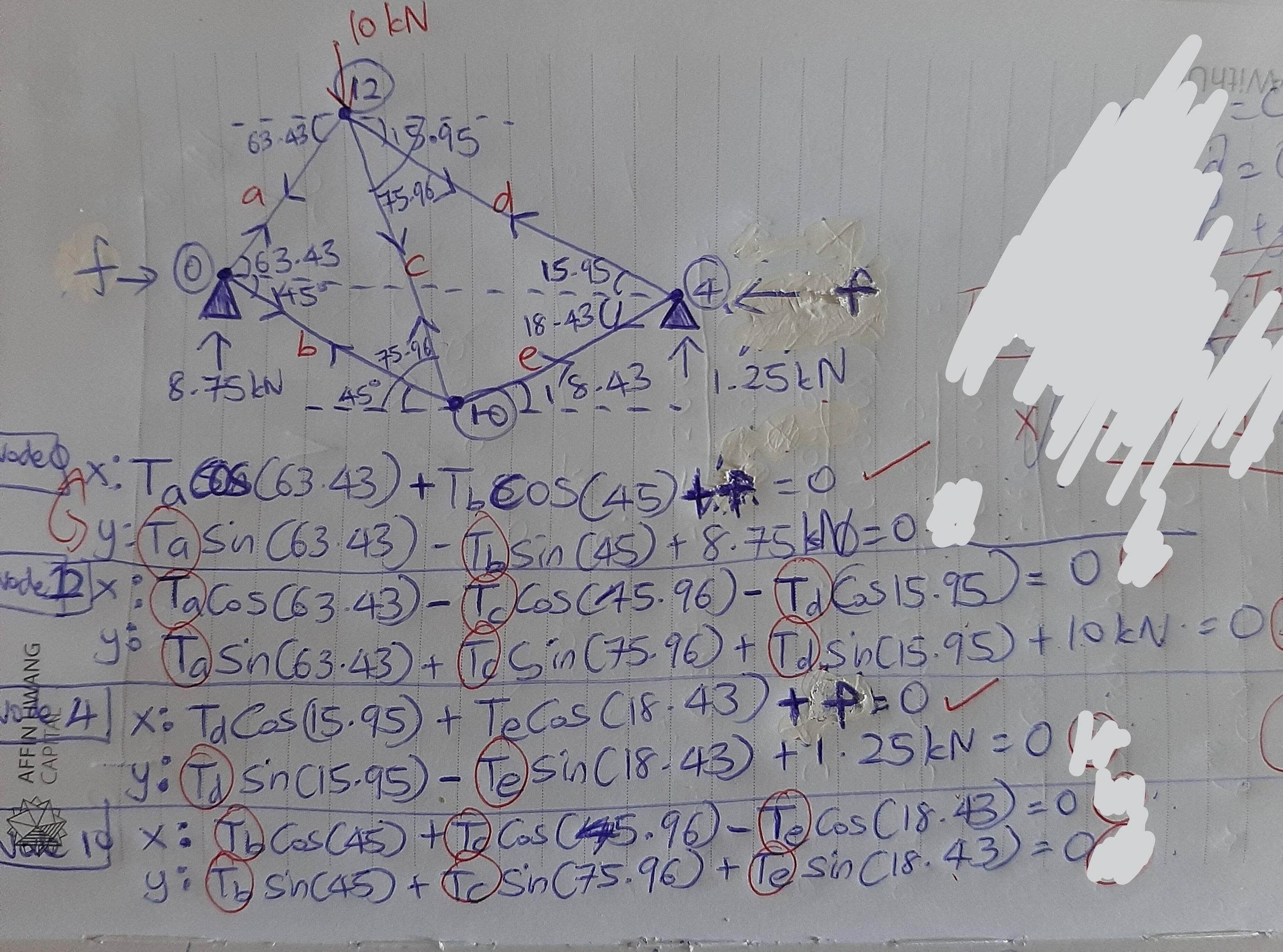
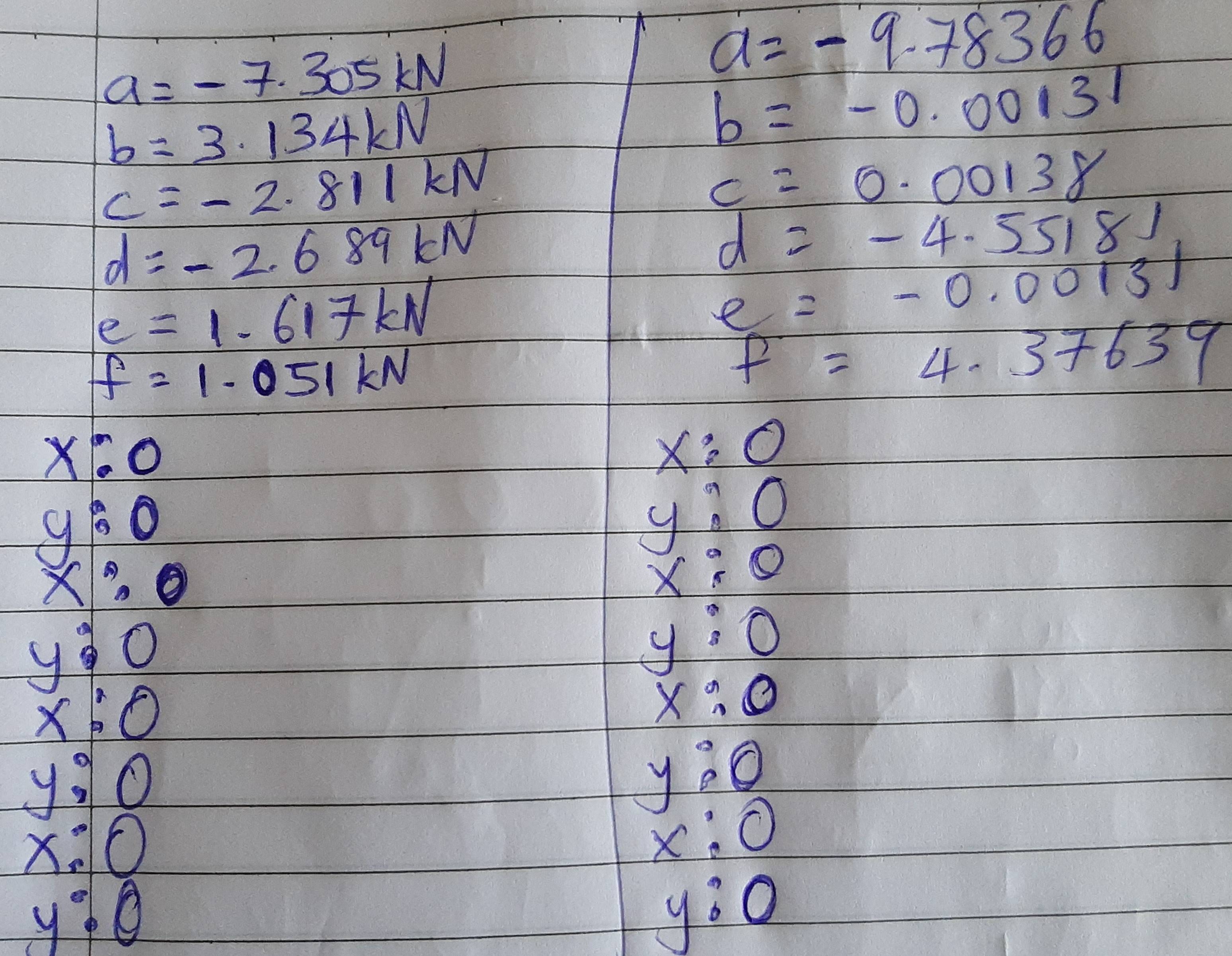
enter image description here enter image description here

enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Welcome to Engineering! A single structure can only have one valid solution, so one of your results is incorrect. One thing I've noticed is that your computer model is hyperstatic (statically indeterminate), with 2 vertical and 2 horizontal reactions. Is this correct? Trusses are usually externally isostatic (statically determinate), with only three reactions (usually one support resists displacements in X and Y and the other only in Y), so you'd get zero horizontal reaction forces. $\endgroup$
    – Wasabi
    Commented Apr 11, 2022 at 14:41
  • $\begingroup$ Take a look at this question which may help shed some light on how to determine if a truss is determinant or in determinant. Indeterminant structures can still be solved, they just require a modified/different approach. $\endgroup$
    – Forward Ed
    Commented Apr 11, 2022 at 16:35

1 Answer 1

Reset to default
2
$\begingroup$

For a stable structure, there is only one set of solutions corresponding to the applied loads. You made mistake in performing the calculation on an undetermined truss system using the method for determinate, simply supported truss. Here is a simple demonstration of the two systems (determined and undetermined) with the question - are they yield the same results.

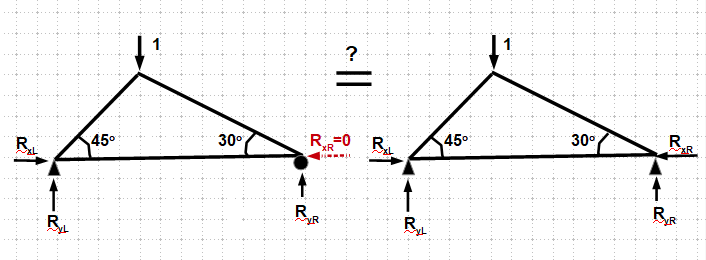
enter image description here

The answer is "NO", the results, for the two systems that have different support types, are different. So what is missing?

Your truss system has 4 unknown reactions (same as the right side sketch above) with only three known equilibrium equations, so this truss system is structurally indeterminate to the first degree.

In order to solve this system accurately, you need to write a displacement compatibility equation (a condition) by:

  1. make it a structurally determined, yet stable, system by releasing one support restrain (in this case, the horizontal restrain at either support), and perform the analysis (as you did).

  2. calculate the lateral displacement of the support, which was released of horizontal restrain, using the member forces obtained.

  3. with the applied load removed, apply a unit horizontal load on the released support and calculate the member stress and displacement of the support.

From step 3 above, you can apply the close-the-gap concept and superimpose the results of the analyses to arrive at the correct answer.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ I believe there is a much easier method I google which is indeterminate truss can be solved using stiffness matrix but it requires the value of E, elastic modulus and cross-section area of beam to be known :) $\endgroup$
    – Jasmine Su
    Commented Apr 11, 2022 at 16:41
  • 1
    $\begingroup$ Yes, if you know the stiffness method. The conclusion will be the same provided that you've specified the boundary conditions correctly. $\endgroup$
    – r13
    Commented Apr 11, 2022 at 18:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.