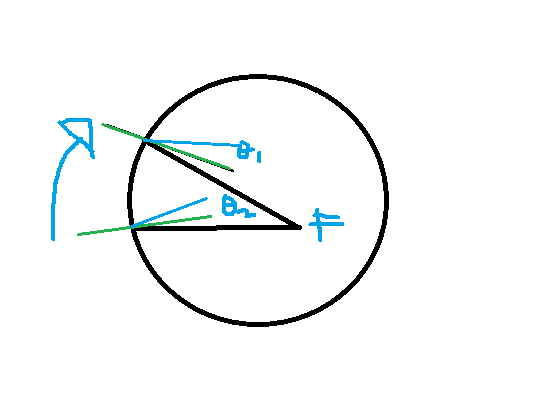
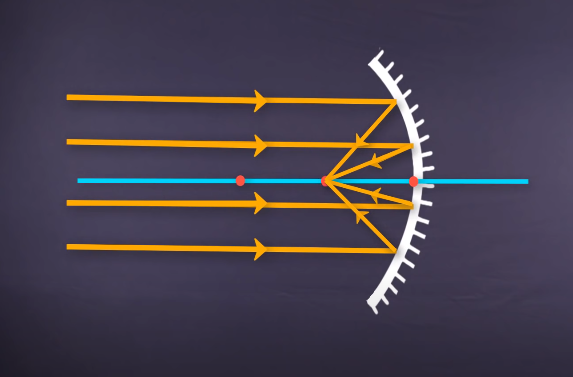
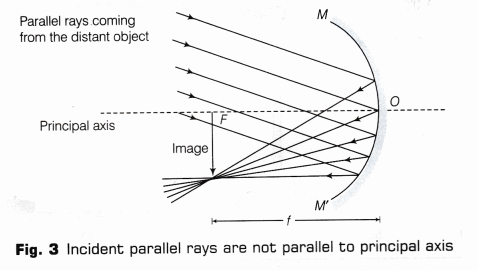
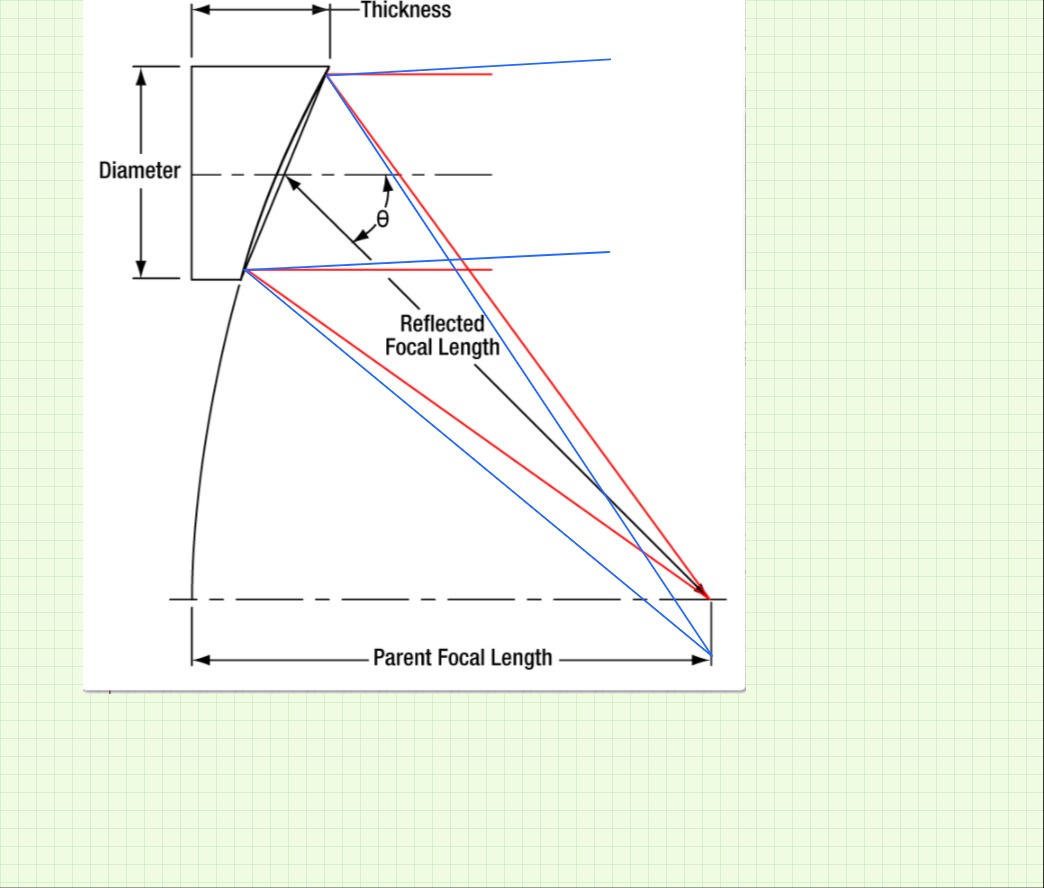
What happens when you change the angle of incidence(normal to the mirror)? How does the angle of reflection change? Is it invariant?
3
-
1$\begingroup$ You could check this yourself for a spherical mirror using a CAD program. Draw the rays of light hitting the mirror and reflect each of them in radial line (from the mirror centre to the point of contact). If you do it for a few parallel rays both on-axis and off-axis you should get your answer. Note that spherical mirrors will approximate a parabola over a small angle (not too deep a dish). $\endgroup$– TransistorCommented Jan 11, 2022 at 13:11
-
$\begingroup$ What are you asking? For a smooth surface, incident angle equals reflected angle based on the normal to the surface at the incidence point. $\endgroup$– Carl WitthoftCommented Jan 11, 2022 at 15:07
-
$\begingroup$ Im asking, from the same point let there be a incident ray from that position onto a concave reflective device. Without moving the point, rotate the incident ray to a different section of the reflective device. What are the relations between travel distance, angle of rotation and the change in incident/reflection angles $\endgroup$– ThreadBucksCommented Jan 12, 2022 at 2:21
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
(this is not much of an answer) I assume you are talking about the case where incident parallel rays are not parallel to the principal axis, i.e.
To my understanding it is not invariant (i.e. the focal point shifts) and it also depends on the shape of the mirror (e.g. parabolic or spherical).
-
$\begingroup$ This illustrate what i was asking quite well. As the incident rays passing through the same focal point begin to rotate, the angle of incidence and reflection appear to decrease from M-?M'. $\endgroup$ Commented Jan 12, 2022 at 2:55
$\begingroup$
$\endgroup$
2
let's take two of the rays with the angle of incidence 5 degrees slanted from normal. .
As shown in the diagram they converge on a point on the focal plane.
- Red lines are parallel light with the principal axis.
- blue lines are 5 degrees off.
-
$\begingroup$ For the red rays, from the point in which two red rays are situated at the bottom right. How does the angle of incidence change as you rotate the red ray counter clockwise? Ofcourse at the point where the incident ray is horizontal and travelling the parent focal length, the angle of incident and reflection are 0. So does the angle of incidence and reflection decrease equal as they reach the horizontal direction? $\endgroup$ Commented Jan 12, 2022 at 2:14
-
$\begingroup$ The red rays at the lower right have converged at the focal point of the mirror. they are not the incidental rays, they are reflected rays. but if you want them to converge at a point higher you would need to project the red rays from a point below horizon. as it is they are coming parallel to the horizon. eventually, if you keep projecting from a source under the horizon when the incidental angle is 90 to the surface reflected ray is 90 too. meaning the ray reflects right back on itself. $\endgroup$– kamranCommented Jan 12, 2022 at 2:54