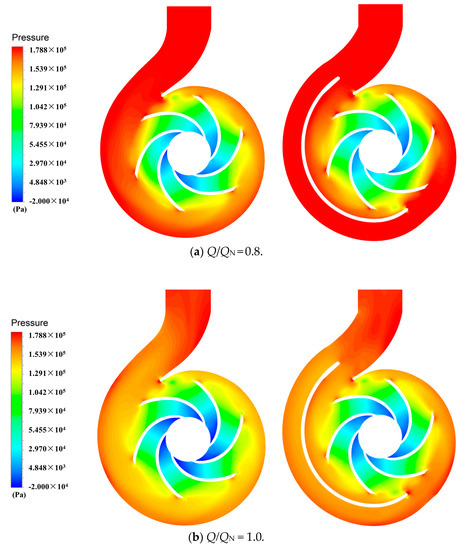
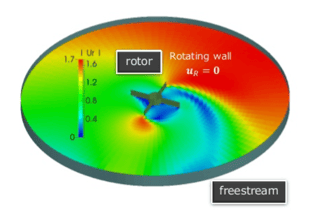
Euler turbomachinery equation doesn't include a term for changes in flow area.
But, an centrifugal impeller (not the volute) has increasing flow area along the path of the flow.
Is the pressure actually constant along the path of the flow, and if not, is there an equation that could predict the change in pressure? 
(It's non trivial to deduce myself since energy is continuously added to the flow as its area expands)
The more complex the geometry, the less likely generalized equations are going to be able to represent it. I understand what you are saying about the area of the volute getting larger as the fluid travels the pathline, but this is inside of a rotating reference frame so you can't compare it to static geometry. Computational Fluid Dynamics (CFD) verified by physical test results is the best way to visualize the pressure distribution for a given geometry and use condition.
Below you can see how the pressure gradually increases as it travels down the volute. This photo was taken from the article "Numerical Investigation on the Mechanism of Double-Volute Balancing Radial Hydraulic Force on the Centrifugal Pump". There are lots of other good examples and papers out there on this topic.
This CFD slideshare by Fumiya Nozaki goes through the basics of setting up your own rotating reference frame test in OpenFOAM.
