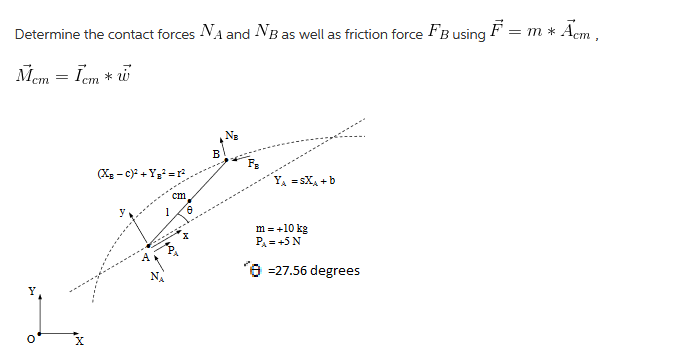
Maybe something like this:
Step 1: By the previous answer, the coordinate $X_A = X_A(t)$ and the angle $\theta = \theta(t)$ are connected by the equation
$$\big(\,X_A + l \cos(\theta + \varphi_0) - c\,\big)^2 \, + \, \big(\,sX_A + l \sin(\theta + \varphi_0) + b\,\big)^2 \, = \, r^2$$
Knowing $X_A$, plug it in the equation and solve for $\theta$.
Step 2: Differentiate the equation from step 1 with respect to $t$ and obtain the equation
$$\big(\,X_A + l \cos(\theta + \varphi_0) - c\,\big)\left(\frac{dX_A}{dt} - l\sin(\theta + \varphi_0)\frac{d\theta}{dt}\right) \, + \, \big(\,sX_A + l \sin(\theta + \varphi_0) + b\,\big)\left(s\frac{dX_A}{dt} + l\cos(\theta + \varphi_0)\frac{d\theta}{dt}\right) = 0$$
Knowing $X_A, \, \theta, \, \frac{dX_A}{dt} = V_A$, plug them in the equation and solve for $\frac{d\theta}{dt}$.
Step 3: Differentiate the equation from step 2 with respect to $t$ and obtain the equation (which you should calcualte):
$$\frac{d}{dt} \left( \, \big(\,X_A + l \cos(\theta + \varphi_0) - c\,\big)\left(\frac{dX_A}{dt} - l\sin(\theta + \varphi_0)\frac{d\theta}{dt}\right) \, + \, \big(\,sX_A + l \sin(\theta + \varphi_0) + b\,\big)\left(s\frac{dX_A}{dt} + l\cos(\theta + \varphi_0)\frac{d\theta}{dt}\right) \, \right) = 0$$ for $\frac{d\theta}{dt}$.
This will be an equation for $X_A, \theta, V_A = \frac{dX_A}{dt}, \, \frac{d\theta}{dt}, \, A_A = \frac{d^2X_A}{dt^2}$ and $\frac{d^2\theta}{dt^2}$. Given $X_A, \theta, V_A = \frac{dX_A}{dt}, \, \frac{d\theta}{dt}, \, A_A = \frac{d^2X_A}{dt^2}$, plug them in the equation and solve for $\frac{d^2\theta}{dt^2}$.
Step 4: Calculate the coordinates of the point $B$ on the circle:
\begin{align}
&X_B = X_A + l\cos(\theta + \varphi_0) \\
&Y_B = sX_A + l\sin(\theta + \varphi_0) + b
\end{align}
Step 5: Calculate the coordinates of the unit vectors $\vec{n}_A$ and $\vec{n}_B$ at points $A$ and $B$, perpendicular to the line and the circle respectively. For $\vec{n}_A$:
\begin{align}
&n_{x,A} = \frac{-s}{\sqrt{1+s^2}} \\
&n_{y,A} = \frac{1}{\sqrt{1+s^2}}
\end{align}
and for $\vec{n}_B$:
\begin{align}
&n_{x,B} = \frac{X_B - c}{\sqrt{(X_B - c)^2 + Y_B^2}} = \frac{X_B - c}{r}\\
&n_{y,B} = \frac{Y_B}{\sqrt{(X_B - c)^2 + Y_B^2}} = \frac{Y_B}{r}
\end{align}
Step 6: Calculate the coordinates of the unit vectors $\vec{t}_A$ and $\vec{t}_B$ at points $A$ and $B$, tangent to the line and the circle respectively. For $\vec{t}_A$:
\begin{align}
&t_{x,A} = \frac{1}{\sqrt{1+s^2}} \\
&t_{y,A} = \frac{s}{\sqrt{1+s^2}}
\end{align}
and for $\vec{t}_B$:
\begin{align}
&t_{x,B} = \frac{Y_B}{\sqrt{(X_B - c)^2 + Y_B^2}} = \frac{Y_B}{r}\\
&t_{y,B} = \frac{c - X_B}{\sqrt{(X_B - c)^2 + Y_B^2}} = \frac{c - X_B}{r}
\end{align}
Step 7: Calculate the acceleration of point $B$, by differentiating twice with respect to time $t$ the equation for the coordinates $X_B, \, Y_B$:
\begin{align}
&A_{x,B} = \frac{d^2X_A}{dt^2} = A_A - l\sin(\theta + \varphi_0)\frac{d^2\theta}{dt^2} - l\cos(\theta + \varphi_0)\left(\frac{d\theta}{dt}\right)^2\\
&A_{y,B} = \frac{d^2Y_A}{dt^2} =s\,A_A + l\cos(\theta + \varphi_0)\frac{d^2\theta}{dt^2} - l\sin(\theta + \varphi_0)\left(\frac{d\theta}{dt}\right)^2
\end{align}
Since, up to know, you are either given or have calculated the variables $A_A, \, \theta, \, \frac{d\theta}{dt}, \, \frac{d^2\theta}{dt^2}$, you can plug them in the equations and calculate the coordinates $A_{x,B}, \, A_{y,B}$ of the acceleration vector $\vec{A}_B$ of point $B$. The coordinates of the acceleration vector $\vec{A}_A$ of point $A$ are $A_{A}, \, sA_{A}$.
Step 8: Finally put together the linear system of three scalar equations and three unknown force magnitudes $N_A, \, N_B, \, F_B$:
\begin{align}
& \left(\frac{ml^2}{12}\right) \, \frac{d^2\theta }{dt^2} \, \hat{k} \, = \, \frac{1}{2} \, \big(l \, \cos(\theta + \varphi_0)\hat{i} + l \, \sin(\theta + \varphi_0)\hat{j}\big) \times \left(\,F_B \vec{t}_B + N_B\vec{n}_B - P_A \vec{t}_A - N_A \vec{n}_A\,\right)\\
&\frac{m}{2} \big(\vec{A}_A + \vec{A}_B\big) = F_B \vec{t}_B + N_B\vec{n}_B + P_A \vec{t}_A + N_A \vec{n}_A
\end{align} and since you already know all the other parameters in this system, including $P_A$ which is given, solve for $N_A, \, N_B, \, F_B$. Here $\hat{i}, \, \hat{j}, \, \hat{k}$ are the pairwise orthogonal unit vectors of the inertial coordinate system of the system. The vector $\hat{k}$ is perpendicular to the 2D picture.