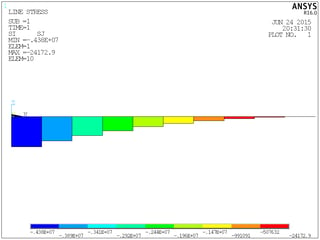
I'm doing a static analysis on a uniformly loaded cantilever beam in ANSYS APDL. I'm using BEAM188 with all the default options, so it uses linear form. for the element behaviour, which I think means the bending moments and tensions are linear within the element.
I want to plot the bending moments along the beam with an area contour, for that I'm using:
- ETABLE,MI,SMISC,3
- ETABLE,MJ,SMISC,16
- PLLS,MI,MJ,1,0
This are the axial stresses due to bending, it looks very similar to the bending moment, it shows discontinuities between elements and for each element it shows the same value on both nodes.
Why does MI=MJ? Shouldn't there be a linear variation of the moment in the elements? Does it make sense for it to be discontinuous between elements? Or is this just a bad plot and I should plot the values on the nodes I get with PRESOL,M as opposed the plot each I and J node values for each element.
The results overall seem good, but I'm not sure if this is a correct representation of the behaviour of the elements.
I'm sorry for such a basic question. I hope I wasn't too confusing.