Lift produced by an airplane wing is related to airspeed - this much is clear; a plane moving too slowly will stall. But what is that relation? Linear? Quadratic? Exponential? I don't need the exact equation, which is surely quite complex, just the character of the relation.
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ The fact that the lift equation is actually not that complex at all is one of the things I liked most about studying aerodynamics. It's when you look into that "lift coefficient" and how to find it that things get weird... $\endgroup$– anaximanderCommented Jun 3, 2015 at 12:58
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
Quadratically.
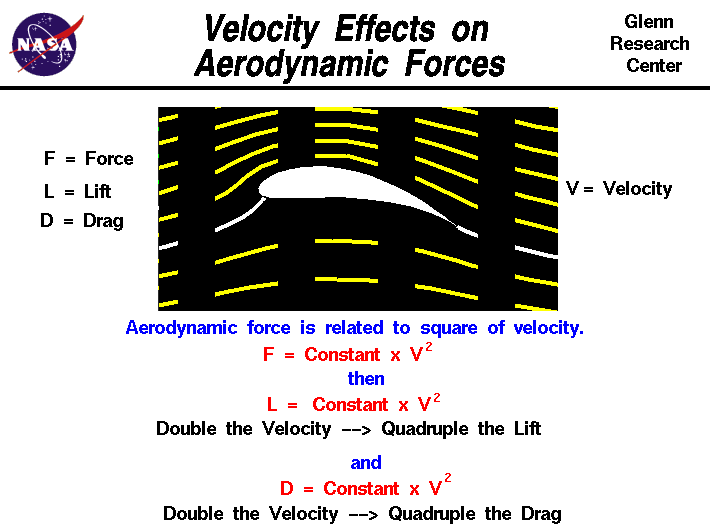
NASA page #1 says that, as an approximation, $$\text{Lift}\propto v^2$$ where $v$ is the airspeed.
NASA page #2 says that, for a better calculation, $$\text{Lift}=av^2+bv+c$$ where $a$, $b$, and $c$ are constants. This is odd because it implies that lift is not merely proportional to $v^2$, because there is the $bv$ term in there, as well as the $c$ term.
But there is a better equation, given here (and here), among other places: $$\text{Lift}=\frac{1}{2}\rho v^2 \times \text{lift coefficient} \times \text{area}$$ where $\rho$ is air density.
This is similar to the drag formula.
$\begingroup$
$\endgroup$
As has already been mentioned, the primary relationship is that lift goes with the square of the airspeed.
To give you some intuition as to why this is, consider what a wing does. As it moves along, it deflects air downwards. Lift is the upwards force from imparting downward momentum on the air the wing passes thru.
Momentum is mass times velocity, and force is momentum per time. As the airspeed increases, both more air per unit time is pushed downward, and it is pushed downward faster. Put another way, the force is (mass / time)(velocity), with both mass/time and velocity roughly proportional to the airspeed, so the force is proportional to the square of the airspeed.
answered Jun 2, 2015 at 18:54
$\begingroup$
$\endgroup$
2
In addition to the basic equation ($L = CL * 0.5 * \rho * V^2 * \text{Ref Area}$), the coefficient of lift varies with speed if the speed range of concern is large enough, e.g. the Reynolds number changes considerably (maybe 2-3 times). The Reynolds number affects $CL$, especially for higher $CL$ values.
-
$\begingroup$ And how would it it change for extreme velocities (and low medium density)? In particular I'm interested in what would happen to the lift of a space plane on reentry from LEO; instead of diving into dense atmosphere, exposing itself to extreme reentry heat, would it be capable of gliding over the upper reaches of the atmosphere letting its drag slow it down gradually and only "sinking" as much as it loses lift with speed (and restoring it as the atmosphere gets dense enough to provide more lift) $\endgroup$– SF.Commented Jun 3, 2015 at 7:03
-
$\begingroup$ Oh, this is a very unusual topic. I think this deserves a question on its own, probably it will collect more interest too. $\endgroup$ Commented Jun 3, 2015 at 16:15
$\begingroup$
$\endgroup$
It's all based in air pressure. Lifting requires velocity because it needs to create pressure gradients between the wing profile, so the air that is lower in pressure in the bottom part of the wing tend to equalize with the pressure in the upper part. When you stall, that means that you are no longer creating pressure between the upper and lower part of the wing profile.
That's why you have different wing profile geometries, so that you can make different pressure ranges in different velocity scales.
answered Jun 3, 2015 at 14:17
$\begingroup$
$\endgroup$
Lift is proportional to the square of velocity.
