0
$\begingroup$

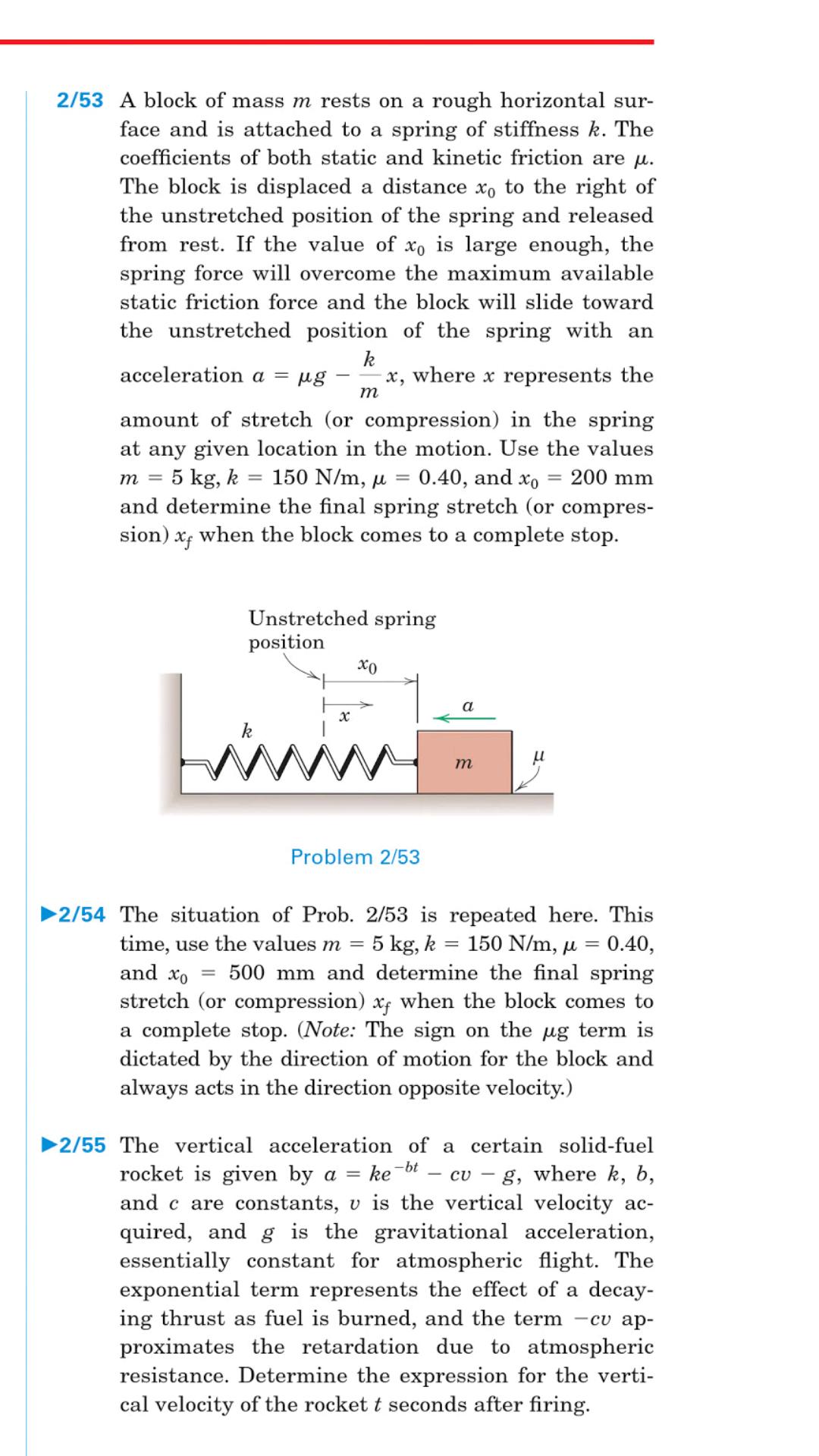
enter image description here

So I'm a hobbiest who loves doing and learning about different subjects. Often I run into difficult problems that I normally can find solutions to however this one stumped me, I have reviewed many physics journals on the problem but either they are two complex or tackle the much easier version of 'viscous' friction.(p 2/54) Essentially when I even begin a solution I yield to this:

Ma = sign(v) * mg*mu - kx

This would be simple if the friction didn't change direction. Does anyone have a suggestion on how to approach this problem? I'm not looking for someone to solve it I just want an idea on how to start it. Thank you

Improve this question
$\endgroup$
4
  • $\begingroup$ You may get better luck on the physics page, but I'll give a good shot below. $\endgroup$
    – Mark
    Commented Jun 25, 2018 at 16:55
  • $\begingroup$ @Mark, Thank you I would like to see how someone else approaches this problem. I posted it on the physics site as well so we'll see $\endgroup$
    – chris360
    Commented Jun 25, 2018 at 17:10
  • $\begingroup$ @chris360 please do not double-post. If you think, correctly, that this is a Physics.SE question, then please delete it here. $\endgroup$
    – Carl Witthoft
    Commented Jun 25, 2018 at 18:18
  • $\begingroup$ @CarlWitthoft - that seems to contradict with the Meta in Physics - physics.meta.stackexchange.com/questions/7525/… $\endgroup$
    – Mark
    Commented Jun 25, 2018 at 18:38

2 Answers 2

Reset to default
1
$\begingroup$

The sign change problem can be avoided slightly - by substituting a different equation for sign(v).

$$ma = mg\mu*sign(v) - kx$$ $$ma = mg\mu\lim_{n\to\infty}tanh(nv)-kx$$

Ignoring the limit for now, we have a real differential equation! Since we don't depend on t explicitly, we'll make an immediate autonomous substitution. $v(x) = v$. Then, $a(t) = v*\frac{dv}{dx} = v*v'$

$$vmv' = mg\mu\lim_{n\to\infty}{tanh(nv)}-{kx}$$

As a first order equation, this is easier, but not by much.

Improve this answer
$\endgroup$
3
  • $\begingroup$ If you take the limit as n goes to infinity of hyperbolic tangent you get π/2, so to counter it (to get 1) would I just place a 2/π in front of it? $\endgroup$
    – chris360
    Commented Jun 25, 2018 at 18:55
  • $\begingroup$ Yes, that’s correct $\endgroup$
    – Mark
    Commented Jun 25, 2018 at 18:56
  • $\begingroup$ I'm not sure I know how to solve the DEQ but I'm gunna try! $\endgroup$
    – chris360
    Commented Jun 25, 2018 at 18:57
1
$\begingroup$

Method 1

This would be simple if the friction didn't change direction

Indeed. So here is one way to deal with it. First, determine the initial velocity at t=0. E.g. let's just say v(0) is positive. Then instead of

$ma =sign(v) * mg*\mu - kx$

Just write

$ma =mg*\mu - kx$

Now, solve that equation, which should be pretty easy, and plot velocity versus time. You'll note that at some point, the velocity is no longer positive, and has changed to negative. Let's just call that time $t_1$. i.e. $v(t_1)=0$. So at that point, "stop" that solution, and write a new equation

$ma =-mg*\mu - kx$

this equation "starts" at time $t=t_1$ with initial conditions $v(t_1)=0$ and $x(t_1)$ determined from the ending position of the first solution. This equation should also be easy to solve. Continue the process, switching back and forth as necessary. At each point, you will also need to check if the spring force is enough to overcome the static friction. At some point it will not be, and then you stop. At the end, stitch together all of the partial solutions to each segment into one big piecewise solution.

Method 2

Use numerical integration. E.g. apply Euler's method for solving differential equations. Over each time step, you can assume that the friction force acts in a constant direction. Assuming the time steps are small enough, you'll get about the same answer as above.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.