2
$\begingroup$


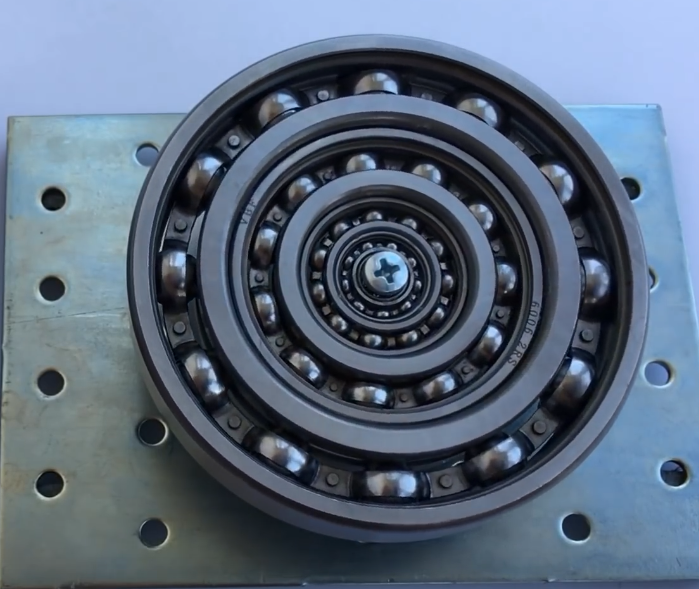
I have a few questions relating to a multiple layered spinner that uses ball bearings to reduce friction, kind of similar to a fidget spinner. The image below is an example of the multilayered bearing spinner.

Bearing Spinner

My questions are as follows:

  1. Does having multiple layer of bearing rings decrease the overall friction on the outermost ring and allow it to spin for a longer period of time?
  2. Does increasing the number of ball bearings in each ring decrease friction?
  3. Are there any formulas or relation that would allow me to calculate the overall run time of the outermost ring for a given tangential force?
Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

The more you increase the number of balls and tracks increases the friction, what does change is the speed differential between the any track and its predecessor / successor - but guaranteeing that the middle tracks will share the changes of speed....

If you want a suggestion for a long life spinner : use a spinner that is supported in a magnetic field then it is basically only air friction and, if you put it in a vacuum not even that.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for the answer, Solar Mike. However, if I decrease the number of bearing balls, say 4 placed at diametrically opposite ends, the total friction would be reduced as the contact point is much lesser. But does the lower number of bearing balls affect the spinning motion, say I'm just using 3 balls, placed in a triangular formation. $\endgroup$
    – Vijey
    Commented Jan 10, 2018 at 9:02
  • $\begingroup$ What do you think will happen to the stability? Will the races start to wobble and then slow down? Why don’t you run some tests and find out - you have already built the first version. $\endgroup$
    – Solar Mike
    Commented Jan 10, 2018 at 9:05
0
$\begingroup$

If you reduce the number of layers of bearings to just one large diameter, and try to add all their mass to exterior layer, you almost double the angular moment of inertia of the system, which is going to be more efficient in conserving the momentum, but also will save on the leak and waste of energy due to wobbling of intermediary layers and friction due to out of plane vibration of those layers and fluttering of them.

I better alternative would be just one thin disk or even some radial spokes leading to the ball bearing with the total mas of your system.

lets compare two cases, first one you have many many layers of rings, ilmost like a solid disk, and second one with just one large disk same size as the first case and mass concentrated on the perimeter.

$$ I_{many\ rings} = \frac{1}{2} MR^2 $$ $$ I_{one \ ring} = MR^2 $$ M= mass , R = radius

As we see I of one ring is twice as much as I of many rings. And also we don't have all the extra friction of many rings.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.