What is the bending moment formula for continuously supported beam of equal span?

Question

Is there a standard chart in Eurocode for the maximum and minimum bending moment in uniformly loaded beam for indeterminate structures where we can use directly? Many textbooks give different coefficient values for the moment for continuous beam, some wl2/10, some 0.046wl2. I know that hogging and sagging moment are different. Is there an authoritative reference for this?

Answer 1

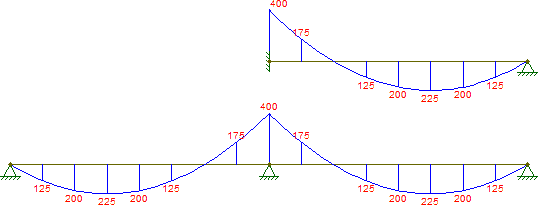
A continuous beam with two spans with uniformly distributed load looks like this:

This is symmetric, and we know by inspection that the rotation of the beam at the central support will be null. So let's make our lives a bit easier and only look at the right half of the beam, considering the central support as fixed. Obviously, this is only possible because the beam and its loading is symmetric.

This beam is still statically indeterminate, however. But let's get rid of the rightmost support for now:

This is an isostatic structure we can trivially solve. However, the deflection at the extremity will obviously be non-null, which isn't correct for our true condition. Let's calculate what that deflection would be, using the fundamental beam equation:

$$\frac{\text{d}^2}{\text{d}x^2}\left(EI\frac{\text{d}^2w}{\text{d}x^2}\right) = q$$ which tells us that the fourth derivative of the deflection is equal to the applied load.

So, integrating the applied load we get the shear force:

$$\begin{gather} Q = \int\limits_0^L q\text{d}x = qx + C_1 \\ Q(0) = C_1 = -qL \\ \therefore Q = qx - qL \end{gather}$$

Integrating the shear force we get the bending moment:

$$\begin{gather} M = \int\limits_0^L (qx - qL)\text{d}x = \frac{qx^2}{2} - qLx + C_2 \\ M(0) = C_2 = \frac{qL^2}{2} \\ \therefore M = \frac{qx^2}{2} - qLx + \frac{qL^2}{2} \end{gather}$$

Integrating the bending moment we get the curvature times the stiffness:

$$\begin{gather} EI\theta = \int\limits_0^L \left(\frac{qx^2}{2} - qLx + \frac{qL^2}{2}\right)\text{d}x = \frac{qx^3}{6} - \frac{qLx^2}{2} + \frac{qL^2x}{2} + C_3 \\ \theta(0) = C_3 = 0 \\ \therefore EI\theta = \frac{qx^3}{6} - \frac{qLx^2}{2} + \frac{qL^2x}{2} \end{gather}$$

Integrating the curvature we get the deflection:

$$\begin{gather} EI\delta = \int\limits_0^L \left(\frac{qx^3}{6} - \frac{qLx^2}{2} + \frac{qL^2x}{2}\right)\text{d}x = \frac{qx^4}{24} - \frac{qLx^3}{6} + \frac{qL^2x^2}{4} + C_4 \\ \delta(0) = C_4 = 0 \\ \therefore EI\delta = \frac{qx^4}{24} - \frac{qLx^3}{6} + \frac{qL^2x^2}{4} \end{gather}$$

Therefore, at the end of the cantilever the deflection is

$$\begin{align} EI\delta(L) &= \frac{qL^4}{24} - \frac{qL^4}{6} + \frac{qL^4}{4} \\ \delta(L) &= \frac{qL^4}{8EI} \end{align}$$

So, in order to regain compatibility with our true condition, we need to bring that deflection down to zero. That's done by the vertical reaction force of the rightmost support. So let's replace that support with a concentrated vertical force and calculate the deflection caused by that force:

$$\begin{gather} Q = \int\limits_0^L 0\text{d}x = C_1 \\ Q(0) = C_1 = -P \\ \therefore Q = -P \\ M = \int\limits_0^L -P\text{d}x = -Px + C_2 \\ M(0) = C_2 = PL \\ \therefore M = -Px + PL \\ \theta = \int\limits_0^L (-Px + PL)\text{d}x = \frac{-Px^2}{2} + PLx + C_3 \\ \theta(0) = C_3 = 0 \\ \therefore EI\theta = \frac{-Px^2}{2} + PLx \\ \delta = \int\limits_0^L \left(\frac{-Px^2}{2} + PLx\right)\text{d}x = \frac{-Px^3}{6} + \frac{PLx^2}{2} + C_4 \\ \delta(0) = C_4 = 0 \\ \therefore EI\delta = \frac{-Px^3}{6} + \frac{PLx^2}{2} \\ \delta(L) = \frac{PL^3}{3EI} \end{gather}$$

So now, we can calculate the necessary force to counteract the deflection at the rightmost extremity:

$$\begin{gather} \frac{PL^3}{3EI} = -\frac{qL^4}{8EI} \\ \therefore P = -\frac{3qL}{8} \end{gather}$$

So, looking at the component equations for the bending moment from the uniform load and the reaction force, we can add them together to get the final bending moment equation for the span:

$$\begin{align} M &= \frac{qx^2}{2} - qLx + \frac{qL^2}{2} - Px + PL \\ M &= \frac{qx^2}{2} - qLx + \frac{qL^2}{2} - \left(\frac{-3qL}{8}\right)x + \left(\frac{-3qL}{8}\right)L \\ M &= \frac{qx^2}{2} - \left(qL - \frac{3qL}{8}\right)x - \frac{3qL^2}{8} + \frac{qL^2}{2} \\ M &= q\left(\frac{x^2}{2} - \frac{5Lx}{8} + \frac{L^2}{8}\right) \end{align}$$

The minimum moment is found at the central support and is equal to

$$M(0) = \frac{qL^2}{8}$$

The maximum moment is trickier, since it isn't necessarily at midspan. First we need to calculate the moment's derivative zero to find its position:

$$\begin{align} M' &= q\left(x - \frac{5L}{8}\right) = 0 \\ \therefore x &= \frac{5L}{8} \\ \therefore M_{max} &= M\left(\frac{5L}{8}\right) \\ &= q\left(\frac{\left(\dfrac{5L}{8}\right)^2}{2} - \frac{5L\left(\dfrac{5L}{8}\right)}{8} + \frac{L^2}{8}\right) \\ &= -\frac{9qL^2}{128} \approx -\frac{qL^2}{14.2}\\ \end{align}$$

That approximation is a classic numerical shortcut for the maximum moment on a fixed-and-pinned beam. And obviously all my results assume that $q > 0$ points upwards. So all the loads in the beams at the start of my answer have $q = -1\text{ kN/m}$. If you prefer to think of such loads as positive, just flip the sign in my results.

And then, to confirm our results, here is a beam with $L=5\text{ m}$ (per span) and $q=128\text{ kN/m}$. The equations give us

$$\begin{align} M_{min} &= \frac{qL^2}{8} = -400\text{ kNm}\\ M_{max} &= -\frac{9qL^2}{128} = 225\text{ kNm}\\ \end{align}$$

Answer 2

I would suggest Timoshenko, Strength of Materials is an authoritative reference (used in the UK ...) : This is found on the www, and also, copies can be found in second-hand bookshops but even then they are not cheap...

Link removed to avoid "link rot"...