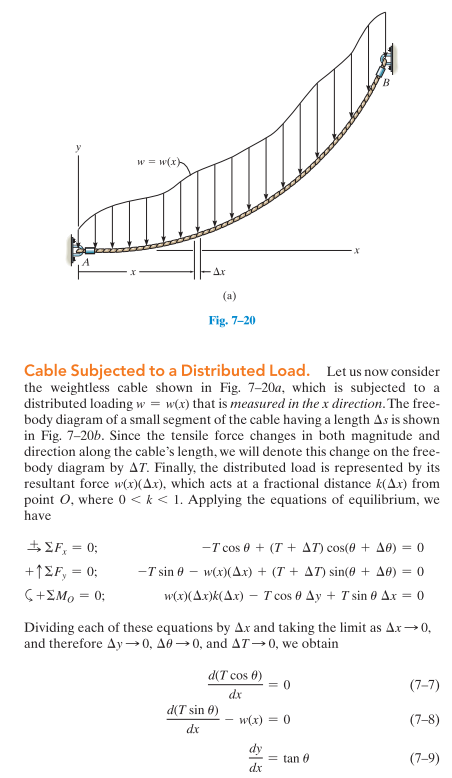
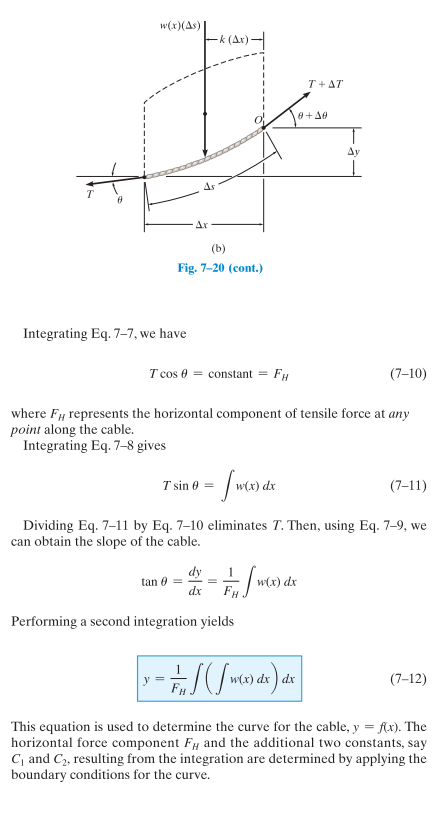
I am looking at Hibbeler's section on a `Cable Subjected to a Distributed Load' in Engineering Mechanics - Statics, Ed13, as shown in the attached images.
I am getting stuck deriving equation (7-7). I derive the horizontal equilibrium equation just fine;
$$ |\vec{T} + \vec{\Delta T}| \cdot \cos(\theta + \Delta \theta) - |\vec{T}|\cdot \cos(\theta) = 0 $$
I rearrange to describe horizontal tension component as a function of $\Delta x$ (given $\Delta T$ and $\Delta \theta$ are ultimately functions of $\Delta x$);
$$ |\vec{T}|\cdot \cos(\theta) = |\vec{T} + \vec{\Delta T}|\cdot \cos(\theta + \Delta \theta) $$
Then I express the differential;
$$ \frac{\Delta(|\vec{T}|\cdot \cos(\theta))}{\Delta x} = \frac{|\vec{T} + \vec{\Delta T}|\cdot \cos(\theta + \Delta \theta)}{\Delta x} $$
Then when I go to express the derivative, I come up with a limit which does not exist.
$$ \frac{d(|\vec{T}|\cdot \cos(\theta))}{dx} = \lim_{\Delta x \to 0}\frac{|\vec{T} + \vec{\Delta T}|\cdot \cos(\theta + \Delta \theta)}{\Delta x} $$
Further to this, it is not obvious to me how the resultant force from the distributed load acting over $\Delta x$ can be defined as $w(x)\Delta x$ (in line 8) as the author suggests. I feel it should be defined as;
$$ \Delta F = \int_{x}^{x+\Delta x}w(x)dx $$
becuase the loading intensity function is not constant. Any pointers as to where my errors are? Appreciate any advice offered. Thanks.