2
$\begingroup$

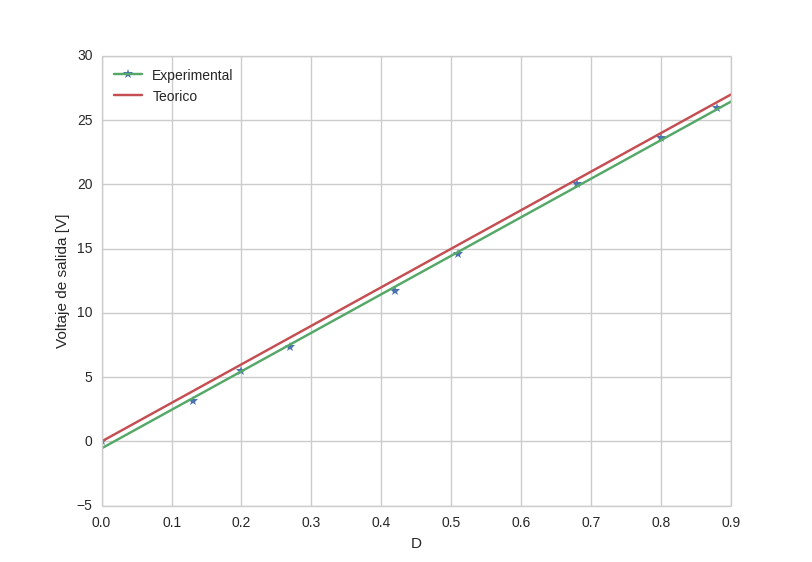
I made a DC-DC converter its ideal transfer function is: $$ V_0(D) = DV_i $$

I took measurements of its response and then made a linear regression, I got this result:

$$ V_0(D) = 0.98DV_i -0.42 $$

Quite similar to the expected value. Now, can I say that the system has a linear response?

strictly speaking, the system response should be zero when the input is zero, but in this case, if D = 0:

$$ V_0 = -0.42 $$

What would be the correct way to refer at the response of this system?

Edit:

Here is a plot of the theoric response, red, vs experimental values green. The 0.42 basically represents an offset because the losses of the system.

enter image description here

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ I believe the term is affine $\endgroup$
    – Nayuki
    Commented Jun 5, 2016 at 1:36
  • $\begingroup$ Well it seems you have an awful small amount of data (only 8 points). It's hard to come to a conclusion about your system's response with so few observation points. $\endgroup$
    – Lucas Franceschi
    Commented Jun 5, 2016 at 1:48
  • 1
    $\begingroup$ I would say, it is linear in static states, but the behavior might be non-linear under dynamic conditions. $\endgroup$
    – MaestroGlanz
    Commented Jun 6, 2016 at 8:11
  • 1
    $\begingroup$ See also this question in relation to Nayuki's comment. $\endgroup$
    – Karlo
    Commented Jun 8, 2016 at 15:47
  • $\begingroup$ Probably your system have a bias. The switching maybe... $\endgroup$
    – leCrazyEngineer
    Commented Jul 17, 2016 at 1:22

1 Answer 1

Reset to default
1
$\begingroup$

Well, I wouldn't say the result is quite similar, I don't have any information on scale or units of measurement but the $-0.42$ value might be significant. You should analyze other data, such as the correlation factor, and decide if the linear model you use to regress the data is appropriate.

To answer your question: no. I think that, based only on what you have presented, you cannot conclude your system has a linear response. You should try to fit other models to prove they don't fit as well as a linear one and also present some more data. That doesn't mean it's not linear though only that you need to do some more analysis before concluding that.

EDIT: By the way, if a system does have a $Y(x) = ax + c$ response it is by definition linear, I think your question is whether your system does have such a response.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I update the question, you're right the 0.42 was out of context. $\endgroup$
    – Luis Ramon Ramirez Rodriguez
    Commented Jun 5, 2016 at 1:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.