I am trying to derive the maximum beam slope formula $\theta = \dfrac{wL^3}{24EI}$ for this cantilever beam:
What method can I use for this derivation? So far I can only find simply supported beams and derivations for the $\theta_{max}$ formula at the free end (e.g., "slope at free end" column of this table). I tried doing it by hand, but I cannot seem to get to the correct answer.
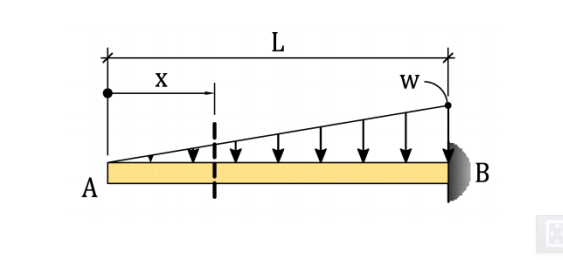
The way to derive the deflection is via the relation $w = EI\dfrac{d^4\delta}{d^4x}$, which means that the deflection is the fourth integral of the loading (and rotation can be taken as the derivative of the deflection and therefore equal to the third integral of the loading).
So, let's begin with the loading: $$w = w_{max}\dfrac{x}{L}$$
The first integral of the load is the shear force: $$Q = \int w = w_{max}\dfrac{x^2}{2L} + C_1$$ we know that in this case $C_1 = Q(0) = 0$ since it is the free end of the beam.
The second integral of the load is the bending moment: $$M = \int Q = w_{max}\dfrac{x^3}{6L} + C_2$$ we know that in this case $C_2 = M(0) = 0$ as well since it is the free end of the beam.
The third integral of the load is the beam's rotation (times its stiffness): $$\theta = \dfrac{1}{EI}\int M = \dfrac{1}{EI}\left(w_{max}\dfrac{x^4}{24L} + C_3\right)$$ we know that in this case $\theta(L) = 0$ since it is the fixed end of the beam, therefore \begin{align} \theta(L) = 0 &= w_{max}\dfrac{L^3}{24} + C_3 \\ \therefore C_3 &= -w_{max}\dfrac{L^3}{24} \\ \therefore \theta &= \dfrac{w_{max}}{EI}\left(\dfrac{x^4}{24L} - \dfrac{L^3}{24}\right) \\ \therefore \theta(0) &= -\dfrac{w_{max}L^3}{24EI} \end{align}
