1
$\begingroup$

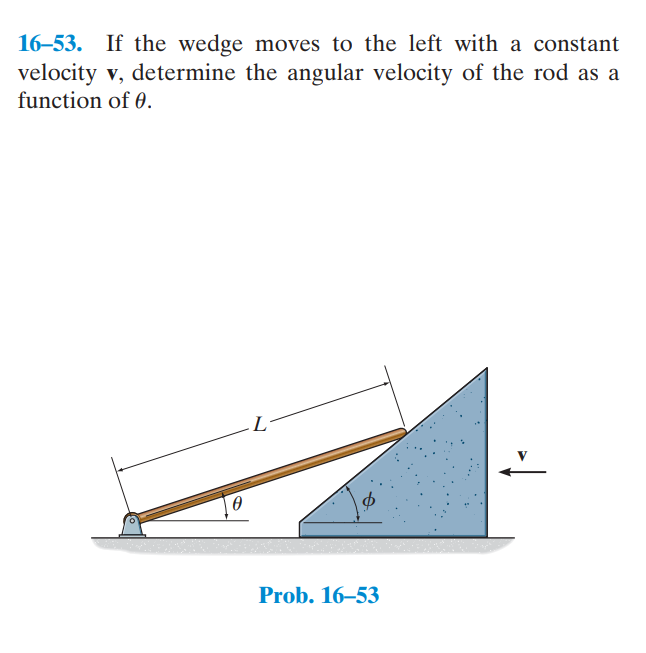
I am seeking help for a textbook question that I am stuck on. enter image description here

My work is attached below. I broke the velocity vector into two components, one that is perpendicular to the wedge, causing the rod to rotate, and the other is the force acting along the surface of the wedge. I am unsure if this is the correct approach, and my answer is in terms of φ, not θ, and I can't find the relationship between the two. enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Use "instantaneous center" method $\endgroup$
    – Tony Stewart EE75
    Commented Sep 16, 2022 at 6:01
  • 1
    $\begingroup$ Your attempt is useless. Make an expression for cos(θ) in a rectangular triangle as a function of L, θ, the angle of the wedge and the remaining horizontal distance to the rod joint. Differentiate it. Solve the derivative of θ which is the wanted angular velocity. Differentation rule knowledge for trigonometric and nested functions is a must. $\endgroup$
    – user33233
    Commented Sep 16, 2022 at 15:52
  • 1
    $\begingroup$ (continued) Seemingly the case is already solved, but the said cos(θ) is shown in the next image: i.sstatic.net/U1EQP.png $\endgroup$
    – user33233
    Commented Sep 16, 2022 at 23:34
  • $\begingroup$ How did you figure out that the remaining length on the wedge is Lsin(theta)/tan(phi)? $\endgroup$
    – Sherwood
    Commented Sep 18, 2022 at 16:11
  • $\begingroup$ There's used 2 rectangular triangles. At first one gets from L and theta the vertical side length L sin(theta). In the smaller triangle tan(phi) is by tangent definition the calculated vertical side Lsin(theta)/ the length of the wedge bottom portion below the rod. $\endgroup$
    – user33233
    Commented Sep 18, 2022 at 18:50

2 Answers 2

Reset to default
2
$\begingroup$

There are many ways to solve this problem. Probably the easiest is to use absolute coordinates.

e.g. using the following points:

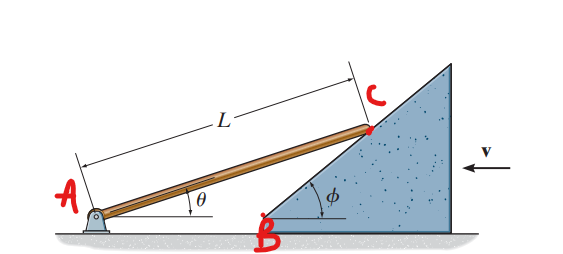
enter image description here

estimate the length between points AB and the length L. this can be done with the law of sines:

$$ \frac{x_{BA}}{\sin(\phi-\theta)} =\frac{L}{\sin (180^o -\phi)} $$

This can be written as:

$$ x_{BA} =\frac{L}{\sin (180^o -\phi)} \sin(\phi-\theta) $$

you can find the velocity by differntiating:

$$ \dot {x}_{BA} = - \frac{L}{\sin (180^o -\phi)} \cos(\phi-\theta) \dot{\theta} $$

However, because point B is moving with the wedge and point A is fixed:

$$ \dot {x}_{BA} = v \rightarrow v = - \frac{L \cos(\phi-\theta) }{\sin (180^o -\phi)} \dot{\theta} $$

The angular velocity for AC $\omega_{AC}$ is $\dot{\theta}$, therefore: $$\omega_{AC} = \dot{\theta} = - \frac{\sin (180^o -\phi)}{L \cos(\phi-{\color{red}\theta}) } v $$

The negative sign, means that for negative velocity (point to the left) the angular velocity is positive.

Improve this answer
$\endgroup$
0
$\begingroup$

enter image description here

Let the pivot be the point $(0,0)$ , and let's show the coordinates of the other end of the rod with $x$ and $y$. Note that $$x=Lcos\theta \quad,\quad y=Lsin\theta$$ and therefore $$dx=-Lsin\theta d\theta \quad,\quad dy=Lcos\theta d\theta \quad (1)$$
In time $dt$ , the rod and the wedge move as shown above. Solid lines and dashed lines correspond to beginning and end of this time interval, respectively. Suppose the sliding end of the rod moves in this time interval from $(x_1,y_1)$ to $(x_2,y_2)$ . The difference between the $x$ coordinates of these points is $dx$ , and the difference between their $y$ coordinates is $dy$ .

The horizontal displacement of the wedge in time $dt$ is $vdt$. Let the oblique edge of the wedge before and after this displacement be represented by $L_1$ and $L_2$, respectively. The equations of these two lines are: $$L_1 : \quad x = y cot \phi + b $$ $$L_2 : \quad x = y cot \phi + b + vdt $$ Note that $(x_1,y_1)$ is on $L_1$ and $(x_2,y_2)$ is on $L_2$. So: $$b = x_1 - y_1 cot \phi $$ $$x_2 = y_2 cot \phi + (x_1 - y_1 cot \phi) + vdt $$ By rearranging and noting that $x_2 - x_1 = dx$ and $y_2 - y_1 = dy$ we have: $$dx - cot \phi dy = vdt $$ And by substitution from (1) we have: $$-L(sin\theta+cot\phi cos\theta)d\theta = vdt $$ Or: $$\frac{d\theta}{dt} = \frac{-v}{L(sin\theta+cot\phi cos\theta)}$$ $$= \frac{-vsin\phi}{Lcos(\theta -\phi)} $$ Finally, note that in this particular example, where the wedge is moving towards left, $v$ is negative. Therefore $\frac{d\theta}{dt}$ is positive.

Improve this answer
$\endgroup$
5
  • $\begingroup$ @user287001 The answer already specifies that $Lcos\theta$ is the horizontal image of the rod. This length is not a function of $v$. It only depends on $\theta$. $\endgroup$
    – Saeed
    Commented Sep 16, 2022 at 22:50
  • $\begingroup$ @user287001 Thank you for the corrective remark. $\endgroup$
    – Saeed
    Commented Sep 17, 2022 at 5:42
  • $\begingroup$ needs still fixes. Check the sign. The angle of the rod should grow. In sentence And by substitution from (1) we have: $$-L(cos\theta+cot\phi cos\theta)d\theta = vdt $$ dx has got cos instead of sin. $\endgroup$
    – user33233
    Commented Sep 17, 2022 at 6:36
  • $\begingroup$ @user287001 Good eye! I had mistyped dx in that formula and the next. As for the sign, an explanation was added in the end: $v$ is negative in this example. $\endgroup$
    – Saeed
    Commented Sep 17, 2022 at 13:35
  • 1
    $\begingroup$ Now it's OK. You get the same without infinitesimal movements by differentiating the formula of cos(θ) shown in my comment below the question. Only write out dθ/dt from the differentiated formula. $\endgroup$
    – user33233
    Commented Sep 17, 2022 at 13:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.