Resultant hydrostatic force on a gate for a fluid that doesn’t reach its center of gravity

Question

Forgive me for these drawings, I can't think of a better way to illustrate the problem right now.

When looking for the resultant hydrostatic force on a flat surface, we can use the formula $F = PA$: force equals the resultant pressure exerted on the surface's center of gravity, multiplied by the surface area.

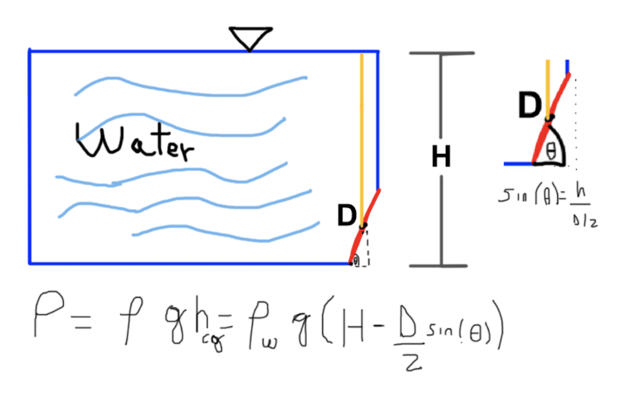
Consider the following system. A water pool of depth H is given, there's a disk-shaped gate of diameter D in the bottom with an inclination of $\theta$. Finding the resulting pressure exerted on said gate is not a hard task: we can find the depth of the center of gravity of our gate with respect to the water fluid, $h_{cg}$, with trigonometry. Then, the formula for pressure yields $P = \rho_w g h_{cg} = \rho_w g \left( H - \frac{D}{2} sin \left(\theta\right) \right)$.

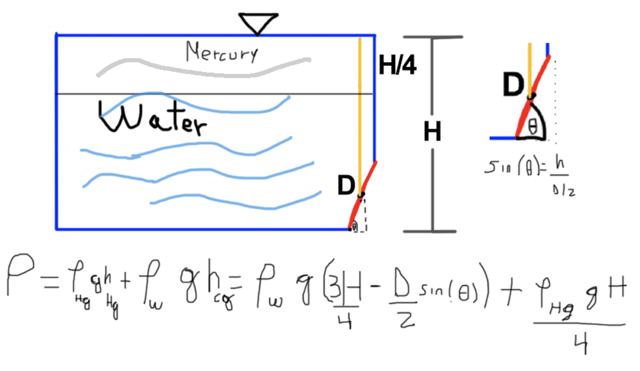
We can also deal with multiple fluids of varying density. The following image, for example, adds mercury in the top H/4 section:

It isn't hard to use the whole mercury depth $\rho_{hg} g \frac{H}{4}$ and then, once again, consider the remaining portion of water up to the center of gravity of the gate, $\rho_w g (\frac{3H}{4} - \frac{D}{2} sin(\theta))$. The resultant pressure is the sum of these two terms.

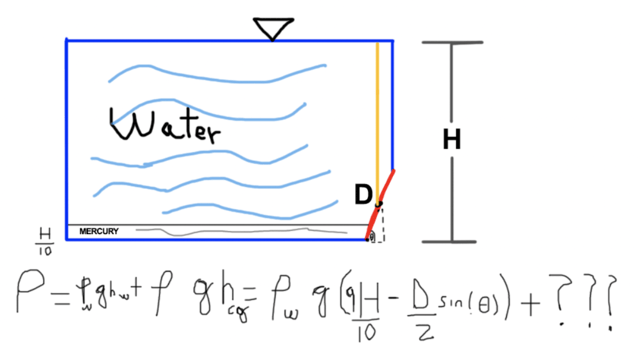
Which leads me to my actual question: What can we do in the case where the fluid goes from the bottom up? The example given below puts mercury in the bottom H/10 section of the pool. It clearly doesn't reach the gate's center of gravity. What can we do in these cases? Is there a way to project the mercury-section pressure on the center of gravity? Do we have any alternatives?

Thanks in advance!

Answer 1

Assume the water has reached the mid-height of the gate, below that is the mercury. Then the upper half of the gate is subjected to water pressure. Once the pressure diagram is drawn, you can find the force by integrating the respective pressure over the half-circle and find the force center.

The bottom half of the gate is subjected to the pressure of the water (as surcharge pressure) plus the pressure from the mercury. The procedure to find the force and force center is the same as the above.

Note the force center may not coincident with the geometry center of the half-circle.

Finally, you can find the total force applied on the gated by algebraic addition, and find the center of the applied force by taking moment about the upper or lower hinge point.

Note: The above assumes a clear interface can be maintained between the two fluids.

Answer 2

I am going to address stratified layers of liquid and for simplicity assume your gate is a square, not a disc.

First off the gravity will always place the liquids layered, heavy to light from the bottom to top. So the mercury will always settle to the bottom.

Let's call the thickness of mercury $H_M< D/2 sin\theta$ and the thickness of water $H-H_M$.

Then the hydrostatic distribution from the bottom will be two triangles with different slopes. We set the datum at the interface between the water and mercury and ignore the atmospheric pressure.

Then we have two trapezoids of pressure acting on the gate.

From the datum up to the level of the top of the gate, we have $P= D/2*sin\theta*1$ to $P=H-H_M*1$ and we know the pressure acts at the mid-height of this trapezoid.

From the datum to the bottom of the tank we have the pressure of the water above the datum plus the hydrostatic pressure of mercury.

So the top and bottom of this trapezoid are

$$P_{top}=(H-H_M)*1\ and\quad the bottom\ pressure= \ P_{bottom}=P_{top}+ H_M*13.5$$

And the resultant of these two vectors (P_w+P_m)falls at the height of

$$ H_{effective}= \frac { (P_{W}*d_{W from datum} + P_{M}*d_{mercuty from datum})}{(P_{W}+ P_{M}) } $$

Now we can apply this effective pressure at its resultant height to the gate.