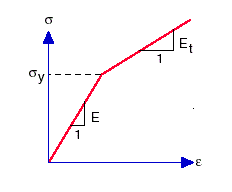
Attached you may find a picture for isotropic bilinear hardening up until the ultimate tensile strength. The first line is the elastic region while the second one is plastic. I couldn't understand that why is plasticity even a non-linearity in FEA? I mean if I just consider the graph below, then a point in a FEA model will just move over this graph only. If it switches from first line to the other, then there is just a change in the elastic modulus. If I am conducting a geometrically linear analysis, then how could inputting a material plasticity model, like the one showed below, make the analysis still be non-linear?
-
3$\begingroup$ Err its clearly nonlinear on account of it having two line segments. Everything that is not a single line in its whole domain is not linear. You need a whole different class of solvers to solve nonlinear equations because you now need to keep track of the actual path that was taken for the solution whereas for a linear solution is guaranteed to be a simple solution. You can just disregard the path and jump directly to the solution. $\endgroup$– joojaaCommented Jul 6, 2021 at 19:30
-
2$\begingroup$ "LInear" means "if you double the loads, you get exactly twice the displacements". It should be obvious this is not true for your stress-strain curve. Also, in a structure with this type of material behavior and redundant load paths, there may not be a unique solution for every possible set of applied loads. $\endgroup$– alephzeroCommented Jul 7, 2021 at 9:39
-
$\begingroup$ Understood. Makes sense. Thanks, to both of you. $\endgroup$– Rameez Ul HaqCommented Jul 7, 2021 at 12:59
-
$\begingroup$ I am curious about where you get this diagram, can you please provide the source? Thanks. $\endgroup$– r13Commented Jul 7, 2021 at 17:08
1 Answer
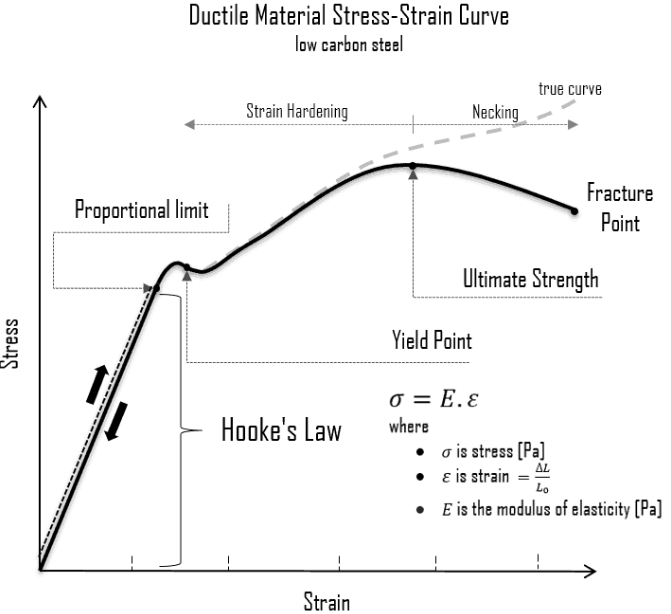
In the plastic range, the stress-strain relationship is non-linear as shown in the graph below. With consideration of geometric changes after yield, the true stress-strain curve (dotted line in the graph) shall be used instead of the normal curve, and non-linearity needs to be considered in the analysis.
Note: Due to the shrinking of section area and the ignored effect of developed elongation to further elongation, true stress and strain are different from engineering stress and strain.
$\delta_t = \delta(1 + \epsilon)$, and
$\epsilon_t = ln(1 + \epsilon)$
-
$\begingroup$ The question has nothing to do with plastic behaviour. The stress-strain curve in the OP's example is completely reversible. A real material showing a similar effect, but with the graph curving the other way, is rubber. $\endgroup$ Commented Jul 7, 2021 at 9:37
-
$\begingroup$ @alephzero On your mentioning of "rubber material", please read this quora.com/…. $\endgroup$– r13Commented Jul 7, 2021 at 16:41
-
$\begingroup$ For the downvoters, please indicate your dissent for a material that possesses linear elastic behavior up to the yield and will have some degree of permanent distortion upon unloading after stressed beyond the yield point (non-linear). And please provide the type of material that will deform linearly after yield. Thanks. $\endgroup$– r13Commented Jul 7, 2021 at 17:06
-
$\begingroup$ @alephzero I read the OP's question several times and failed to understand why a straight line was shown after the yield point unless it was an idealization for the convenience of analysis (I doubt). Also, in the opening, the OP stated "...a picture for isotropic bilinear hardening up until the ultimate tensile strength. The first line is the elastic region while the second one is plastic....", why is "plastic" if the stress and strain is linear within that range? $\endgroup$– r13Commented Jul 7, 2021 at 17:36
-
$\begingroup$ I guess I couldn't get a straight answer then. So disappointing. $\endgroup$– r13Commented Jul 8, 2021 at 11:59