1
$\begingroup$

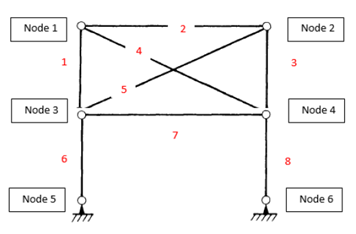
The exercise is taken from linear algebra and its applications, prof. G. Strang enter image description here

Exercise 2.4.8 Truss

I have already found that: m=n=8 → system is statically determinate, therefore solvable by equilibrium equation.

A is a 8 by 8 square matrix.

Also we know, there aren’t any rigid movements since the truss is fixed. Also, because 8-8 is 0, we're expecting 0 solutions to Au=0

I wrote the forces eq. equilibrium:

$f_{H_1 }=-y_2-y_4 cos⁡30$

$f_{H_2 }=y_2+y_5 cos⁡30$

$f_{H_3 }=-y_7-y_5 cos⁡30$

$f_{H_4 }=y_7+y_4 cos⁡30$

$f_{V_1 }=-y_2-y_4 sin⁡30$

$f_{V_2 }=y_2+y_5 sin⁡30$

$f_{V_3 }=-y_7-y_5 sin⁡30$

$f_{V_4 }=y_7+y_4 sin⁡30$

How should I continue and say something regarding the stability of the system?

Improve this question
$\endgroup$
3
  • $\begingroup$ IMHO, any lateral force would make this collapse. Nodes 1234 indeed create a statically determinate body. However, the rod 3-5 and 4-6 are parallel and of equal length. Therefore body 1234 can freely rotate. $\endgroup$
    – NMech
    Commented Jan 23, 2021 at 8:44
  • $\begingroup$ yes, but how can i show it mathematically? $\endgroup$
    – Efrat Brayer
    Commented Jan 23, 2021 at 8:54
  • $\begingroup$ @Efrat Brayer Too far away from my school years, so I can't tell you how to write the mathematic expressions required to demonstrate the stability of this system. But, if you provide a horizontal force at node 1, then you shall find horizontal forces on nodes 3 and 4, which are acting on member 7, and force it to go side way (horizontal displacement). Now you can see why the system is unstable -: the pin supports (joints 5 and 6) can't resist rotation due to the horizontal force on member 7, nor joints 3 and 4. From this observation, you shall be able to write the equilibrium equations. $\endgroup$
    – r13
    Commented Mar 13, 2021 at 14:07

3 Answers 3

Reset to default
1
$\begingroup$

IMHO, any lateral force would make this collapse. Nodes 1234 indeed create a statically determinate body.

However, the rod 3-5 and 4-6 are parallel and of equal length. Therefore body 1234 can freely rotate.

Regarding how you show that mathematically, (without knowing the context of your assignment):

  • 1234 is a rigid body
  • you can substitute 1234 with a single rod 34 .
  • for the new system you have nodes 3,4,5,6 and rods 53, 34, 56.

Therefore in the new equivalent system you have 4 nodes and 3 rods. Therefore, there are 8 unknowns and only 6 equations.

Improve this answer
$\endgroup$
0
$\begingroup$

The frame is externally unstable.

ED = R - 3 - S = 4 - 3 - 4 = -3 < 0, unstable.

R = number of support reaction S = number of internal joint releases (4 moment releases at the hinges)

Improve this answer
$\endgroup$
0
$\begingroup$

You got the horizontal equilibrium equations correct. Now add them all up and you'll get $f_{H_1} + f_{H_2} + f_{H_3} + f_{H_4} = 0$. That equation says that the sum of all external loads equals zero. So any combination of these that doesn't fulfill that requirement will result in collapse of the structure, mathematically represented by the equation's LHS not being equal to the RHS. For example, if $f_{H_1} = 1$ and all of the others = 0, you'll get 1 = 0, so mechanically speaking the system is not in equilibrium.

You do not need the vertical direction to solve the task, but it can demonstrate the difference. You got the vertical equations wrong, though, because you kept the hotizontal trusses in there. You need to leave those out and account for the vertical ones instead (apart from 1 and 3, 6 and 8 will also appear). Now if you get them right and add them up just like the horizontal ones, you'll see that there will actually be unknowns left, not just external forces, which means that strictly in the vertical direction, the structure is not unstable.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.