How high will a flywheel catapult launch a payload?

Question

First off, this is not a homework question or anything like that. I'm trying to build a catapult to launch a payload using a flywheel as an energy device!

It goes like this:

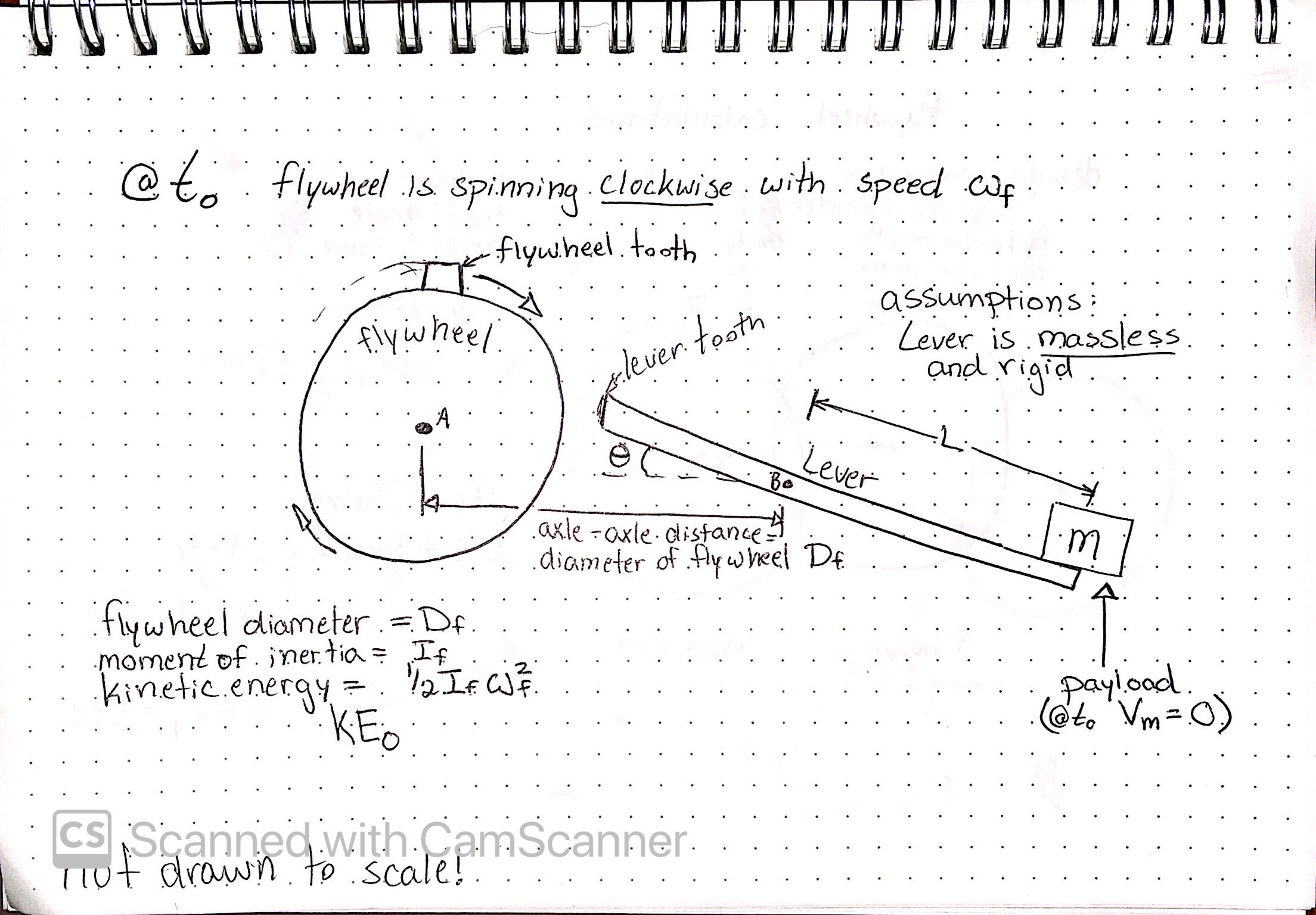
1. The flywheel spins up to maximum speed. All other components are at rest.
2. The catapult lever (in resting position, with a payload at the end of it) engages a tooth.
3. The flywheel tooth (always extended) connects with the catapult lever tooth and rotates it over a certain angle. During this time the flywheel is slowed down a certain amount and the lever gains a great amount of speed.
4. At the end of the interaction between the flywheel and the lever tooth (after some degree of rotation) the lever gets to the end of its stroke and abruptly stops, the payload continues flying upward and the remaining energy of the flywheel makes it continue to spin (it can freely "slip" past the lever tooth at the end of the movement).

I've attached a couple pictures which describe the two states, T0 and T1 (basically step 3 and 4 respectively).

My question is, how do I determine the final energy of the flywheel after this interaction given the following parameters: Moment of Inertia and initial Kinetic Energy of the flywheel, mass of payload, and the basic geometry between them. Assume the weight and inertia of the catapult lever are negligible and there is no friction between the interaction of the two moving parts.

I originally thought this problem would be as easy as assuming all the kinetic energy of the flywheel just went into the upward motion of the mass (the flywheel would come to a complete stop). However, after thinking about it for a while I realized it is probably not that simple at all...I smell some differential equations which scare me and has been a while since I've done any of that which is why I am asking for some help. Maybe it's not that complicated after all, but I am at a dead end. Anything would be appreciated.

Thanks in advance.

Answer 1

This is a very interesting problem.

Energy approach

At first I was inclined to solve it though energy i.e.:

$$\frac{1}{2}I_{fly}\omega_0^2 = \frac{1}{2}I_{fly}\omega_1^2 + \frac{1}{2}I_{lev}\omega_1^2 + \frac{1}{2} m \cdot (\omega_1\cdot L)^2$$

where:

• $I_{fly}$ is the moment of inertia of the flywheel $I_{fly} = \frac{1}{2} m_{fly} r_{fly}^2$
• $I_{lev}$ is the moment of inertia of the lever $I_{lev} = \frac{1}{12} m_{lev} \left(L+ \frac{D_{fly}}{2}\right)^2 + m_{lev}\left(\frac{L-D}{2}\right)^2$
• $\omega_0$, $\omega_1$: are the angular velocities at the beginning and end of engagement respectively.
• $m_{lev}$: mass of the lever
• $m$: mass of the "missile"

From this equation, it is pretty straight forward to obtain the $\omega_1$:

$$\omega_1 = \sqrt{\frac{I_{fly}}{I_{fly}+ I_{lev}+ m \cdot L^2}}\omega_0$$

Then the only thing you need to do is work out the launch angle ($\theta$), break up into components and you can estimate maximum height and horizontal distance traveled.

Although, I believe the above mentioned method will give you a ball-park figure, I doubt it will be accurate. There are two assumptions 'with issues' here:

• there is full engagement during the duration of the slow down of the flywheel (i.e. there is no impact).
• disengagement between the mass and the level occurs the moment the engagement of the tooth with the lever finishes. However, the time of disengagement of the mass will be depended on factors such as how its mounted on the level, coefficient of friction etc.

Impact

The main problem with the above approach would be the speed of impact during first engagement.

If the lever and mass are small then the angular velocity of the level + mass will be greater than the angular velocity of the flywheel, i.e. there will be only brief contact at the beginning of the impact and maybe secondary impacts (which might not provide any extra energy to the mass.)

If the lever and mass are large then probably what will happen is that the flywheel will either recoil or slow completely down. In any case, this means that the travel angle will not be $\theta$ but something else entirely.

Answer 2

As an upper limit - the maximum power transfer theorum says maximum power is transferred when energy in the flywheel and the mass are shared equally.

Mass energy becomes 0.5 x m x v^2 and
max height is given by E=mgh so

Height_max = E_flywheel/(2 x m x g)

In reality actual energy transferred and consequent height will approach but not equal this.

eg 100 gram mass, 100 Joule flywheel energy
Height <= E_flywheel/(2 x m x g)
= 100 /(2 x 9.8) m
~= 5 metres

Drag coefficient of the projectile will alter actual height.

At "launch" E_flywheel/2 = 0.5 x m x V^2
so V = (Ef / m)^0.5
In the above example
V = sqrt(100/.1) ~= 32 m/s
That's fast enough for drag to make a significant difference depending on frontal area and profile.