Does the volume change under the tensile or compressive stress?

Question

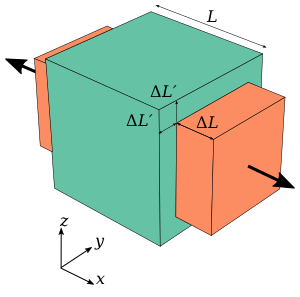
This confusion arise because my teacher says that volume does not change under tensile/compressive stress within the elastic limit of the material ( consider metal here). But as far as I can see via applying Poisson's ratio it does ( otherwise lateral strain would be proportional to square root of longitudinal strain). So does the volume chaneg under tensile stress ? What might be the molecular basis of such?

---

I defined a quantity (cause it was simple to calculate) $ \frac {\Delta A}{A}$ and then found that

via **Poisson's ratio

$$ \frac {\Delta A}{A} = \alpha \epsilon _{lon}( \alpha \epsilon _{lon} +2)$$

whereas the one derived via assuming the volume to be constant (i.e., $AL = A_0 L_0$) was $$ \frac {\Delta A}{A} = \frac {\epsilon _{lon}}{\epsilon _{lon} +1}$$

Here $ \alpha = \frac {\epsilon_{lat}}{\epsilon_{lon}}$

Answer 1

Yes, the volume changes.

The relative change of volume $ΔV/V $of a cube due to the stretch of the material :

Using $V = L^3$ and

$V + \Delta V = (L + \Delta L)\left(L + \Delta L'\right)^2$:

:$\frac{\Delta V}{V} = \left(1 + \frac{\Delta L}{L} \right)\left(1 + \frac{\Delta L'}{L} \right)^2 - 1$

Using the above derived relationship between $\Delta L$ and $\Delta L'$:

:$\frac {\Delta V} {V} = \left(1+\frac{\Delta L}{L} \right)^{1-2\nu} - 1$

and for very small values of $\Delta L \ and \ \Delta L'$, the first-order approximation yields:

$$\frac {\Delta V} {V} \approx (1-2\nu)\frac{\Delta L}{L}$$

For isotropic materials, we can use Lamé parameters

$$ \frac{1}{2} - \frac{E}{6K}$$

where K is bulk modulus and E is elastic modulus or Young's modulus. wikipedia link