How can I calculate "bendability" of aluminium tubes?

Question

Disclaimer: sadly not an engineer (I hope this is the right place for such my question. Please let me know if it isn't).

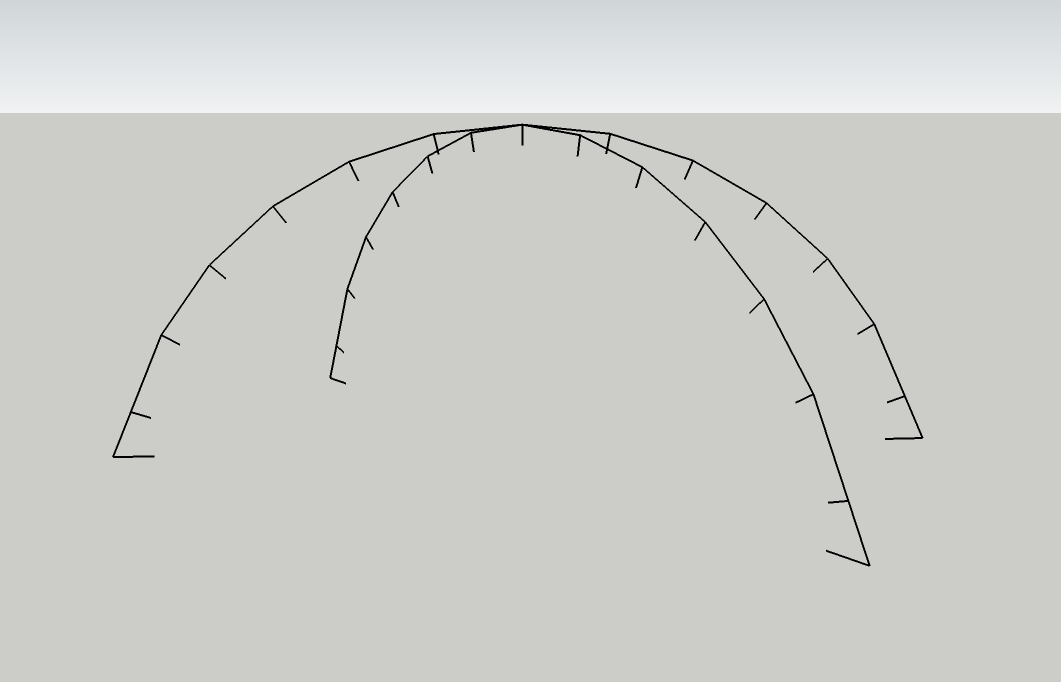
I'm designing a tent. I know most of the dimensions of the first prototype I want to build, but I'm struggling with the poles.

Don't mind the short lines

When inserting the poles into the rings at the extremities of the tent base (groundsheet), they bend to form the shape of the tent, as we can see in this Naturehike Cloud Up 2 pitching video.

In my design, I need to be able to predict how much (how far) the poles will be able to bend. I'd love to use Easton's Syclone poles, but I doubt they'd sell them to me. So I'm interested in aluminium poles such as these:

Knowing this is essential for my project, because the bendability of the poles will determine lots of aspects of the design (such as pole parts length for example).

So, my question is: how can I predict (or simulate [that'd be awesome]) the bendability of my poles? Any suggestion is immensely welcomed.

Answer 1

As has been said this question lends itself to empirical test better.

But just to answer your question hypothetically: $$\frac{1}{R}= \frac {M}{EI} $$ Meaning the more moment or less the stiffness of the aluminum pipe, the more it bends.

if you need to calculate the I or second moment of area of the pipe:

$ I_x = \pi (D^4-d^4)/64 $

Where D is the outside radius and, d the inside one.

And E is the Young modulus of the particular alum you use, which you can find it in the manufacturer data sheet.

Edit

So let's just assume as an example we pick 1/2inch by 1/16 inch thickness aluminum pipes 6061-T6 with E= 10000ksi and yield at 40000psi.

First, we define. C= 1/2 diameter= 1/4inch,

And $ I=\pi( \frac{ 1}{2}^4-\frac{3}{8}^4)=0.0209inch^4 $ We know $$ \sigma= \frac{M*C}{I} $$

Let's assume 75% of the tube's yield strength $$40000*0.75 =30000psi\quad 30000=\frac{1/4M}{0.0209} $$

from here we find the M and plug it into the original answer and find the R radius with a safety factor of 1.25%.

And for simlicity we assume the overlapped section of the pipe to be rigid and not bending.

Answer 2

I don't know how to calculate it, but you're going to need something like a 6063 T9; it's drawn then aged. But as Chris states below, it's the yield strength that you need a lot of. It might need to be something in the 7xxx series, or also as below, something in a composite.

Answer 3

At the most basic there are two key properties you need to look at.

The yield stress of the material determines how much stress the poles will take before they are permanently deformed. The elastic modulus (Young modulus) determines how far they will bend for a given load.

To calculates stresses and deflection you will need to start with beam bending equations. https://www.engineersedge.com/beam_bending/beam_bending9.htm Just in terms of calculating max curvature it is probably best to treat it as a canilever with a load at one end.

You will also need the inner and outer diameter of the tube to calculate moment of inertia (aka second moment of area).

This is complicated somewhat by the fact that the joints will have different stiffness to the rest of the pole so you may be best off calculating for one length and working with the resulting radius.

This doesn't take into account other loads on the poles but should at least give you an order of magnitude.

Equally it is usually a good idea to look at existing designs to get an idea of what is feasible.

I would also reiterate the advice in the comments that unless you really know what you're doing practical experimentation is likely to be of more practical use than theoretical analysis.

You may also want to consider composites. Certainly GRP is readily available as prefabricated rod and tube and is not terribly expensive,