Elastic (Young's) Modulus relation to the Eigenfrequency

Question

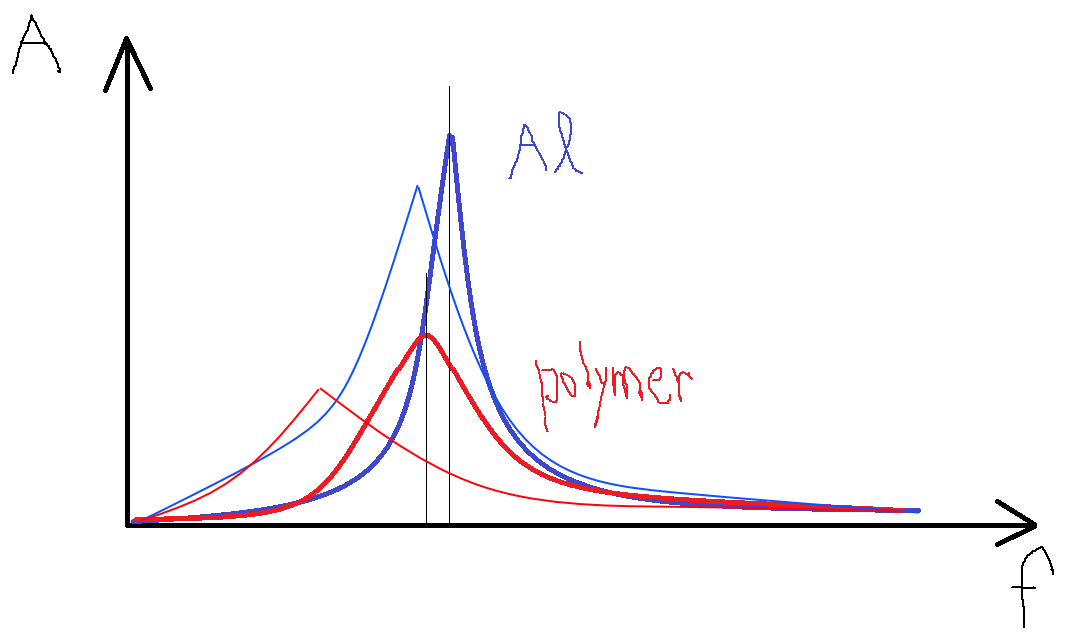
I would like to compare the shape of the frequency spectrum of a plastic and aluminium component. Now if we assume that the plastic and aluminium component only differ in the material, while the mass and stiffness stay the same (I know, large assumption), how would generally differ the shape of the frequency spectrum from each other? Obviously, the damping plays a major role here and would be much higher in case of plastic, therefore the response amplitude would be lower, the resonance zone wider and a shift (which is many times neglected) towards a lower frequency would be made... Could I in a very simplified version, just for the sake of understanding, draw it like this? Another question would be the temperature dependence of the elastic modulus. If the temperature rises, the modulus is reduced which also affects the eigenfrequency. In case of aluminium not so much, but in case of plastics, the difference can be enormous. My understanding is that in case of elevated temperatures, the damping increases; in case of plastic much more than in case of aluminium. Could I visualize this as:

Any constructive answers are much appreciated! Kind regards,

Luka

Answer 1

In the perfectly elastic spectrum, response to the dampening coefficient of the second order differential equation of vibration behaves very similar to how you have shown, but not quite. It is well documented by reviewing how a system responds when subjected to a forced time-varying load at different speeds. Notice how it doesn't get wider for the frequency shifts as the dampening increases:

$$\begin{gather} x(t) = X_0 \sin(\omega t + \phi) \\ X_0 = \dfrac{KF_0}{\sqrt{\left(1-\dfrac{\omega^2}{\omega_n^2}\right)^2+\left(2\varsigma\dfrac{\omega}{\omega_n}\right)^2}} \\ \phi = \tan^{-1}\left(\dfrac{-2\varsigma\dfrac{\omega}{\omega_n}}{1-\dfrac{\omega^2}{\omega_n^2}}\right) \end{gather}$$

However, as time goes on, the natural frequency of a material experiencing creep will vary. While creep adds to the dampening, it adds some other time-dependent side effects. From Wikipedia:

Creep (sometimes called cold flow) is the tendency of a solid material to move slowly or deform permanently under the influence of mechanical stresses. It can occur as a result of long-term exposure to high levels of stress that are still below the yield strength of the material.

As a result of creep, the natural frequency of the curve can become wider over time, but that's somewhat speculative until the actual creep mechanism is understood and the governing PDEs are resolved. However, this could give results similar to your temperature plot.