How to handle modal combination in response spectrum analysis for member forces for member with more than 1 element?

Question

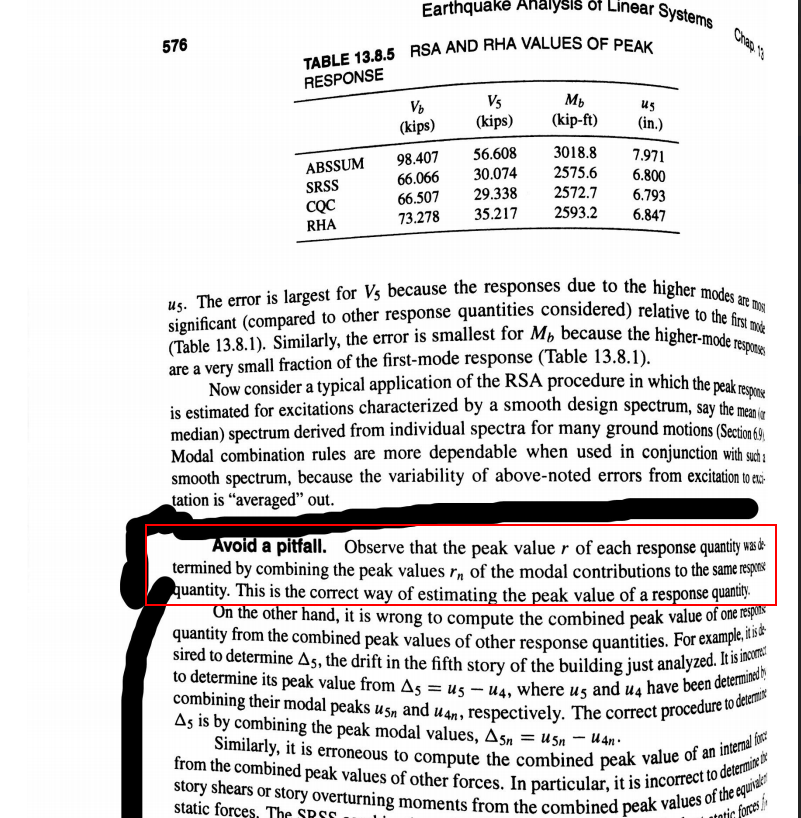
My textbook says for mode combination, the peak value $r$ of each response quantity is determined by combining the peak values $r_n$ of the modal contributions to the same response quantity.

From what I understand, this means that we have to calculate the response (be it displacement, the difference in displacement, the forces or moment) for each mode first, before we apply the modal combination method such as SQSS, CQC and ABS in order to obtain the total response.

This is also verified by the numerical example in the textbook.

However, in the textbook example, all the members are 1-element members, easy to interpret the above clause in the textbook. What about members (beams, columns) that are of more than one element? In FEM we often create a single member out of multiple elements.

Do we combine the element response into member response on per mode basis first, before we combine all the member modal responses into total member response?

Or do we combine the total modal response for each element, and then only combining the element response into member response?

In other words, is the modal combination done at element level or at structural member level?

Answer 1

You are correct that the response for each mode must be calculated before applying some modal combination methodology.

Instead of thinking about the structure as being discretized into numerous elements, I like to think of it in terms of the lumped masses and how many DOFs there are. A more complex structure will simply have more DOFs (and therefore more modes) than a simple 'lollipop' model. But you still come up with modes for the structure as a whole - not modes for individual elements within the structure.

Since you mention RSA, I'm assuming you're approaching all this from the perspective of structures subjected to earthquakes. During an earthquake, multiple modes will be excited, depending on the frequency content of the earthquake. How then, do you determine the overall structure response? RSA is a simplified tool for seismic design -- allowing you to first determine the peak response of the individual modes and then combine those responses using SRSS, CQC, etc. This is opposed to running time-history analyses (very computationally intensive, but sometimes done for complex and/or important structures).