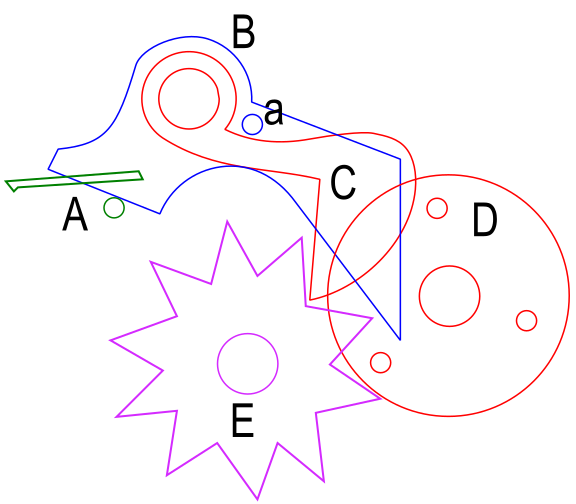
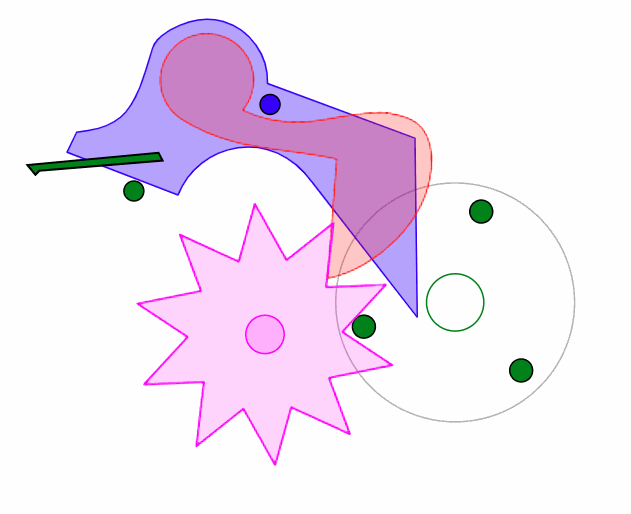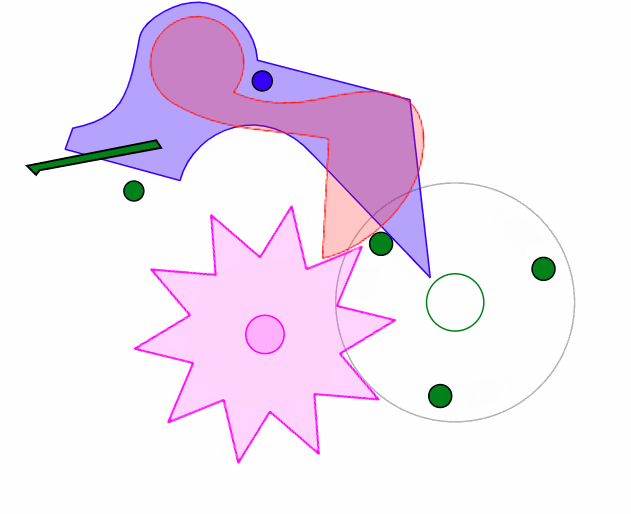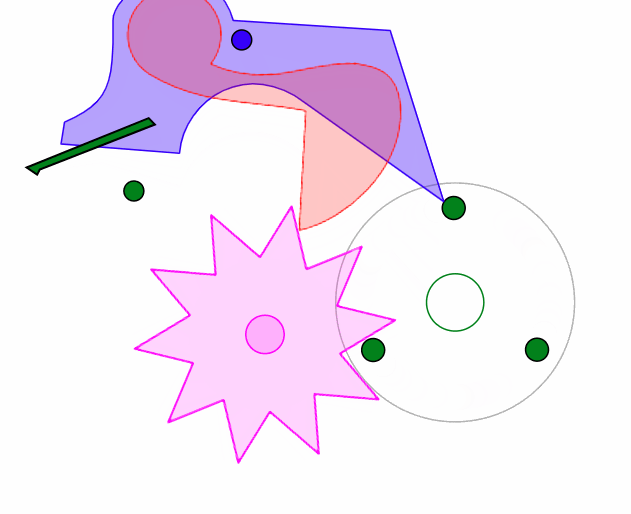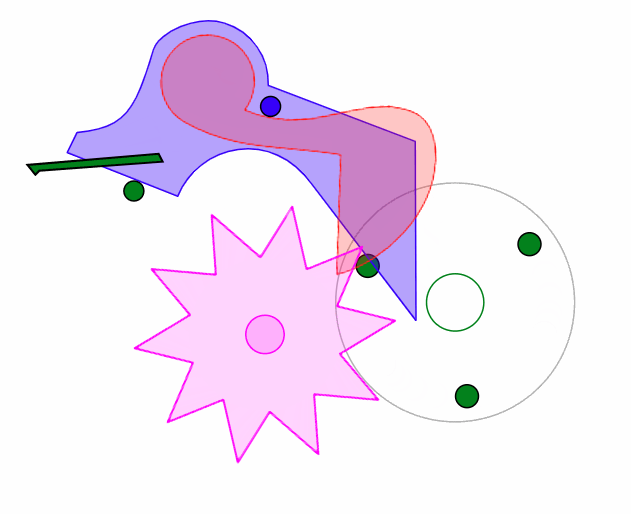
From 507 mechanical movements, No. 63, we get the following diagram and description.
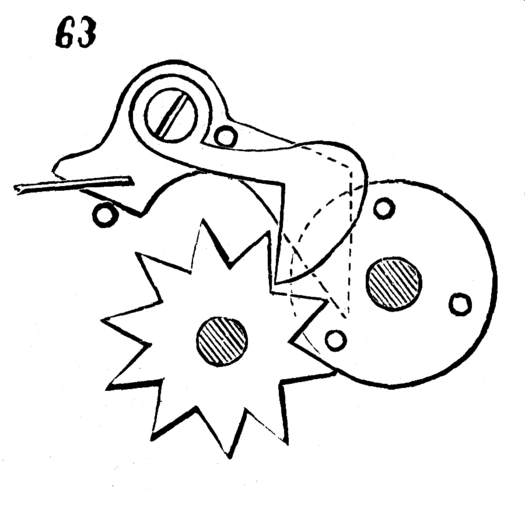
- Jumping or intermittent rotary motion, used for meters and revolution-counters. The drop and attached pawl, carried by a spring at the left, are lifted by pins in the disk at the right. Pins escape first from pawl, which drops into next space of the star-wheel. When pin escapes from drop, spring throws down suddenly the drop, the pin on which strikes the pawl, which, by its action on star-wheel, rapidly gives it a portion of a revolution. This is repeated as each pin passes.
I redrew the figure with colors and labels to help the discussion here:
As far as I can tell, the author means this: the wheel $D$ with pins is rotating clockwise, coming into contact with the "drop", $B$ (blue) and the pawl $C$ (red). The drop also has a pin $a$ which can collide with the pawl. It's not clear whether the components $A$ (in green) are the "spring at the left" that he mentions. According to the description, a pin of $D$ escapes the pawl first, the pawl then drops into the next space of the star wheel; then the pin escapes the drop, which (due to the spring) is thrown down, and what I think is the pin $a$ strikes the pawl, which "by its action on star-wheel", causes a rapid rotation.
But how does the pawl work here? If the pawl and star-wheel are coplanar, does that mean the pins of $D$ cause both the star-wheel and the pawl to be deflected? How does a pin catch the pawl anyway? From the drawing it's not clear. Would the pawl allow rotation of the star-wheel in both directions? It seems close to being able to move against the star-wheel when the star-wheel moves counterclockwise (so the pawl goes up, not down). I doubt the drawing is accurate in terms of scale and how things fit, but even being loose with the interpretation I'm having a hard time understanding what he means.
UPDATE 1: I tried to partially animate the mechanism based on the comment from @jsotola, and maybe this will help clarify things.
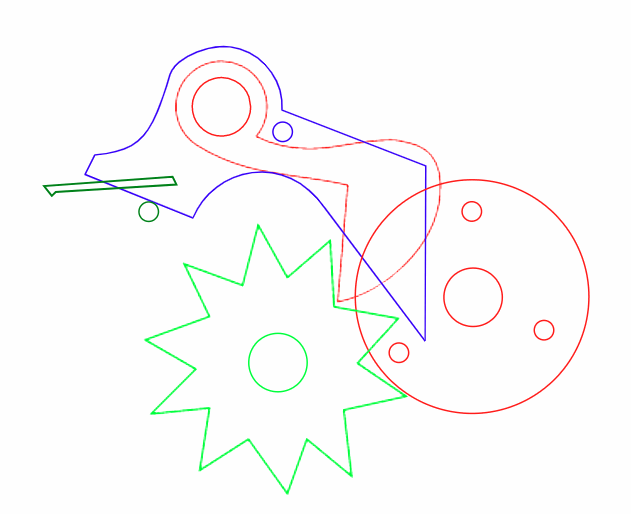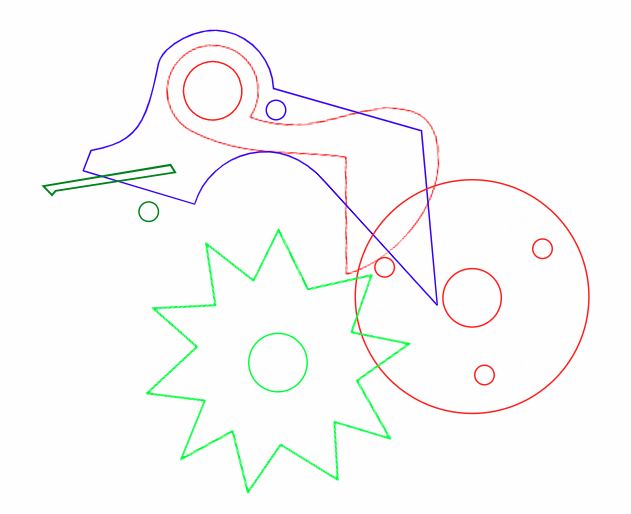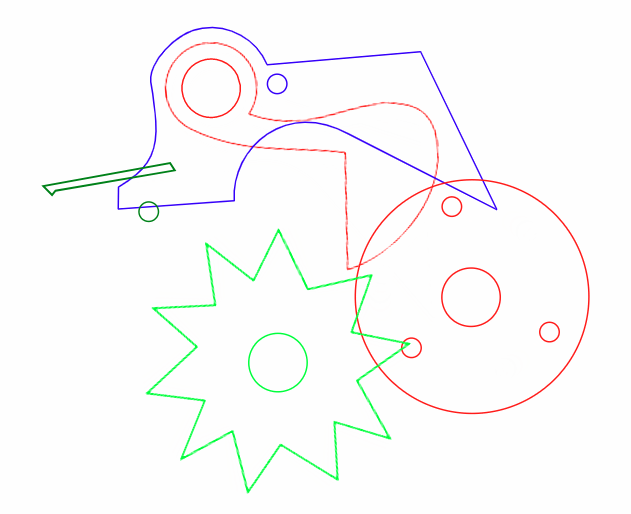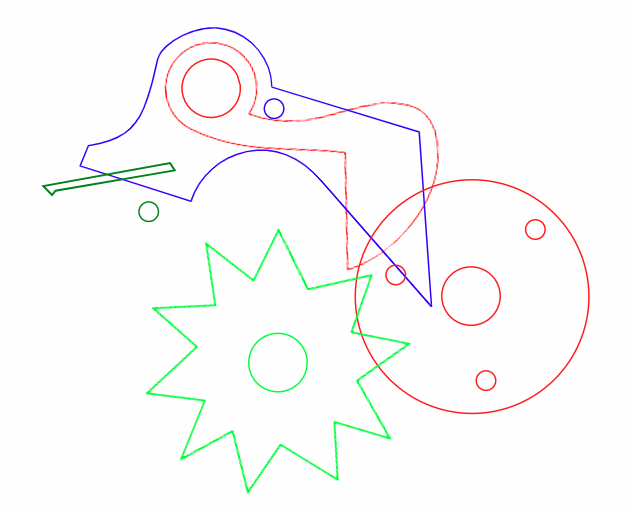 [Old Image, see Update 2]
It's hard to animate this by hand because I'm not sure if the pivots are correct, e.g. does the BC assembly pivot on A as shown, and do they rotate relative to each other?
[Old Image, see Update 2]
It's hard to animate this by hand because I'm not sure if the pivots are correct, e.g. does the BC assembly pivot on A as shown, and do they rotate relative to each other?
UPDATE 2.
I redrew the animated parts to be more idealized, and moved the driving wheel a bit closer. The result has some obvious issues, and I wonder how the pawl hitting the star wheel is supposed to make it rotate rapidly? Which direction is it expected to move?