Let the pivot be the point $(0,0)$ , and let's show the coordinates of the other end of the rod with $x$ and $y$. Note that $$x=Lcos\theta \quad,\quad y=Lsin\theta$$
and therefore
$$dx=-Lsin\theta d\theta \quad,\quad dy=Lcos\theta d\theta \quad (1)$$
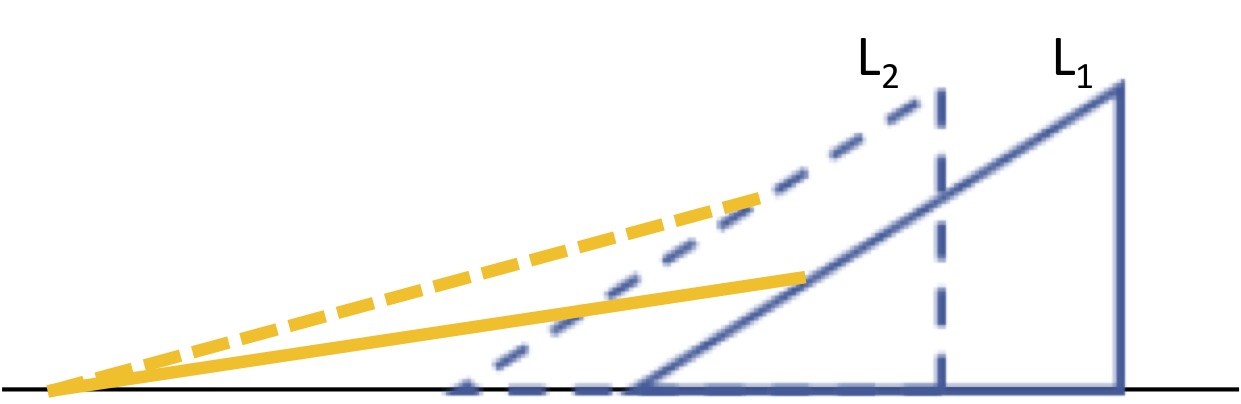
In time $dt$ , the rod and the wedge move as shown above. Solid lines and dashed lines correspond to beginning and end of this time interval, respectively. Suppose the sliding end of the rod moves in this time interval from $(x_1,y_1)$ to $(x_2,y_2)$ . The difference between the $x$ coordinates of these points is $dx$ , and the difference between their $y$ coordinates is $dy$ .
The horizontal displacement of the wedge in time $dt$ is $vdt$. Let the oblique edge of the wedge before and after this displacement be represented by $L_1$ and $L_2$, respectively. The equations of these two lines are: $$L_1 : \quad x = y cot \phi + b $$ $$L_2 : \quad x = y cot \phi + b + vdt $$ Note that $(x_1,y_1)$ is on $L_1$ and $(x_2,y_2)$ is on $L_2$. So: $$b = x_1 - y_1 cot \phi $$ $$x_2 = y_2 cot \phi + (x_1 - y_1 cot \phi) + vdt $$ By rearranging and noting that $x_2 - x_1 = dx$ and $y_2 - y_1 = dy$ we have: $$dx - cot \phi dy = vdt $$ And by substitution from (1) we have: $$-L(cos\theta+cot\phi cos\theta)d\theta = vdt $$$$-L(sin\theta+cot\phi cos\theta)d\theta = vdt $$ Or: $$\frac{d\theta}{dt} = \frac{-v}{L(cos\theta+cot\phi cos\theta)}$$$$\frac{d\theta}{dt} = \frac{-v}{L(sin\theta+cot\phi cos\theta)}$$ $$= \frac{-vsin\phi}{Lcos(\theta -\phi)} $$ Finally, note that in this particular example, where the wedge is moving towards left, $v$ is negative. Therefore $\frac{d\theta}{dt}$ is positive.