By the context it means a beam with an infinite number of degrees of freedom, which means a full continuum solution for the deflection equation.
You can work this out by the fact that the first drawing shows the deflection as $v(x,t)$, the second drawing (a 1-dof model) shows the deflection as $v(t)$ (and there is an underlying interpolation function implicit in the black line) and the third drawing shows the deflection as $v_1(t)$, $v_2(t)$ and $v_3(t)$.
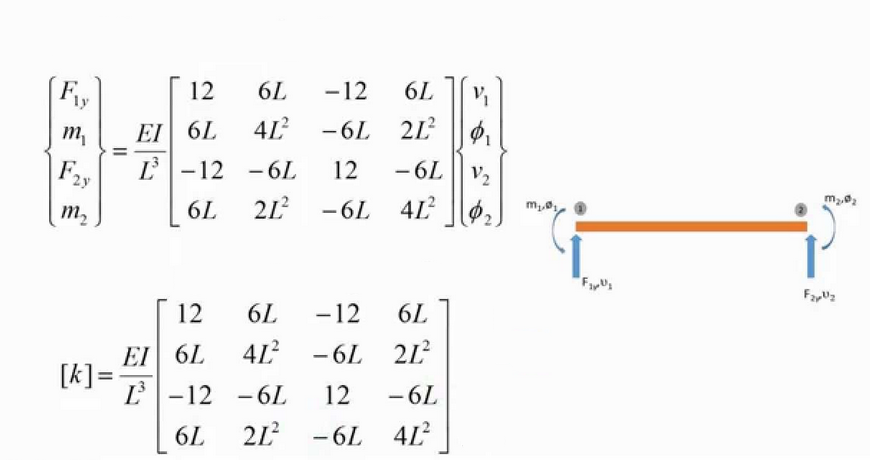
These types of discretizations are common when working on frame theory. Perhaps during your studies you've encountered a situation like the picture below.
This is a 4 dof discretization of a beam element. If you fix the left end and condense the rotation into the deflection you'll end up with the second case on your picture. These are all idealizations of the real behavior of the beam, which is the distributed mass case shown in the first picture.
Edit: I forgot to add an example of a distributed mass beam you may have encountered in classes or introductory literature. Euler-Bernoulli beam theory states the deflection of a beam is given by $$ \frac{d^2}{dx^2}\left(E(x)I(x)\frac{d^2v}{dx^2}\right)=q(t) $$ If you solve that differential equation (with appropriate boundary conditions) you'll get the continuous solution for displacement, which would be the $v(x,t)$ shown in the first picture.