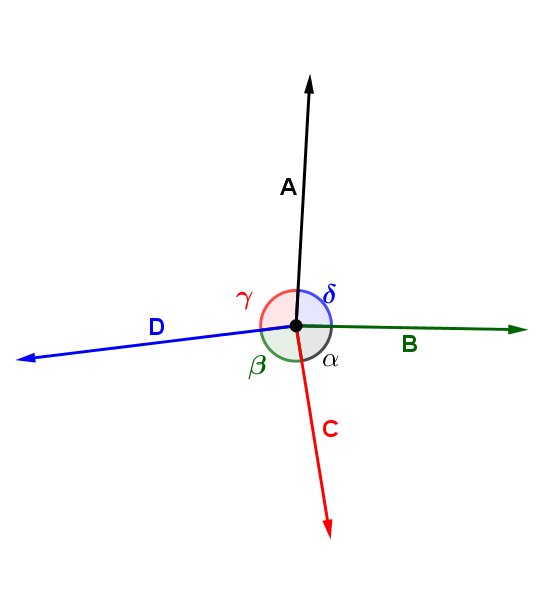
In physicsstatics, Lami's theoremLami's theorem is an equation relating the magnitudes of three coplanar, concurrent and non-collinear vectors, which keeps an object in static equilibrium, with the angles directly opposite to the corresponding vectors. According to the theorem
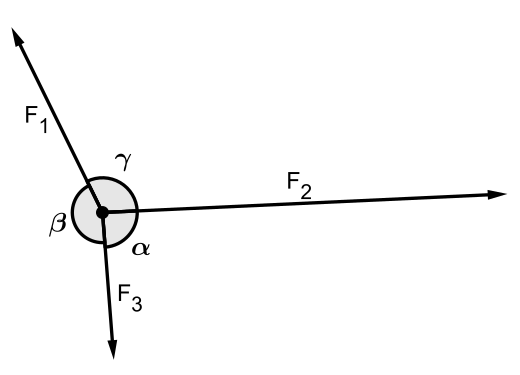
$$\frac{A}{\sin{\alpha}}=\frac{B}{\sin{\beta}}=\frac{C}{\sin{\gamma}}.\tag{1}$$$$\frac{F_1}{\sin{\alpha}}=\frac{F_2}{\sin{\beta}}=\frac{F_3}{\sin{\gamma}}.\tag{1}$$
where $A$$F_1$, $B$$F_2$ and $C$$F_3$ are the magnitudes of the three coplanar, concurrent and non-collinear vectors, $V_A$, $V_B$, $V_C$, which keep the object in static equilibrium, and $α$$\alpha$, $β$$\beta$ and $γ$$\gamma$ are the angles directly opposite to the vectors. See an illustration here(see Figure 1).
Lami's theorem is applied in static analysis of mechanical and structural systems. The theorem is named after Bernard Lamy. Its proof is essentially based on the law of sines.
On the Internet there are hundreds of static equilibrium problems where they apply Lami's theorem to a three-force system, see for instance Bernard LamyDubey - Engineering Mechanics: Statics and Dynamics, section 3.10. Although Dubey's book is recent (2013), there is not a single equilibrium problem based on a four-force system. Coincidentally, the author of this note has come across questions on the Internet questioning the possibility of applying Lami's theorem for more than three forces. In this note we give a generalization of Lami's theorem for four forces.
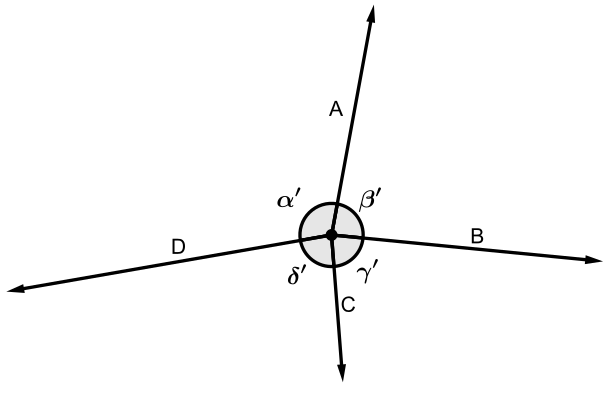
Theorem 1 (Generalization). If four coplanar, concurrent and non-collinear vectorsforces act upon an object, and the object remains in static equilibrium, then
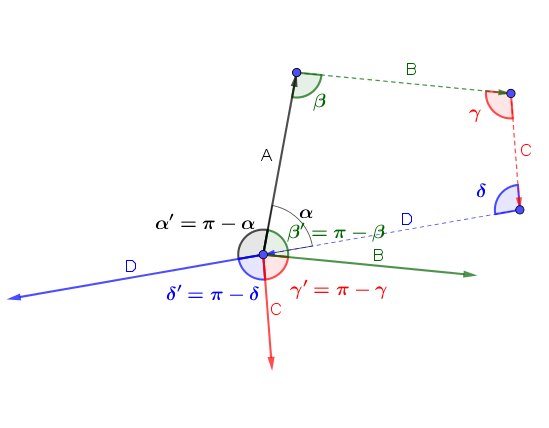
$$\frac{AB+CD}{\sin{\alpha}}=\frac{AD+BC}{\sin{\beta}}=\frac{AB+CD}{\sin{\gamma}}=\frac{AD+BC}{\sin{\delta}}.\tag{2}$$$$AD\sin{\alpha'}+BC\sin{\gamma'}=AB\sin{\beta'}+CD\sin{\delta'}.\tag{2}$$
where $A$, $B$, $C$ and $D$ are the magnitudes of the four vectors and $\alpha$$\alpha'$, $\beta$$\beta'$, $\gamma$$\gamma'$ and $\delta$$\delta'$ are the angles between the vectors such that $\alpha+\gamma=\pi=\beta+\delta$them (see figure aboveFigure 2).
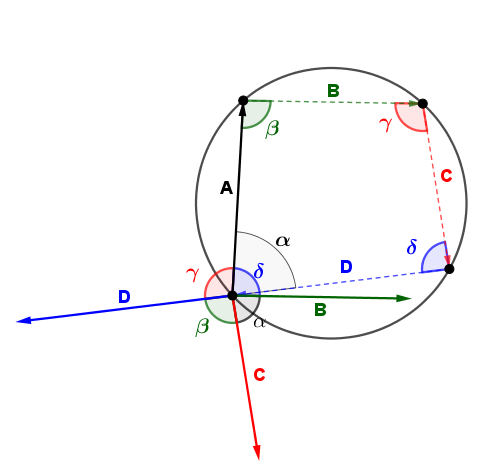
Proof. The proof relies on our generalization of the sine law, which is not hard to prove. Indeed, considerConsider the cyclic quadrilateral formed by the four vectors in such a manner that the head of one touches the tail of another (see figure belowFigure 3) and denote $\Delta$ its area. Keep in mind thatIf $\alpha+\gamma=\pi=\beta+\delta$. Since$\alpha$, $\Delta=(AD+BC)\sin\alpha=(AB+CD)\sin\beta$$\beta$, $\gamma$ and $\delta$ are the interior angles of the quadrilateral, then its area can be written as
$$\Delta=\frac12AD\sin{\alpha}+\frac12BC\sin{\gamma}=\frac12AB\sin{\beta}+\frac12CD\sin{\delta}\tag{3}$$
and as $\sin{\alpha'}=\sin{(\pi-\alpha)}=\sin{\alpha}$, and similarly for $\beta'$, $\gamma'$ and $\delta'$, the relation in $(2)$ follows.
$\square$
The relation in $(2)$ generalizes $(1)$Theorem 1 is a generalization in the sense that if one of the vectors vanishes, the relation we obtain is that of Lami's theorem. Consider the relation $$\frac{AB+CD}{\sin\alpha}=\frac{AD+BC}{\sin\beta}.\tag{3}$$
Suppose thatIndeed, for instance suppose $C=0$, then the relation $(3)$$(2)$ reduces to
$$\frac{B}{\sin\alpha}=\frac{D}{\sin\beta},$$
which $$D\sin{\alpha'}=B\sin{\beta'},\tag{4}$$ which is Lami's theorem.
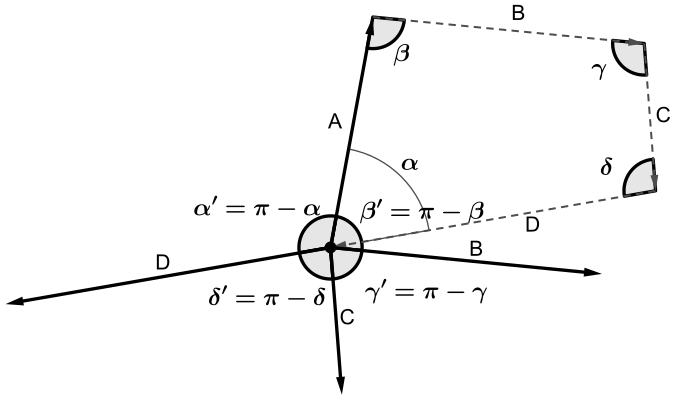
Generalization for a general quadrilateral. If four coplanar, concurrent and non-collinear vectors act upon an object, and the object remains in static equilibrium, then
$$AD\sin{\alpha'}+BC\sin{\gamma'}=AB\sin{\beta'}+CD\sin{\delta'}.\tag{4}$$
where $A$, $B$, $C$ and $D$ are the magnitudes of the four vectors and $\alpha'$, $\beta'$, $\gamma'$ and $\delta'$ are the angles between the vectors (see figure above).
Proof. Since the area of the general quadrilateral can be written as $$\Delta=\frac12AD\sin{\alpha}+\frac12BCsin{\gamma}=\frac12AB\sin{\beta}+\frac12CD\sin{\delta}\tag{5}$$ and as $\sin{\alpha'}=\sin{(\pi-\alpha)}=\sin{\alpha}$, and similarly for $\beta'$, $\gamma'$ and $\delta'$, the relation in $(4)$ follows.
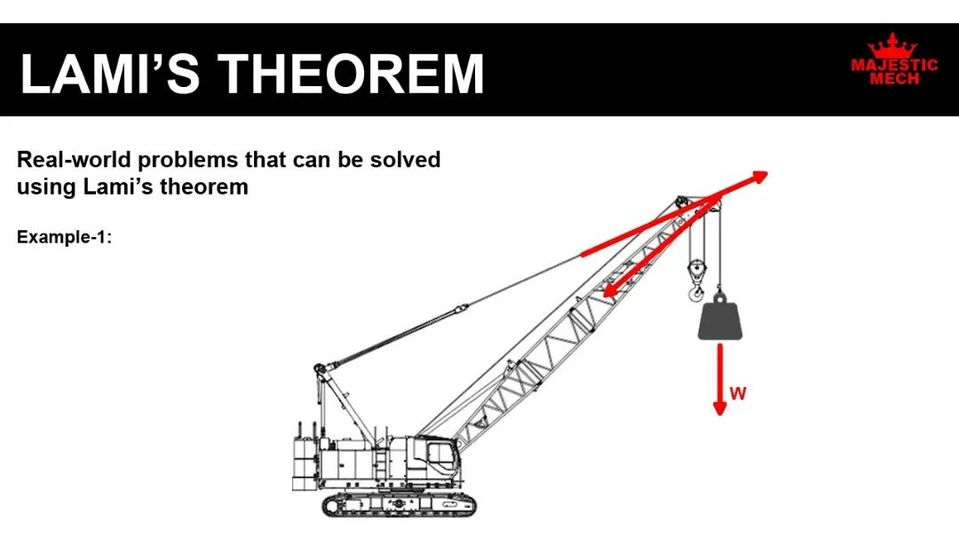
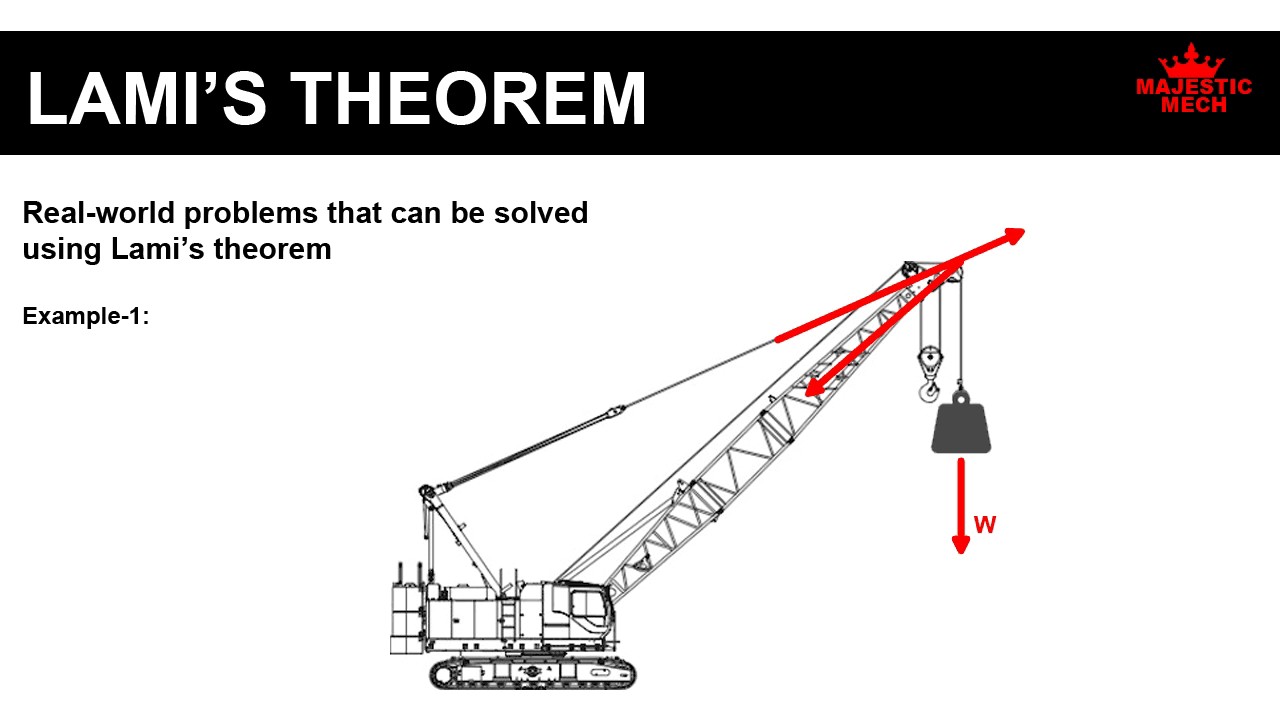
Questions: Is Lami's theorem used in the design of cranes as the image below suggest? If so, could the generalization presented be used in the design of more efficient cranes given that we have 4 vectors instead of 3?
.svg){kind=link}