(edit 3/31/2018. Completed all equations for uniform load. It will take a few days to complete and correct this answer.)
I would split the trapezoidal load
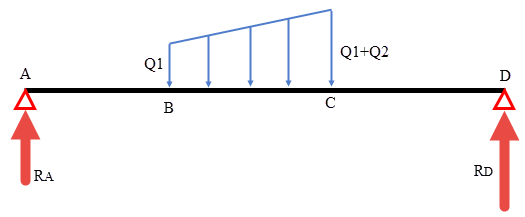
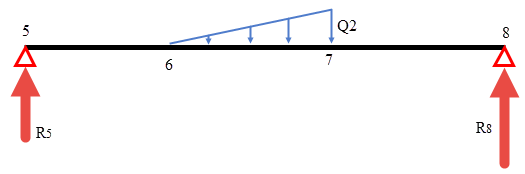
into the sum of a uniform load and triangular load
and triangular load
The reactions $R_1$, $R_5$, $R_4$, and $R_8$ can be calculated from statics. $$R_4=Q_1L_{23}\frac{L_{12}+\frac 12L_{23}}{L_{14}}$$ $$R_1=Q_1L_{23}-R_4$$ $$R_5=\frac 12Q_2L_{67}(\frac 13L_{67}+L_{78})\frac{1}{L_{58}}$$ $$R_8=\frac 12Q_2L_{67}-R_5$$
The uniform and triangular loads can be integrated section by section to get the shear, moment, slope, and displacement. In each of these equations, x is measuresmeasured from the previous point, not the beginning of the beam. Using the following equations, it is easy to create a spreadsheet to calculate the value at each point $V_1, V_2, \ldots, \delta_7, \delta_8$ or at any points in between using the equations that are functions of (x).
Uniform load, shear: $V(x)=V_i+\int load(x)\,dx$ where i is at the start of the segment and load(x)=$Q_1$ between points 2 and 3. $$V_{1}=R_1$$ $$V_{12}(x)=V_1$$ $$V_{2}=V_1$$ $$V_{23}(x)=V_2-Q_1x$$ $$V_3=V_2-Q_1L_{23}$$ $$V_{34}(x)=V_3$$ $$V_4=V_3$$
Uniform load, moment: $M(x)=M_i+\int V(x)\,dx$ $$M_{1}=0$$ $$M_{12}(x)=M_1+V_1x$$ $$M_2=M_1+V_1L_{12}$$ $$M_{23}(x)=M_2+V_2x-\frac 12Q_1x^2$$ $$M_{3}=M_2+V_2L_{23}-\frac 12Q_1L_{23}^2$$ $$M_{34}(x)=M_3+V_3x$$ $$M_{4}=M_3+V_3L_{34}$$ $M_4$ should equal 0.
Uniform load, slope: $\theta(x)=\theta_i+\int \frac{M(x)}{EI}\,dx$
$$\theta_{1}=unknown$$ $$\theta_{12}(x)=\theta_1+\frac{1}{EI}(M_1x+\frac 12V_1x^2)$$ $$\theta_{2}=\theta_1+\frac{1}{EI}(M_1L_{12}+\frac 12V_1L_{12}^2)=\theta_1+c_{s2}$$ $$\theta_{23}(x)=\theta_2+\frac{1}{EI}(M_2x+\frac 12V_2x^2-\frac 16Q_1x^3)$$ $$\theta_{3}=\theta_2+\frac{1}{EI}(M_2L_{23}+\frac 12V_2L_{23}^2-\frac 16Q_1L_{23}^3)=\theta_2+c_{s3}$$ $$\theta_{34}(x)=\theta_3+\frac{1}{EI}(M_3x+\frac 12V_3x^2)$$ $$\theta_{4}=\theta_3+\frac{1}{EI}(M_3L_{34}+\frac 12V_3L_{34}^2)$$
Uniform load, displacement: $\delta (x)=\delta_i+\int \theta(x)\,dx$ $$\delta_1=0$$ $$\delta_{12}(x)=\delta_1+\theta_1x+\frac{1}{EI}(\frac 12M_1x^2+\frac 16V_1x^3)$$ $$\delta_2=\delta_1+\theta_1L_{12}+\frac{1}{EI}(\frac 12M_1L_{12}^2+\frac 16V_1L_{12}^3)=\delta_1+\theta_1L_{12}+c_{d2}$$ $$\delta_{23}(x)=\delta_2+\theta_2x+\frac{1}{EI}(\frac 12M_2x^2+\frac 16V_2x^3-\frac{1}{24}Q_1x^4)$$ $$\delta_3=\delta_2+\theta_2L_{23}+\frac{1}{EI}(\frac 12M_2L_{23}^2+\frac 16V_2L_{23}^3-\frac{1}{24}Q_1L_{23}^4)=\delta_2+\theta_2L_{23}+c_{d3}$$ $$\delta_{34}(x)=\delta_3+\theta_3x+\frac{1}{EI}(\frac 12M_3x^2+\frac 16V_3x^3)$$ $$\delta_4=\delta_3+\theta_3L_{34}+\frac{1}{EI}(\frac 12M_3L_{34}^2+\frac 16V_3L_{34}^3)=\delta_3+\theta_3L_{34}+c_{d4}$$
The last equation gives $\delta_4=0=\theta_1$+known values$\delta_4=0=\theta_1+known\; values$ after you make all of the substitutions, so this gives the result for $\theta_1$ as follows (where $c_s$ and $c_d$ are simplification of constant terms defined above):
$$\theta_1=-\frac{\delta_1+c_{d2}+c_{d3}+c_{d4}+c_{s2}L_{23}+(c_{s2}+c_{s3})L_{34}}{L_{14}}$$
All values are now knowknown for the beam with a uniform load.
Similar equations are written for the triangular load which ends with $\delta_8=0=\theta_5$+known values.
triangular load, shear: $V(x)=V_i+\int load(x)\,dx$ where load(x)=$Q_2\frac xL$ between points 6 and 7. $$V_{5}=R_5$$ $$V_{56}(x)=V_5$$ $$V_{6}=V_5$$ $$V_{67}(x)=V_6-\frac 12Q_2\frac{x^2}{L_{67}}$$ $$V_7=V_6-\frac 12Q_2L_{67}$$ $$V_{78}(x)=V_7$$ $$V_8=V_7$$
triangular load, moment: $M(x)=M_i+\int V(x)\,dx$ $$M_{5}=0$$ $$M_{56}(x)=M_5+V_5x$$ $$M_6=M_5+V_5L_{56}$$ $$M_{67}(x)=M_6+V_6x-\frac 16Q_2\frac{x^3}{L_{67}}$$ $$M_{7}=M_6+V_6L_{67}-\frac 16Q_2L_{67}^2$$ $$M_{78}(x)=M_7+V_7x$$ $$M_{8}=M_7+V_7L_{78}$$ $M_8$ should equal 0.
triangular load, slope: $\theta(x)=\theta_i+\int \frac{M(x)}{EI}\,dx$
$$\theta_{5}=unknown$$ $$\theta_{56}(x)=\theta_5+\frac{1}{EI}(M_5x+\frac 12V_5x^2)$$ $$\theta_{6}=\theta_5+\frac{1}{EI}(M_5L_{56}+\frac 12V_5L_{56}^2)=\theta_5+c_{s6}$$ $$\theta_{67}(x)=\theta_6+\frac{1}{EI}(M_6x+\frac 12V_6x^2-\frac{1}{24}Q_2\frac{x^4}{L_{67}})$$ $$\theta_{7}=\theta_6+\frac{1}{EI}(M_6L_{67}+\frac 12V_6L_{67}^2-\frac{1}{24}Q_2L_{67}^3)=\theta_6+c_{s7}$$ $$\theta_{78}(x)=\theta_7+\frac{1}{EI}(M_7x+\frac 12V_7x^2)$$ $$\theta_{8}=\theta_7+\frac{1}{EI}(M_7L_{78}+\frac 12V_7L_{78}^2)$$
triangular load, displacement: $\delta (x)=\delta_i+\int \theta(x)\,dx$ $$\delta_5=0$$ $$\delta_{56}(x)=\delta_5+\theta_5x+\frac{1}{EI}(\frac 12M_5x^2+\frac 16V_5x^3)$$ $$\delta_6=\delta_5+\theta_5L_{56}+\frac{1}{EI}(\frac 12M_5L_{56}^2+\frac 16V_5L_{56}^3)=\delta_5+\theta_5L_{56}+c_{d6}$$ $$\delta_{67}(x)=\delta_6+\theta_6x+\frac{1}{EI}(\frac 12M_6x^2+\frac 16V_6x^3-\frac{1}{120}Q_2\frac{x^5}{L_{67}})$$ $$\delta_7=\delta_6+\theta_6L_{67}+\frac{1}{EI}(\frac 12M_6L_{67}^2+\frac 16V_6L_{67}^3-\frac{1}{120}Q_2L_{67}^4)=\delta_6+\theta_6L_{67}+c_{d7}$$ $$\delta_{78}(x)=\delta_7+\theta_7x+\frac{1}{EI}(\frac 12M_7x^2+\frac 16V_7x^3)$$ $$\delta_8=\delta_7+\theta_7L_{78}+\frac{1}{EI}(\frac 12M_7L_{78}^2+\frac 16V_7L_{78}^3)=\delta_7+\theta_7L_{78}+c_{d8}$$
The last equation gives $\delta_8=0=\theta_5+known\; values$ after you make all of the substitutions, so this gives the result for $\theta_5$ as follows (where $c_s$ and $c_d$ are terms defined above):
$$\theta_5=-\frac{\delta_5+c_{d6}+c_{d7}+c_{d8}+c_{s6}L_{67}+(c_{s6}+c_{s7})L_{78}}{L_{58}}$$
All values are now known for the beam with a triangular load.
The results for the trapezoidal resultload are thus $$R_A=R_1+R_5$$ $$R_D=R_4+R_8$$ $$V_A=V_1+V5$$ $$V_B=V_2+V6$$ $$\ldots$$ $$\delta_C=\delta_3+\delta_7$$ $$\delta_D=\delta_4+\delta_8$$
An example which I verified with FEA is the sumas follows: $$L_{AB}=20$$ $$L_{BC}=30$$ $$L_{CD}=40$$ $$Q_1=10$$ $$Q_2=15$$ $$E=1E6$$ $$I=0.75$$ gives results of the uniform load result and $$\theta_1=-0.1976\;radians$$ $$\delta_3=-5.2370$$ $$\theta_5=-0.1513\;radians$$ $$\delta_7=-4.2267$$ The point of maximum displacement occurs at $\theta_{23}(x)+\theta_{67}(x)=0$ which is a solution to a 5th order equation which I did not attempt to solve. An approximate solution is at x=23.283 from B to C which gives a maximum displacement of (-5.412)+(-4.333)=(-9.745).
You should get the triangular resultsame results if I did not make any typos.