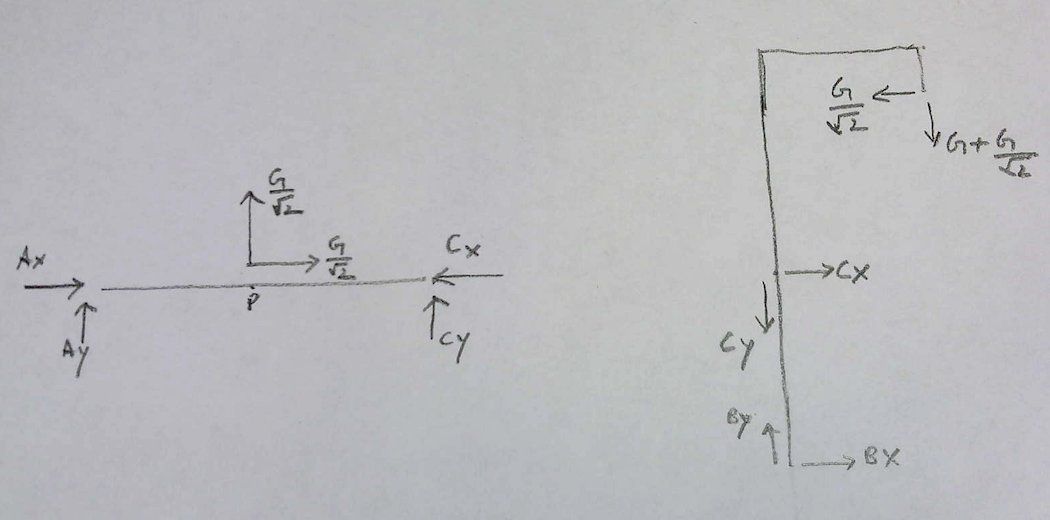
The balance equations would be the following
$$\Sigma F_x = A_x-C_x+\frac{G}{\sqrt{2}}=0$$ $$\Sigma F_y = A_y+C_y+\frac{G}{\sqrt{2}}=0$$ $$\Sigma M_P = A_y a-C_y a=0$$ $$\Sigma F_x = B_x+C_x-\frac{G}{\sqrt{2}}=0$$ $$\Sigma F_y = B_y-C_y-\left(\frac{G}{\sqrt{2}}+G\right)=0$$ $$\Sigma M_B = C_x (3 a)-\frac{G}{\sqrt{2}}(7 a)+ \left(\frac{G}{\sqrt{2}}+G\right)(3 a)=0$$
And the solution turns out as $$ A_x=\frac{1}{6} \left(\sqrt{2} G-6 G\right) \ \ A_y=-\frac{G}{2 \sqrt{2}}$$ $$B_x=\frac{1}{6} \left(6 G-\sqrt{2} G\right)\ \ B_y=\frac{1}{4} \left(\sqrt{2} G+4 G\right)$$ $$C_x=\frac{1}{3} \left(2 \sqrt{2} G-3 G\right) \ \ C_y=-\frac{G}{2 \sqrt{2}}$$
The free-body diagrams